There is some mistake in this Video. Please check images above.
Ex 3.3, 2 - Chapter 3 Class 11 Trigonometric Functions
Last updated at Dec. 13, 2024 by Teachoo
Finding Value of trignometric functions, given angle
Negative Angle Identities
You are here
You are here
Chapter 3 Class 11 Trigonometric Functions
Concept wise
- Radian measure - Conversion
- Arc length
- Finding Value of trignometric functions, given other functions
-
Finding Value of trignometric functions, given angle
- (x + y) formula
- 2x 3x formula - Proving
- 2x 3x formula - Finding value
- cos x + cos y formula
- 2 sin x sin y formula
- Finding Principal solutions
- Finding General Solutions
- Sine and Cosine Formula
Transcript
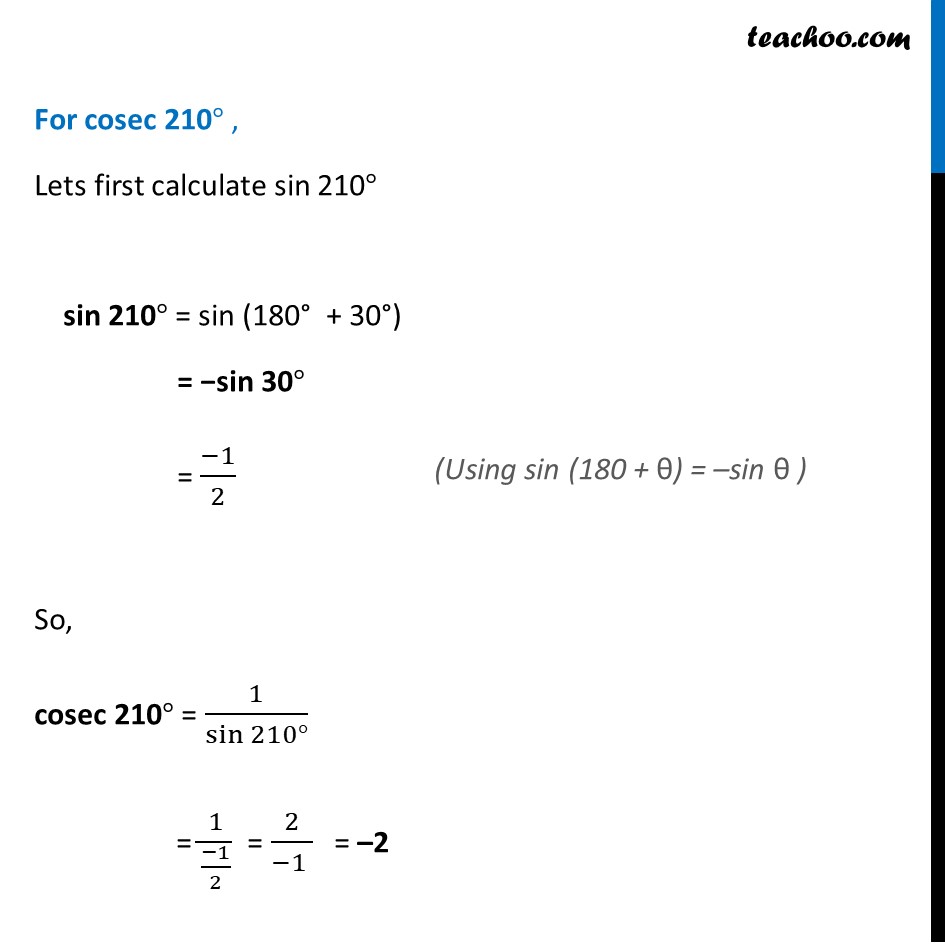
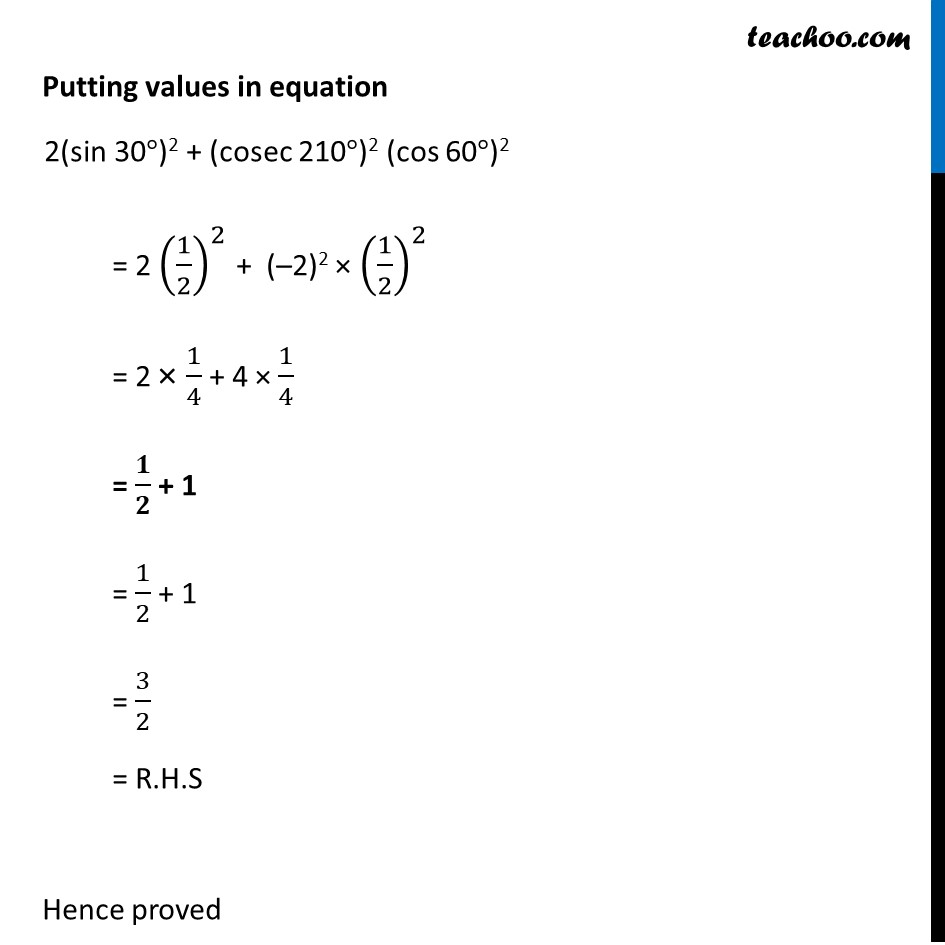
Ex 3.3, 2 Prove that 2sin2 π/6 + cosec2 7π/6 cos2 π/3 = 3/2 Solving L.H.S 2sin2 π/6 + cosec2 7π/6 cos2 π/3 Putting π = 180° = 2 sin2 180/6 + cosec2 (7 ×180)/6 cos2 180/3 = 2sin2 30° + cosec2 210° cos2 60° = 2(sin 30°)2 + (cosec 210°)2 (cos 60°)2 For cosec 210° , Lets first calculate sin 210° sin 210° = sin (180° + 30°) = −sin 30° = (−1)/2 So, cosec 210° = 1/sin〖210°〗 = 1/((−1)/2) = 2/(−1 ) = –2 Putting values in equation 2(sin 30°)2 + (cosec 210°)2 (cos 60°)2 = 2 (1/2)^2 + (–2)2 × (1/2)^2 = 2 × 1/4 + 4 × 1/4 = 𝟏/𝟐 + 1 = 1/2 + 1 = 3/2 = R.H.S Hence proved
