Ex 3.3, 1 - Chapter 3 Class 11 Trigonometric Functions
Last updated at Dec. 13, 2024 by Teachoo
Finding Value of trignometric functions, given angle
Negative Angle Identities
You are here
You are here
Chapter 3 Class 11 Trigonometric Functions
Concept wise
- Radian measure - Conversion
- Arc length
- Finding Value of trignometric functions, given other functions
-
Finding Value of trignometric functions, given angle
- (x + y) formula
- 2x 3x formula - Proving
- 2x 3x formula - Finding value
- cos x + cos y formula
- 2 sin x sin y formula
- Finding Principal solutions
- Finding General Solutions
- Sine and Cosine Formula
Transcript
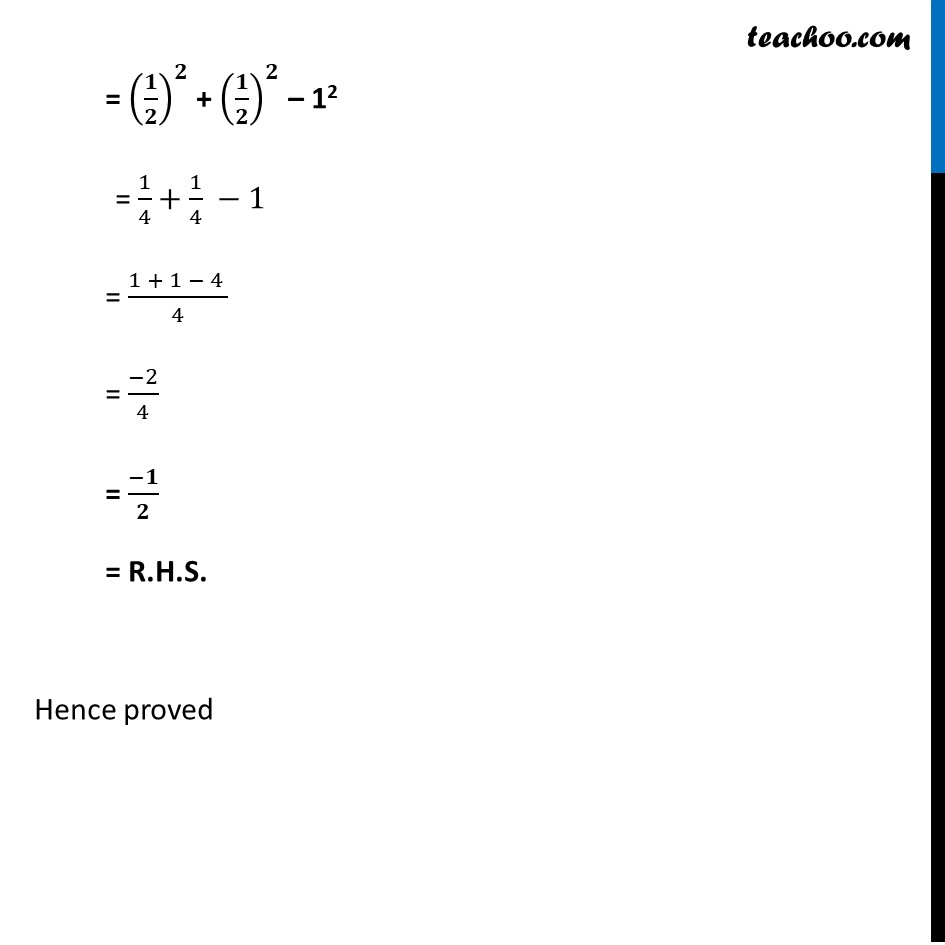
Ex 3.3, 1 Prove that sin2 π/6 + cos2 π/3 – tan2 π/4 = – 1/2 Solving L.H.S sin2 π/6 + cos2 π/3 − tan2 π/4 Putting π = 180° = sin2 (180° )/6 + cos2 (180° )/3 – tan2 (180° )/6 = sin2 30° + cos2 60° – tan2 45° Putting sin 30° = 1/2 , sin 60° = 1/2 , tan 45° = 1 = (𝟏/𝟐)^𝟐 + (𝟏/𝟐)^𝟐 – 12 = 1/4+ 1/4 −1 = (1 + 1 − 4 )/4 = (−2)/4 = (−𝟏)/𝟐 = R.H.S. Hence proved
