Question 16 - CBSE Class 10 Sample Paper for 2024 Boards - Maths Standard - Solutions of Sample Papers for Class 10 Boards

Last updated at Dec. 13, 2024 by Teachoo

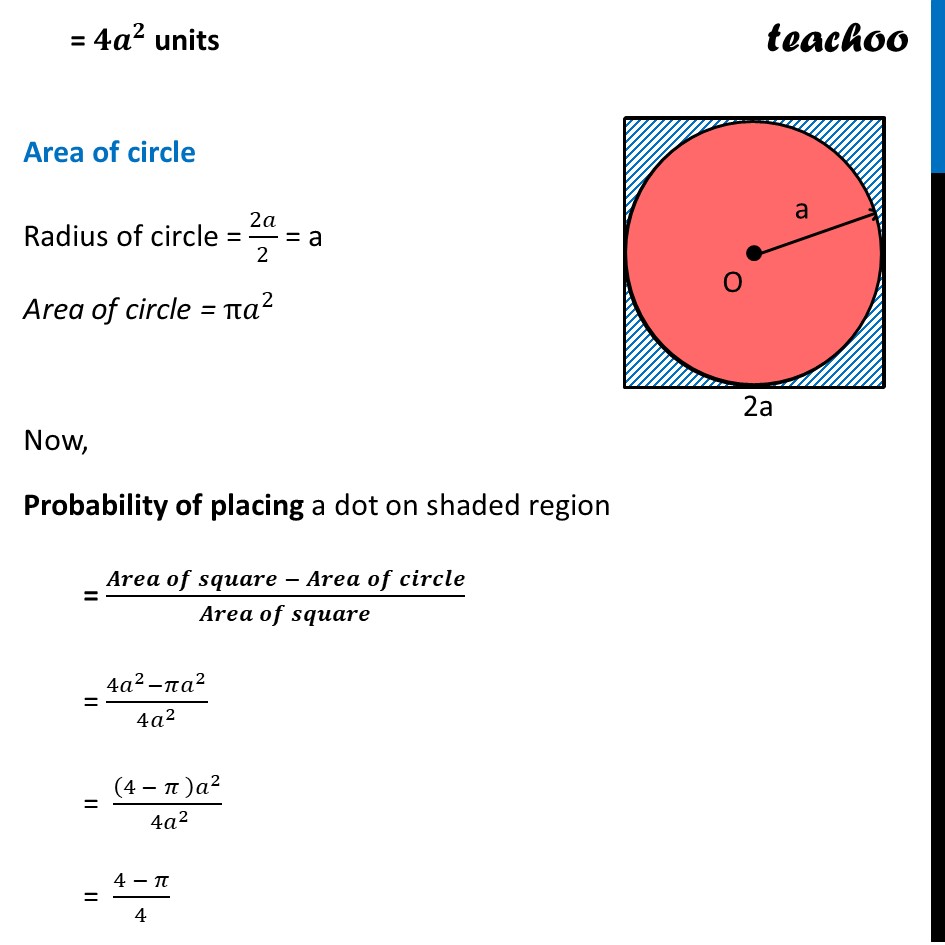
There is a square board of side '2a' units circumscribing a red circle. Jayadev asked to keep a dot on the above said board. The probability that he keeps the dot on the shaded region is.

(a) π/4 (b) (4-π)/4 (c) (π - 4)/4 (d) 4/π

Sample paper Maths Class 10 - 2024 Boards - Standard - Until ques 25.jpg QP Part 1 Standard - Class 10 Maths 2.jpg

Next: Question 17 Important → You saved atleast 2 minutes of distracting ads by going ad-free. Thank you :)

You saved atleast 2 minutes by viewing the ad-free version of this page. Thank you for being a part of Teachoo Black.

Transcript

Probability of placing a dot on shaded region = (𝐴𝑟𝑒𝑎 𝑜𝑓 𝑠ℎ𝑎𝑑𝑒𝑑 𝑟𝑒𝑔𝑖𝑜𝑛 (𝑏𝑙𝑢𝑒))/(𝑇𝑜𝑡𝑎𝑙 𝑎𝑟𝑒𝑎) = (𝑨𝒓𝒆𝒂 𝒐𝒇 𝒔𝒒𝒖𝒂𝒓𝒆 − 𝑨𝒓𝒆𝒂 𝒐𝒇 𝒄𝒊𝒓𝒄𝒍𝒆)/(𝑨𝒓𝒆𝒂 𝒐𝒇 𝒄𝒊𝒓𝒄𝒍𝒆) Area of square Side = 2a Area = 〖(2𝑎)〗^2 =〖 𝟒𝒂〗^𝟐 units Area of circle Radius of circle = 2𝑎/2 = a Area of circle = π𝑎^2 Now, Probability of placing a dot on shaded region = (𝑨𝒓𝒆𝒂 𝒐𝒇 𝒔𝒒𝒖𝒂𝒓𝒆 − 𝑨𝒓𝒆𝒂 𝒐𝒇 𝒄𝒊𝒓𝒄𝒍𝒆)/(𝑨𝒓𝒆𝒂 𝒐𝒇 𝒄𝒊𝒓𝒄𝒍𝒆) = (4𝑎^2−𝜋𝑎^2)/(4𝑎^2 ) = ((4 − 𝜋 ) 𝑎^2)/〖 4𝑎〗^2 = (4 − 𝜋)/4So, the correct answer is (b)

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular