Ex 2.3, 4 - Chapter 2 Class 11 Relations and Functions

Last updated at April 16, 2024 by

Ex 2.3, 4 - Class 11 - Relations and functions - t(c) = 9/5C + 32

Ex 2.3, 4 - Chapter 2 Class 11 Relations and Functions - Part 2
Ex 2.3, 4 - Chapter 2 Class 11 Relations and Functions - Part 3
Ex 2.3, 4 - Chapter 2 Class 11 Relations and Functions - Part 4
Ex 2.3, 4 - Chapter 2 Class 11 Relations and Functions - Part 5

Go Ad-free

Transcript

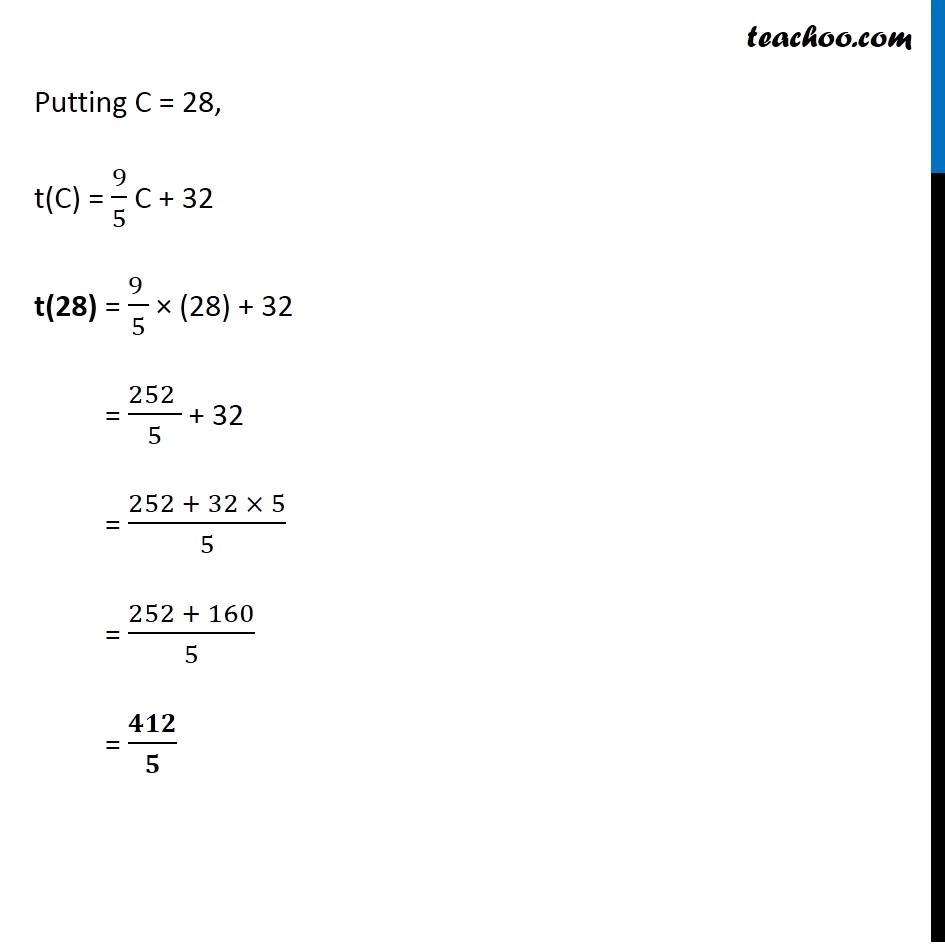
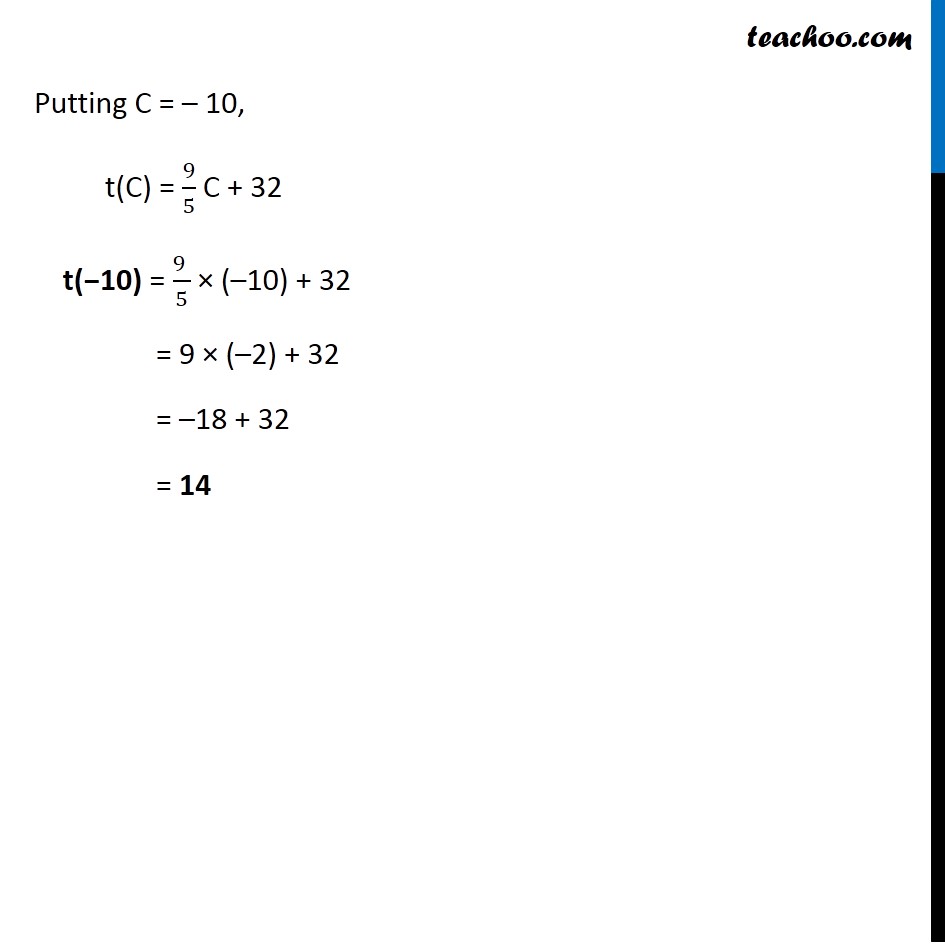
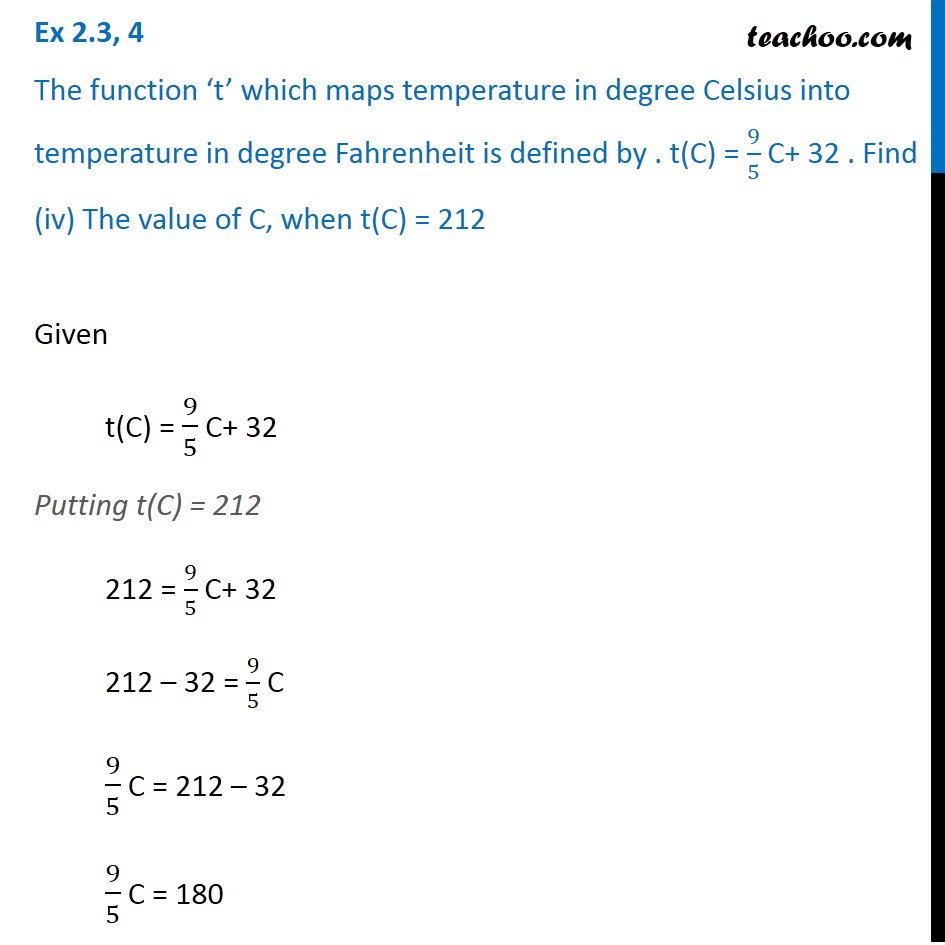
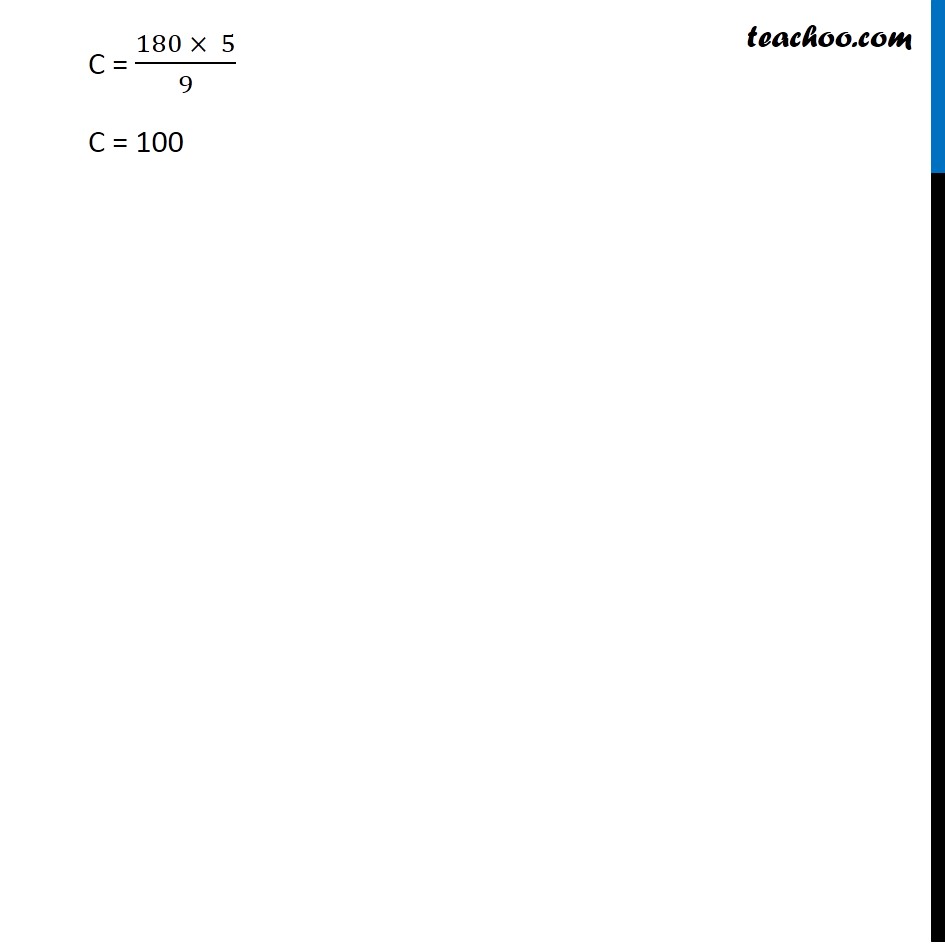
Ex 2.3, 4 The function ‘t’ which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by . t(C) = 9/5 C+ 32 . Find (i) t (0) (ii) t (28) (iii) t (-10) Given t(C) = 9/5 C + 32 Putting C = 0, t(0) = (9 )/5 × (0) + 32 = 0 + 32 = 32 Putting C = 28, t(C) = 9/5 C + 32 t(28) = (9 )/5 × (28) + 32 = (252 )/5 + 32 = (252 + 32 × 5)/5 = (252 + 160)/5 = 𝟒𝟏𝟐/𝟓 Putting C = – 10, t(C) = 9/5 C + 32 t(−10) = (9 )/5 × (–10) + 32 = 9 × (–2) + 32 = –18 + 32 = 14 Ex 2.3, 4 The function ‘t’ which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by . t(C) = 9/5 C+ 32 . Find (iv) The value of C, when t(C) = 212 Given t(C) = 9/5 C+ 32 Putting t(C) = 212 212 = 9/5 C+ 32 212 – 32 = 9/5 C 9/5 C = 212 – 32 9/5 C = 180 C = (180 × 5)/9 C = 100

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo