Learn about Sets on our Youtube Channel - https://www.youtube.com/watch?v=X6znC33Dy1c
Example 31 - Chapter 1 Class 11 Sets
Last updated at Jan. 27, 2020 by Teachoo
Transcript
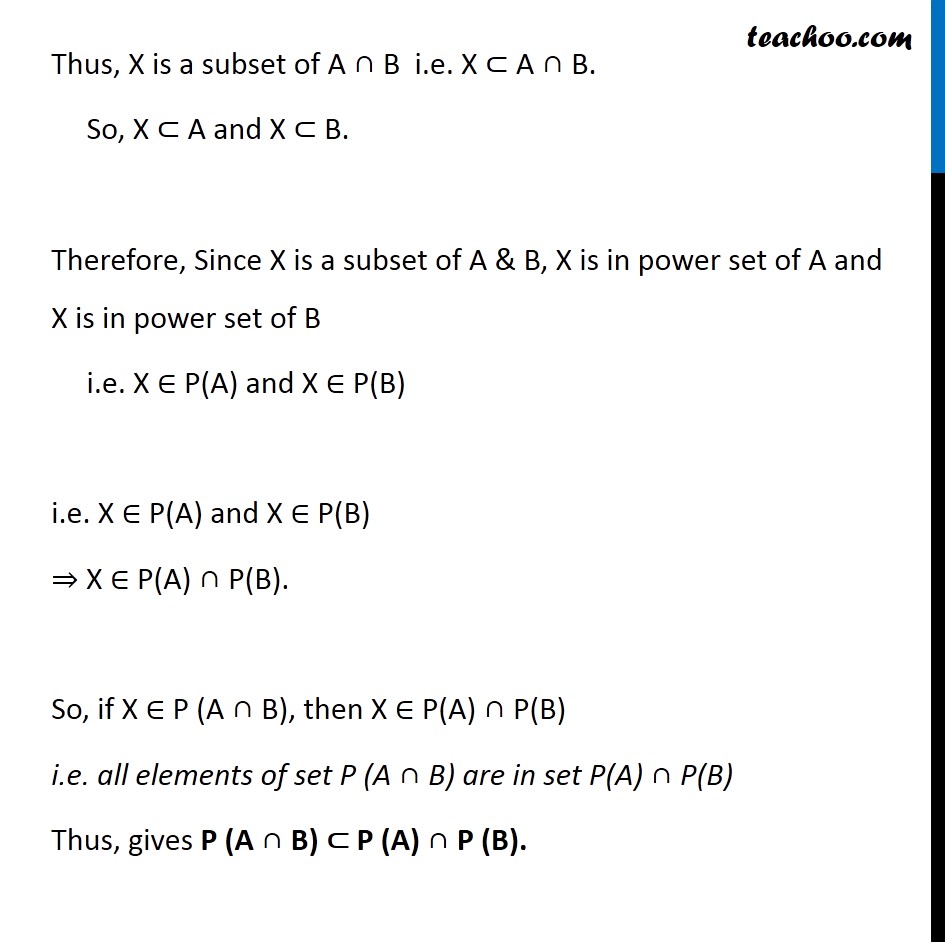
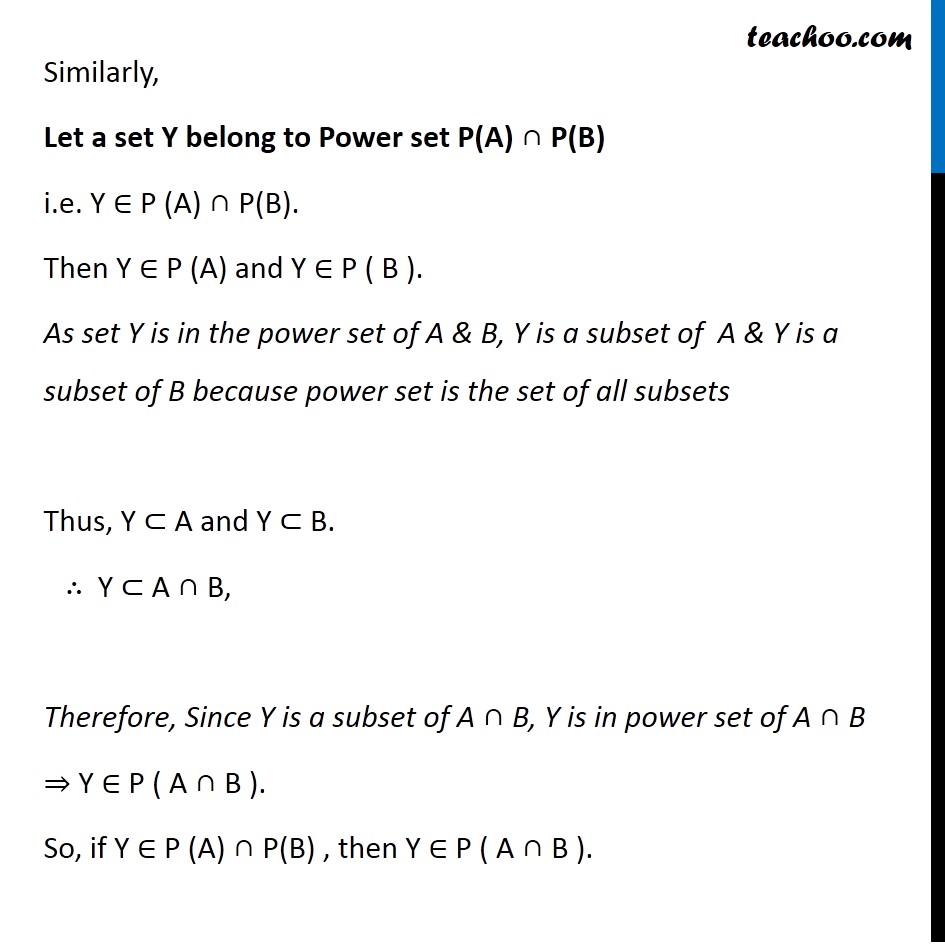
Example 31 For any sets A and B, show that P(A ∩ B) = P(A) ∩ P(B). To prove two sets equal, we need to prove that they are subset of each other i.e.. we have to prove P (A ∩ B) ⊂ P (A) ∩ P (B) & P(A) ∩ P(B) ⊂ P ( A ∩ B) Let a set X belong to Power set P(A ∩ B) i.e. X ∈ P ( A ∩ B ). As set X is in the power set of A ∩ B, X is a subset of A ∩ B because power set is the set of all subsets ⊂ Subset A ⊂ B (all elements of set A in set B) Thus, X is a subset of A ∩ B i.e. X ⊂ A ∩ B. So, X ⊂ A and X ⊂ B. Therefore, Since X is a subset of A & B, X is in power set of A and X is in power set of B i.e. X ∈ P(A) and X ∈ P(B) i.e. X ∈ P(A) and X ∈ P(B) ⇒ X ∈ P(A) ∩ P(B). So, if X ∈ P (A ∩ B), then X ∈ P(A) ∩ P(B) i.e. all elements of set P (A ∩ B) are in set P(A) ∩ P(B) Thus, gives P (A ∩ B) ⊂ P (A) ∩ P (B). Similarly, Let a set Y belong to Power set P(A) ∩ P(B) i.e. Y ∈ P (A) ∩ P(B). Then Y ∈ P (A) and Y ∈ P ( B ). As set Y is in the power set of A & B, Y is a subset of A & Y is a subset of B because power set is the set of all subsets Thus, Y ⊂ A and Y ⊂ B. ∴ Y ⊂ A ∩ B, Therefore, Since Y is a subset of A ∩ B, Y is in power set of A ∩ B ⇒ Y ∈ P ( A ∩ B ). So, if Y ∈ P (A) ∩ P(B) , then Y ∈ P ( A ∩ B ). This gives P (A) ∩ P (B) ⊂ P (A ∩ B) Now, Since P (A ∩ B) ⊂ P (A) ∩ P (B) & P(A) ∩ P(B) ⊂ P ( A ∩ B) Hence, P ( A ∩ B ) = P ( A ) ∩ P ( B ).
Examples
Example 1
Example 2
Example 3
Example 4
Example 5 Important
Example 6 Important
Example 7
Example 8
Example 9 Important
Example 10
Example 11 Important
Example 12
Example 13 Important
Example 14
Example 15
Example 16
Example 17
Example 18 Important
Example 19
Example 20
Example 21
Example 22 Important
Example 23
Example 24 Important
Example 25 Important
Example 26
Example 27 Important
Example 28
Example 29 Important
Example 30 Important
Example 31 Important You are here
Example 32 Important
Example 33 Important
Example 34 Important
Chapter 1 Class 11 Sets
Serial order wise
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 9 years. He provides courses for Maths and Science at Teachoo.