CBSE Class 12 Sample Paper for 2023 Boards
You are here
CBSE Class 12 Sample Paper for 2023 Boards
Last updated at Dec. 13, 2024 by Teachoo
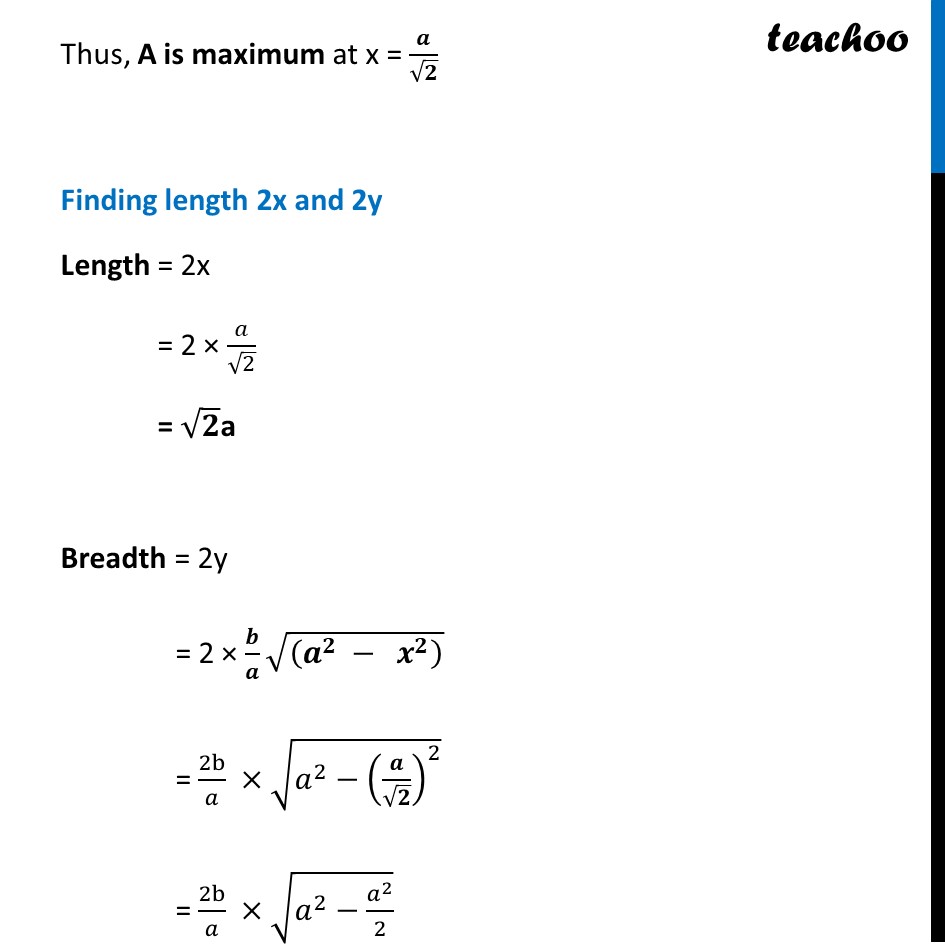
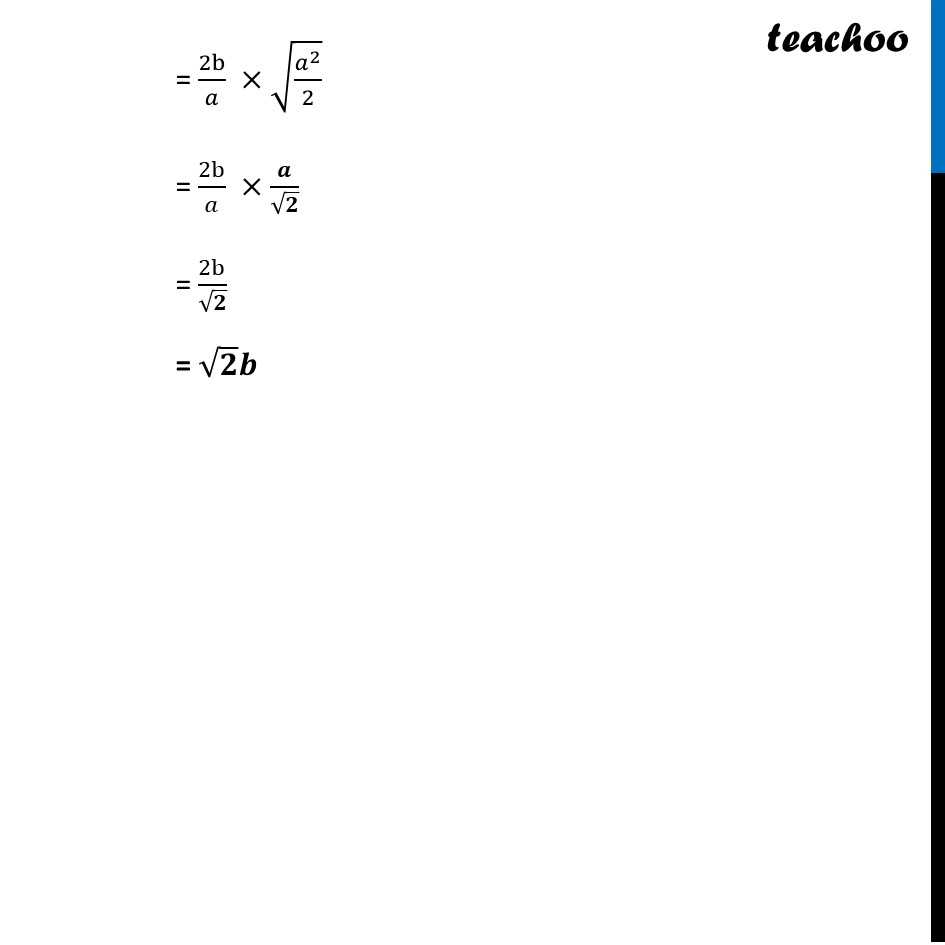
![[Class 12] Use First derivative Test to find length 2x and width 2y - CBSE Class 12 Sample Paper for 2023 Boards](https://cdn.teachoo.com/4a22a488-2dbf-42ba-8a58-ad4f4fee547d/slide52.jpg)
Transcript
Question 37 (iii) (Choice 1) Use First derivative Test to find the length 2x and width 2y of the soccer field (in terms of a and b) that maximize its area.Now, 𝑑𝑍/𝑑𝑥=(32𝑏^2)/𝑎^2 × (𝑎^2 𝑥−2𝑥^3) 𝒅𝒁/𝒅𝒙=(32𝑏^2)/𝑎^2 × 𝑥 × (𝒂^𝟐−𝟐𝒙^𝟐) Since 𝑑𝑍/𝑑𝑥 = changes sign from positive to negative 𝒙 = 𝒂/√𝟐 is the maxima. Thus, A is maximum at x = 𝒂/√𝟐 Finding length 2x and 2y Length = 2x = 2 × 𝑎/√2 = √𝟐a Breadth = 2y = 2 × (" " 𝒃)/𝒂 √((𝒂^𝟐 − 𝒙^𝟐 ) ) = 2b/𝑎 ×√(𝑎^2−(𝒂/√𝟐)^2 ) = 2b/𝑎 ×√(𝑎^2−𝑎^2/2) = 2b/𝑎 ×√(𝑎^2/2) = 2b/𝑎 ×𝒂/√𝟐 = 2b/√𝟐 = √𝟐 𝒃
