CBSE Class 12 Sample Paper for 2023 Boards

Practice Questions CBSE - Maths Class 12 (2023 Boards) →
Class 12
Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Question 37 (ii) - CBSE Class 12 Sample Paper for 2023 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Dec. 13, 2024 by

Find the critical point of the function.

 

Slide49.JPG

Slide50.JPG
Slide51.JPG

Go Ad-free

Transcript

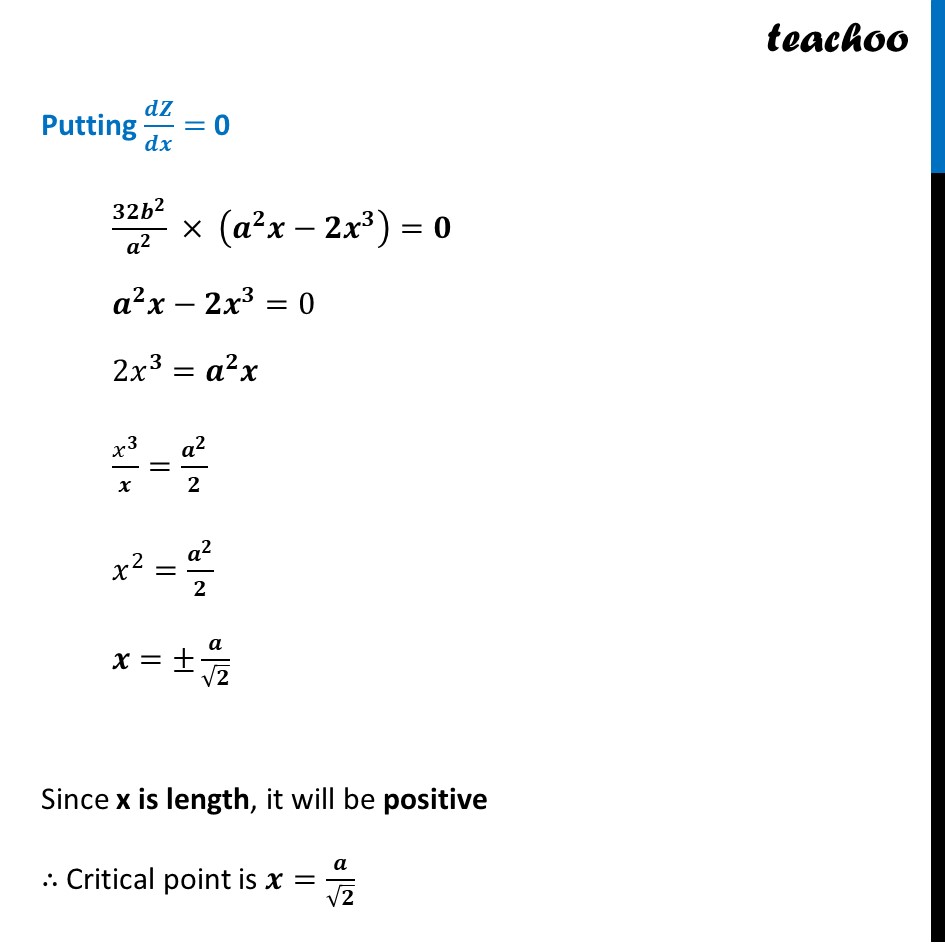
Question 37 (ii) Find the critical point of the function.Now A =(" " 𝟒𝒃)/𝒂 " " 𝒙√((𝒂^𝟐 − 𝒙^𝟐 ) ) We need to maximise A, but A has a square root Which will be difficult to differentiate Let Z = A2 Z = ((" " 𝟒𝒃)/𝒂 𝒙√((𝒂^𝟐 − 𝒙^𝟐 ) ))^2 Z = (16𝑏^2)/𝑎^2 × 𝑥^2 (𝑎^2−𝑥^2 ) Z = (𝟏𝟔𝒃^𝟐)/𝒂^𝟐 × (𝒂^𝟐 𝒙^𝟐−𝒙^𝟒) Since A is positive, A is maximum if A2 is maximum So, we maximise Z = A2 Differentiating w.r.t x 𝑑𝑍/𝑑𝑥= 𝑑((𝟏𝟔𝒃^𝟐)/𝒂^𝟐 (𝑎^2 𝑥^2−𝑥^4))/𝑑𝑥 𝑑𝑍/𝑑𝑥=(16𝑏^2)/𝑎^2 × 𝑑(𝑎^2 𝑥^2−𝑥^4 )/𝑑𝑥 𝑑𝑍/𝑑𝑥=(16𝑏^2)/𝑎^2 × (𝑎^2 × 2𝑥−4𝑥^3) 𝑑𝑍/𝑑𝑥=(16𝑏^2)/𝑎^2 × (〖2𝑎〗^2 𝑥−4𝑥^3) 𝒅𝒁/𝒅𝒙=(𝟑𝟐𝒃^𝟐)/𝒂^𝟐 × (𝒂^𝟐 𝒙−𝟐𝒙^𝟑) Putting 𝒅𝒁/𝒅𝒙= 0 (𝟑𝟐𝒃^𝟐)/𝒂^𝟐 × (𝒂^𝟐 𝒙−𝟐𝒙^𝟑 )=𝟎 𝒂^𝟐 𝒙−𝟐𝒙^𝟑=0 2𝑥^𝟑=𝒂^𝟐 𝒙 𝑥^𝟑/𝒙=𝒂^𝟐/𝟐 𝑥^2=𝒂^𝟐/𝟐 𝒙=±𝒂/√𝟐 Since x is length, it will be positive ∴ Critical point is 𝒙=𝒂/√𝟐

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo