Question 2 - Conversion of one shape to another - Chapter 12 Class 10 Surface Areas and Volumes

Transcript

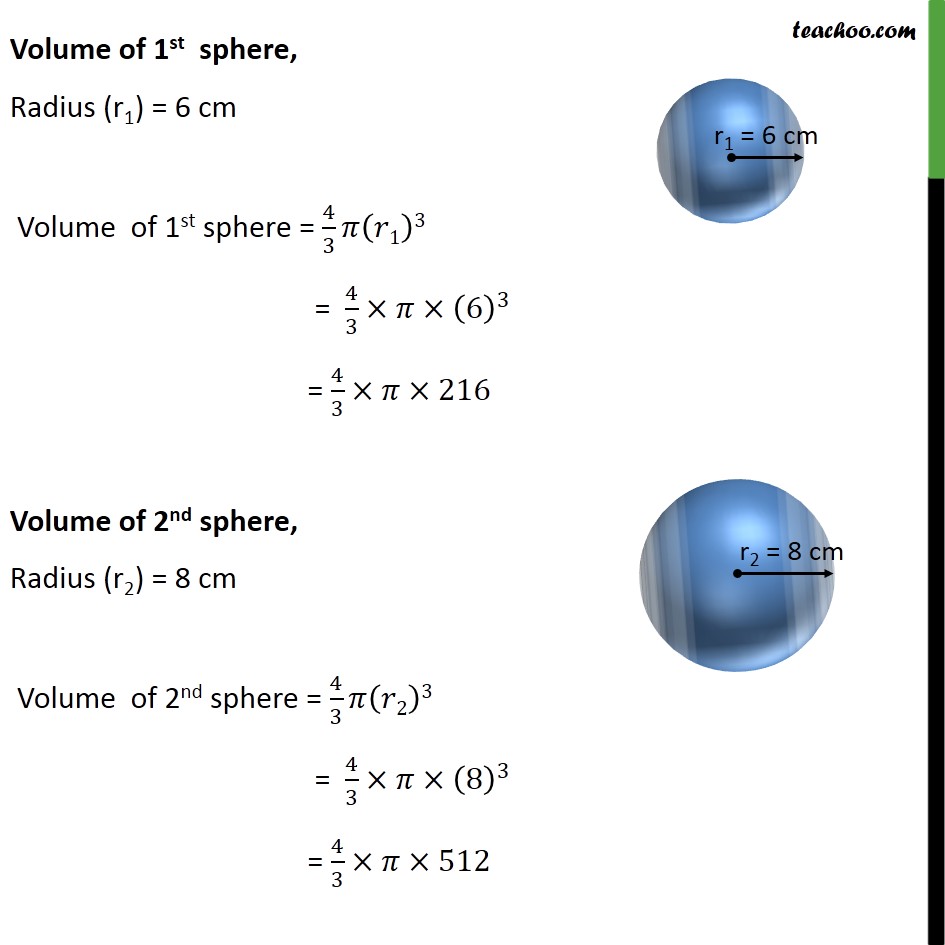
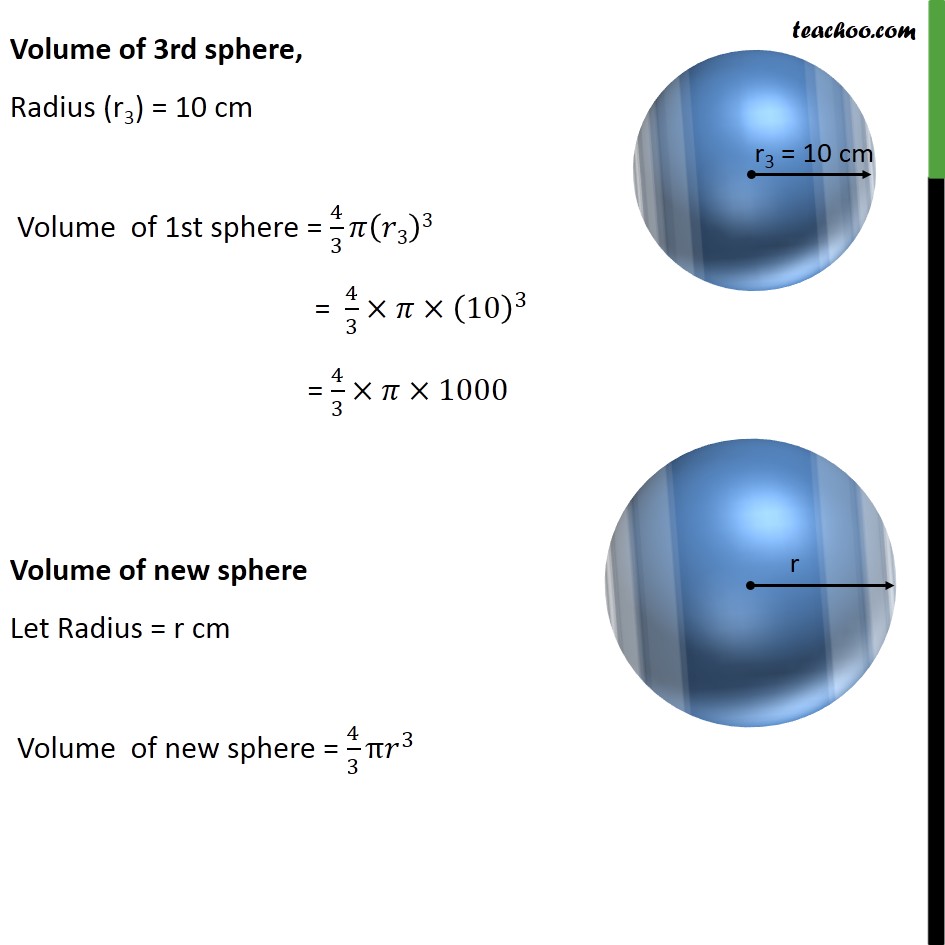
Question 2 Metallic spheres of radii 6 cm, 8 cm and 10 cm, respectively, are melted to form a single solid sphere. Find the radius of the resulting sphere. Since 3 spheres are melted to from one new sphere. Volume of 3 old sphere = volume of new sphere Volume of 1st sphere + volume of 2nd sphere + volume of 3rd sphere = Volume of new sphere Volume of 1st sphere, Radius (r1) = 6 cm Volume of 1st sphere = 4/3 𝜋(𝑟1)3 = 4/3×𝜋×(6)^3 = 4/3×𝜋×216 Volume of 2nd sphere, Radius (r2) = 8 cm Volume of 2nd sphere = 4/3 𝜋(𝑟2)3 = 4/3×𝜋×(8)^3 = 4/3×𝜋×512 Volume of 3rd sphere, Radius (r3) = 10 cm Volume of 1st sphere = 4/3 𝜋(𝑟3)3 = 4/3×𝜋×(10)^3 = 4/3×𝜋×1000 Volume of new sphere Let Radius = r cm Volume of new sphere = 4/3 π𝑟^3 Now , Volume of new sphere = Volume of (1st + 2nd + 3rd ) spheres 4/3 𝜋𝑟3 = 4/3 𝜋×216+4/3 𝜋×512+4/3 𝜋×1000 4/3 𝜋𝑟3 = 4/3 𝜋 (216 + 512 + 1000) 4/3 𝜋𝑟3 = 4/3 𝜋 (1728) r3 = 1728 r = ∛1728 r = ∛(12×12×12) r = ∛((12)3) r = 12 cm Hence, radius of the resulting sphere = 12 cm