Ex 12.2, 3 - Chapter 12 Class 10 Surface Areas and Volumes

Transcript

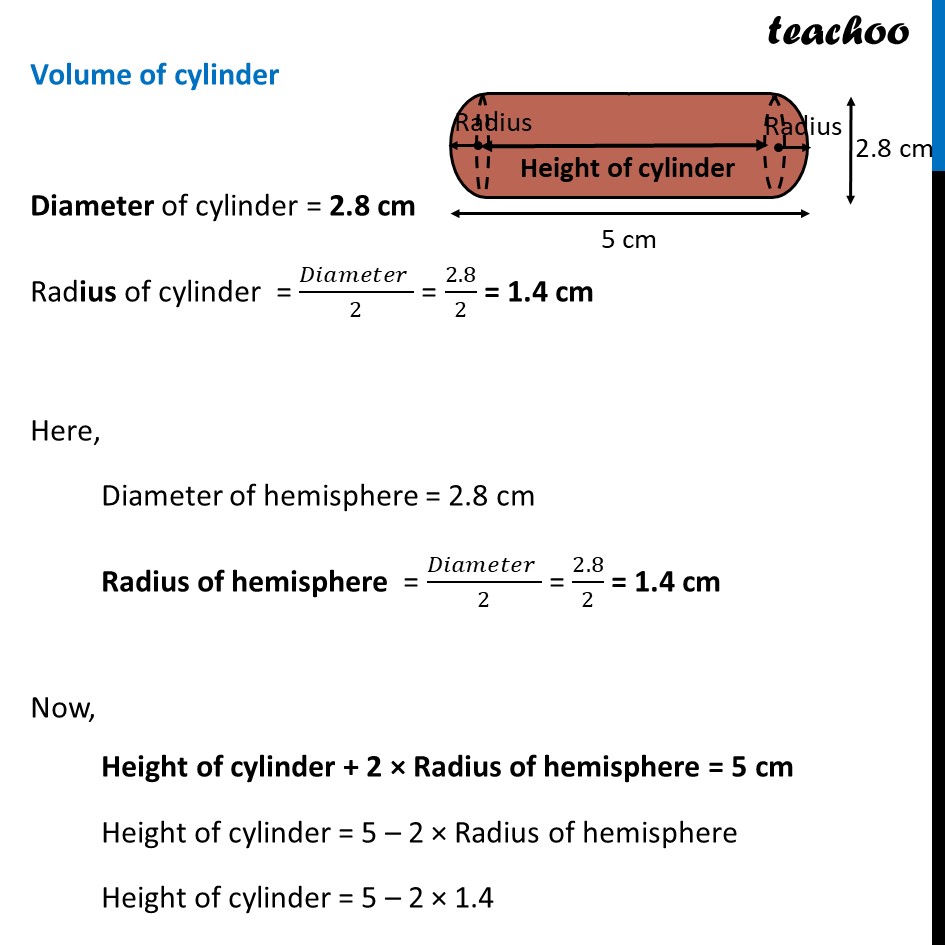
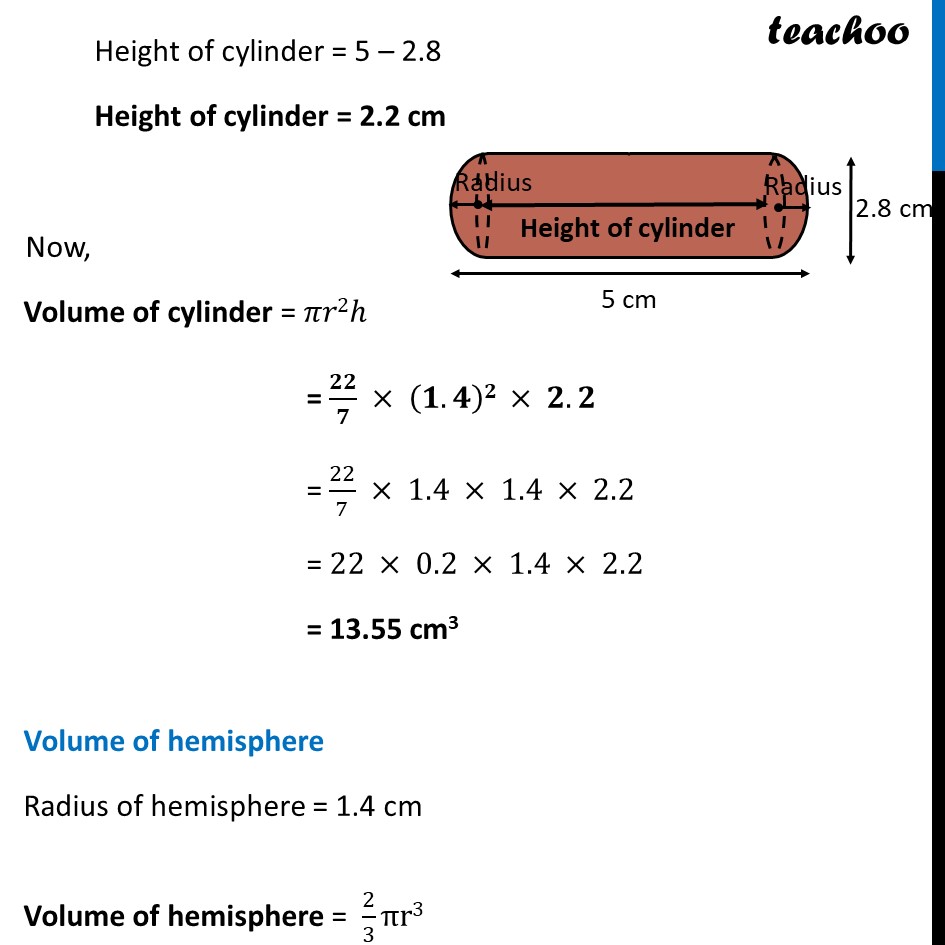
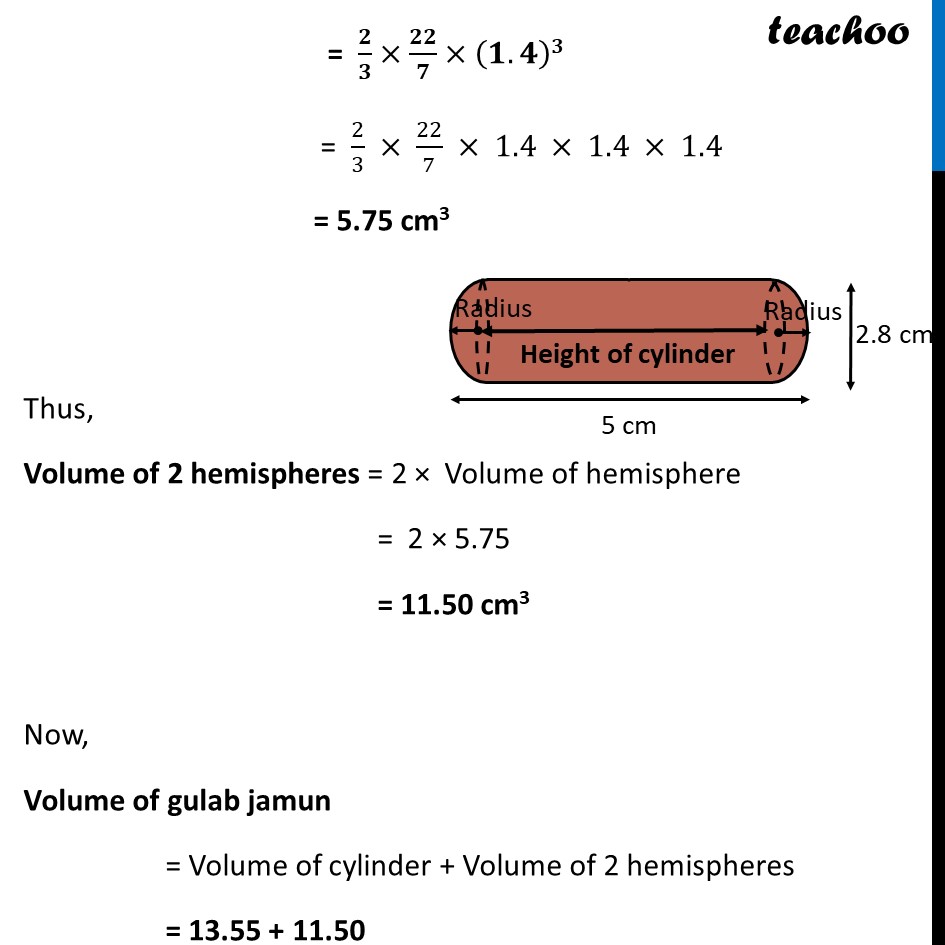
Ex 12.2, 3 Gulab jamun, contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 gulab jamuns, each shaped like a cylinder with two hemispherical ends with length 5 cm and diameter 2.8 cm (see figure) Lets first find Volume of 1 gulab jamun Since gulabjamun contains one cylinder and 2 hemispheres Volume of gulab jamun = Volume of cylinder + Volume of 2 hemispheres Volume of cylinder Diameter of cylinder = 2.8 cm Radius of cylinder = (𝐷𝑖𝑎𝑚𝑒𝑡𝑒𝑟 )/2 = 2.8/2 = 1.4 cm Here, Diameter of hemisphere = 2.8 cm Radius of hemisphere = (𝐷𝑖𝑎𝑚𝑒𝑡𝑒𝑟 )/2 = 2.8/2 = 1.4 cm Now, Height of cylinder + 2 × Radius of hemisphere = 5 cm Height of cylinder = 5 – 2 × Radius of hemisphere Height of cylinder = 5 – 2 × 1.4 Height of cylinder = 5 – 2.8 Height of cylinder = 2.2 cm Now, Volume of cylinder = 𝜋𝑟2ℎ = 𝟐𝟐/𝟕 × (𝟏.𝟒)𝟐 × 𝟐.𝟐 = 22/7 × 1.4 × 1.4 × 2.2 = 22 × 0.2 × 1.4 × 2.2 = 13.55 cm3 Volume of hemisphere Radius of hemisphere = 1.4 cm Volume of hemisphere = 2/3 πr3 = 𝟐/𝟑×𝟐𝟐/𝟕×(𝟏.𝟒)𝟑 = 2/3 × 22/7 × 1.4 × 1.4 × 1.4 = 5.75 cm3 Thus, Volume of 2 hemispheres = 2 × Volume of hemisphere = 2 × 5.75 = 11.50 cm3 Now, Volume of gulab jamun = Volume of cylinder + Volume of 2 hemispheres = 13.55 + 11.50 = 25.05 cm3 So, Volume of 1 gulabjamun = 25.05 cm3 Volume of 45 gulabjamun = 45 × 25.05 cm3 We need to find quantity of syrup in 45 gulabjamun It is given that quantity of syrup in gulabjamun is 30% So, Syrup in 45 gulabjamun = 30% × 45 × 25.05 = 30/100 × 45 × 24.5 = 338.185 cm3 = 338 cm3 (approx)