Example 7 - Chapter 9 Class 10 Some Applications of Trigonometry

Last updated at August 5, 2026 by

Example 7 - From a point on a bridge across a river - Questions easy to difficult

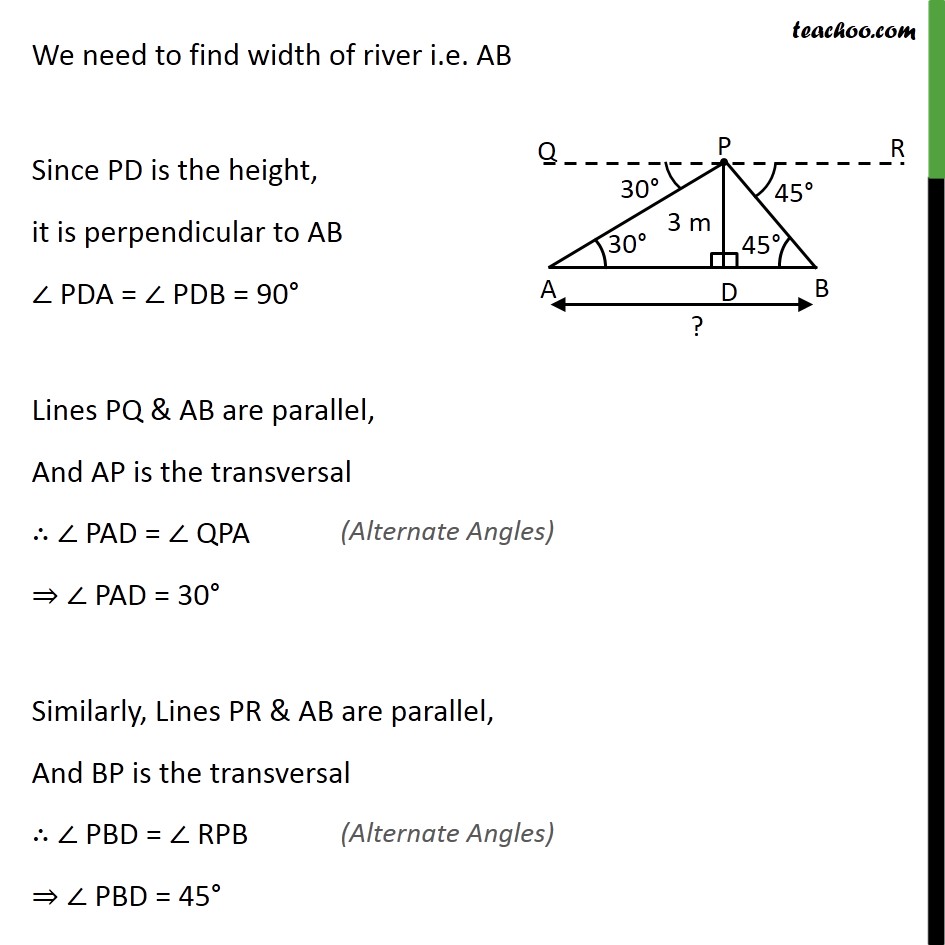
Example 7 - Chapter 9 Class 10 Some Applications of Trigonometry - Part 2
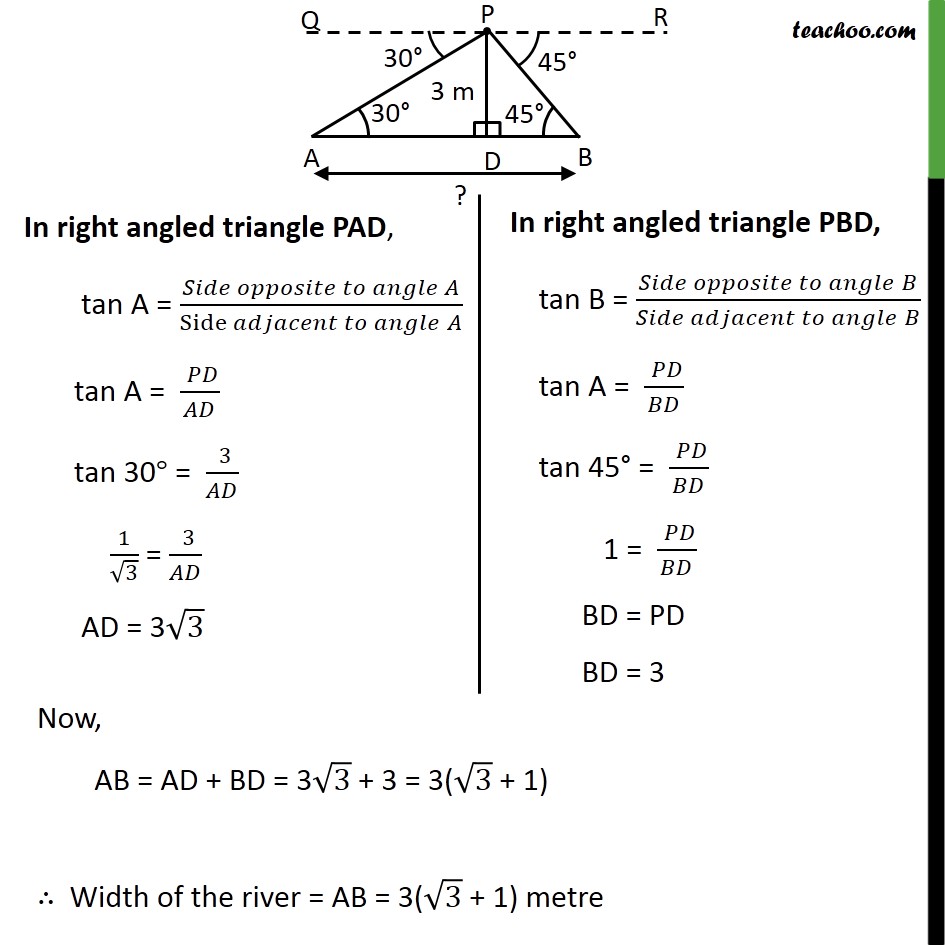
Example 7 - Chapter 9 Class 10 Some Applications of Trigonometry - Part 3

Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 3 questions, selected from your answers, mistakes, and progress.
Remove Ads

Transcript

Example 7 From a point on a bridge across a river, the angles of depression of the banks on opposite sides of the river are 30 and 45 , respectively. If the bridge is at a height of 3 m from the banks, find the width of the river. Let the width of the river = AB And the bridge is at a height of 3m from the banks, So, DP = 3 metre Angle of depression of the banks on opposite sides of the river are 30 and 45 respectively. So, QPA = 30 and RPB = 45 We need to find width of river i.e. AB Since PD is the height, it is perpendicular to AB PDA = PDB = 90 Lines PQ & AB are parallel, And AP is the transversal PAD = QPA PAD = 30 Similarly, Lines PR & AB are parallel, And BP is the transversal PBD = RPB PBD = 45 Now, AB = AD + BD = 3 3 + 3 = 3( 3 + 1) Width of the river = AB = 3( 3 + 1) metre

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.