Example 5 - Chapter 8 Class 10 Introduction to Trignometry (Term 1)
Last updated at Aug. 5, 2021 by Teachoo
Examples
Example 1
Example 2 Important
Example 3
Example 4
Example 5 Important You are here
Example 6
Example 7 Important
Example 8 Important
Example 9 Deleted for CBSE Board 2022 Exams
Example 10 Important Deleted for CBSE Board 2022 Exams
Example 11 Important Deleted for CBSE Board 2022 Exams
Example 12
Example 13 Important
Example 14 Important
Example 15 Important
Chapter 8 Class 10 Introduction to Trignometry (Term 1)
Serial order wise
Transcript
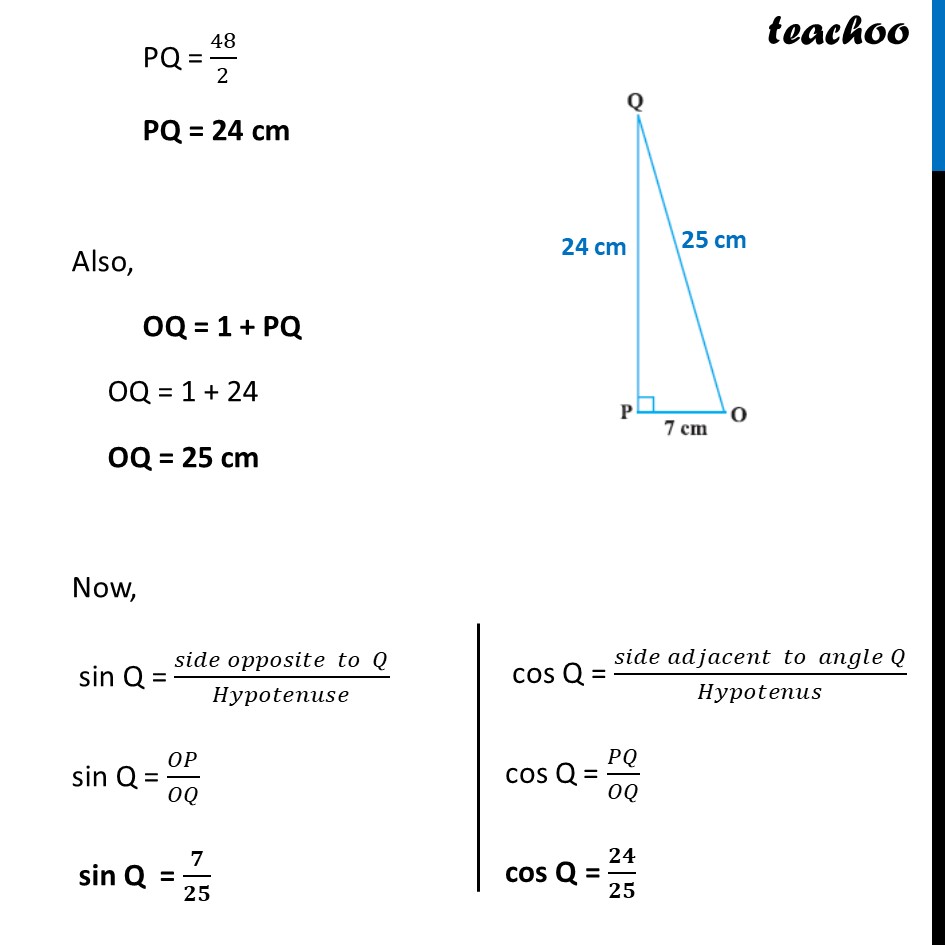
Example 5 In Δ OPQ, right-angled at P, OP = 7 cm and OQ – PQ = 1 cm (see Fig.). Determine the values of sin Q and cos Q. In is given that in triangle OPQ, OQ – PQ = 1 cm OQ = 1 + PQ Using Pythagoras theorem (Hypotenuse)2 = (Height)2 + (Base)2 OQ2 = PQ2 + OP2 (1 + PQ)2 = PQ2 + (7)2 1 + PQ2 + 2PQ = PQ2 + 49 1 + PQ2 + 2PQ – PQ2 – 49 = 0 2PQ – 48 = 0 2PQ = 48 PQ = 48/2 PQ = 24 cm Also, OQ = 1 + PQ OQ = 1 + 24 OQ = 25 cm Now, sin Q = (𝑠𝑖𝑑𝑒 𝑜𝑝𝑝𝑜𝑠𝑖𝑡𝑒 𝑡𝑜 𝑄)/𝐻𝑦𝑝𝑜𝑡𝑒𝑛𝑢𝑠𝑒 sin Q = 𝑂𝑃/𝑂𝑄 sin Q = 7/25
