
Trignometric ratios of Specific Angles - Evaluating
Trignometric ratios of Specific Angles - Evaluating
Last updated at Dec. 13, 2024 by Teachoo


Transcript
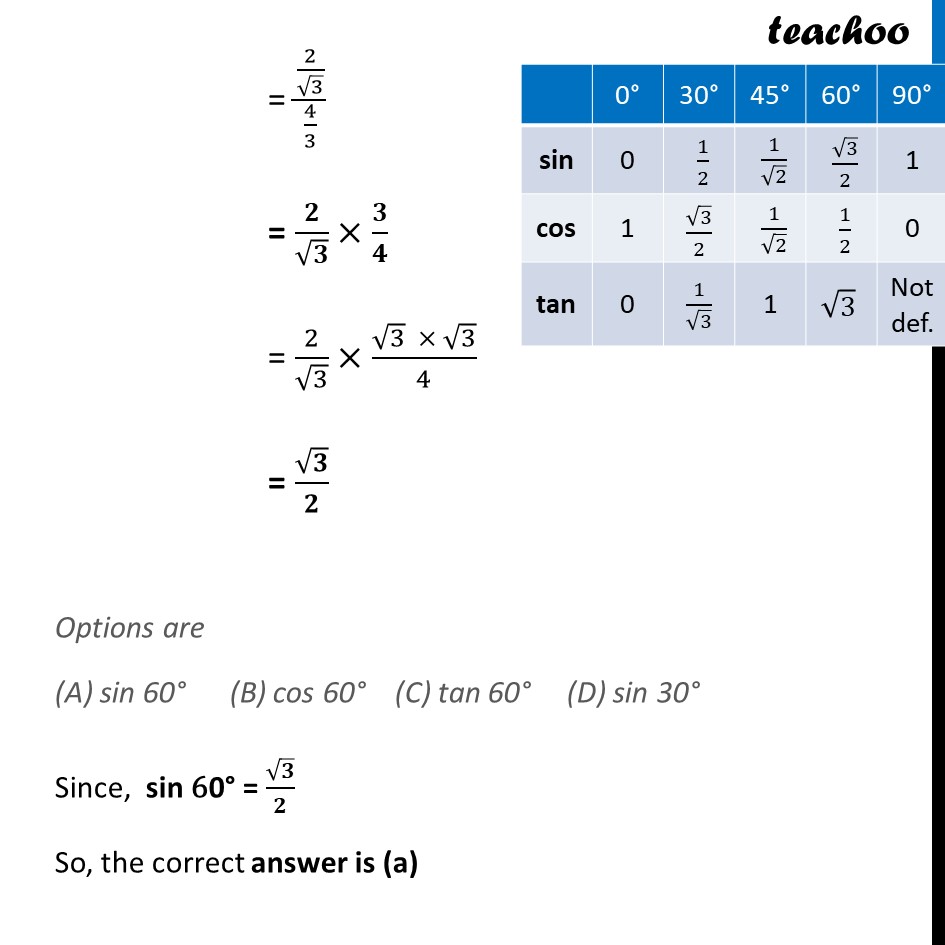
Ex 8.2, 2 Choose the correct option and justify your choice : (i) (2 tan〖30°〗)/(1 + 𝑡𝑎𝑛2 30°) = sin 60° (B) cos 60° (C) tan 60° (D) sin 30° We know that , tan "30°" = 𝟏/√𝟑 So, (𝟐 𝒕𝒂𝒏〖𝟑𝟎°〗)/(𝟏 + 𝒕𝒂𝒏𝟐 𝟑𝟎°) = (𝟐 × (𝟏/√𝟑))/(𝟏 + (𝟏/√𝟑)^𝟐 ) = (2/√3)/(1 + 1/3) = (2/√3)/(4/3) = 𝟐/√𝟑×𝟑/𝟒 = 2/√3×(√3 × √3)/4 = √𝟑/𝟐 Options are (A) sin 60° (B) cos 60° (C) tan 60° (D) sin 30° Since, sin "60°" = √𝟑/𝟐 So, the correct answer is (a)