Check sibling questions

Example 1 - Chapter 6 Class 10 Triangles

Last updated at May 29, 2023 by

Example 1 - If a line intersects sides AB and AC of ABC - Theorem 6.1

Example 1 - Chapter 6 Class 10 Triangles - Part 2

This video is only available for Teachoo black users

Subscribe Now

Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class

Book a free demo

Transcript

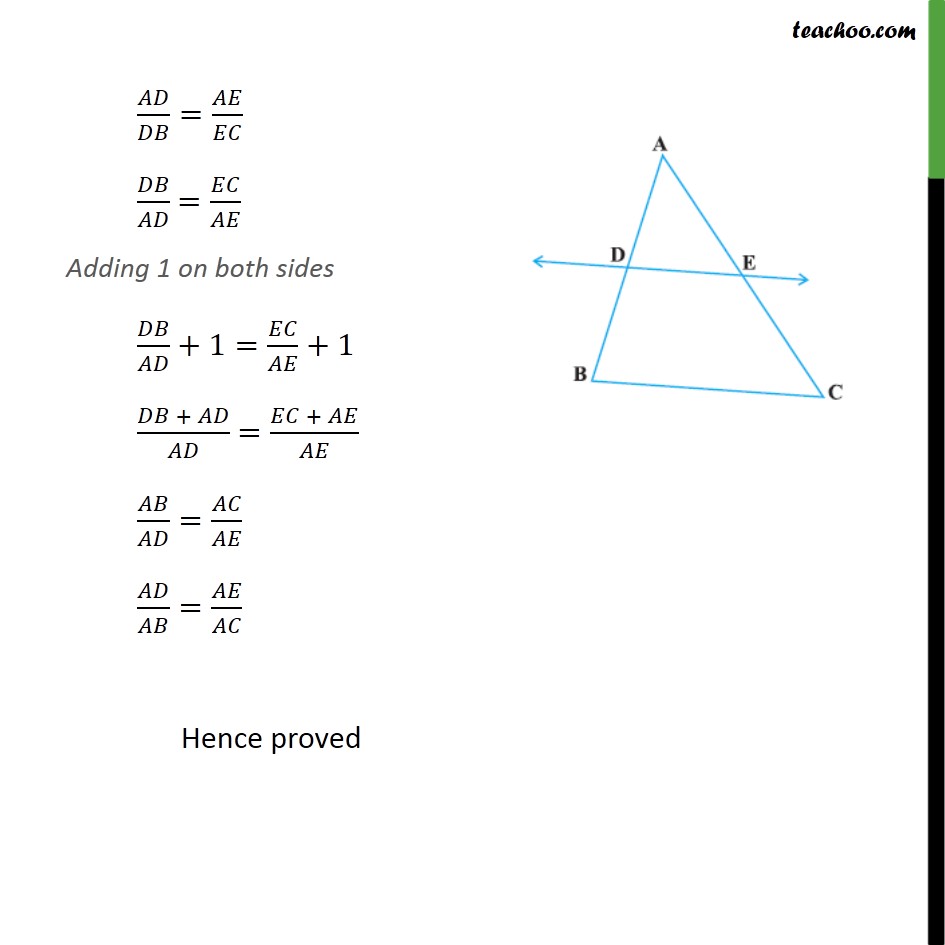
Example 1 If a line intersects sides AB and AC of a Δ ABC at D and E respectively and is parallel to BC, prove that 𝐴𝐷/𝐴𝐵=𝐴𝐸/𝐴𝐶 Given: Δ ABC , where line intersects sides AB and AC at D and E. And DE II BC To Prove : 𝐴𝐷/𝐴𝐵=𝐴𝐸/𝐴𝐶 Proof: We know that if a line drawn parallel to one side of triangle, intersects the other two sides in distinct points, then it divides the other 2 side in same ratio Therefore , 𝐴𝐷/𝐷𝐵=𝐴𝐸/𝐸𝐶 𝐴𝐷/𝐷𝐵=𝐴𝐸/𝐸𝐶 𝐷𝐵/𝐴𝐷 =𝐸𝐶/𝐴𝐸 Adding 1 on both sides 𝐷𝐵/𝐴𝐷+1=𝐸𝐶/𝐴𝐸+1 (𝐷𝐵 + 𝐴𝐷)/𝐴𝐷 =(𝐸𝐶 + 𝐴𝐸)/𝐴𝐸 𝐴𝐵/𝐴𝐷=𝐴𝐶/𝐴𝐸 𝐴𝐷/𝐴𝐵=𝐴𝐸/𝐴𝐶 Hence proved

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.