Check sibling questions

Ex 6.3, 12 - Chapter 6 Class 10 Triangles (Important Question)

Last updated at May 29, 2018 by

Ex 6.3, 12 - Sides AB and BC and median AD of a ABC - Ex 6.3

Ex 6.3, 12 - Chapter 6 Class 10 Triangles - Part 2

This video is only available for Teachoo black users

Subscribe Now

Introducing your new favourite teacher - Teachoo Black, at only ₹83 per month

Join Teachoo Black

Transcript

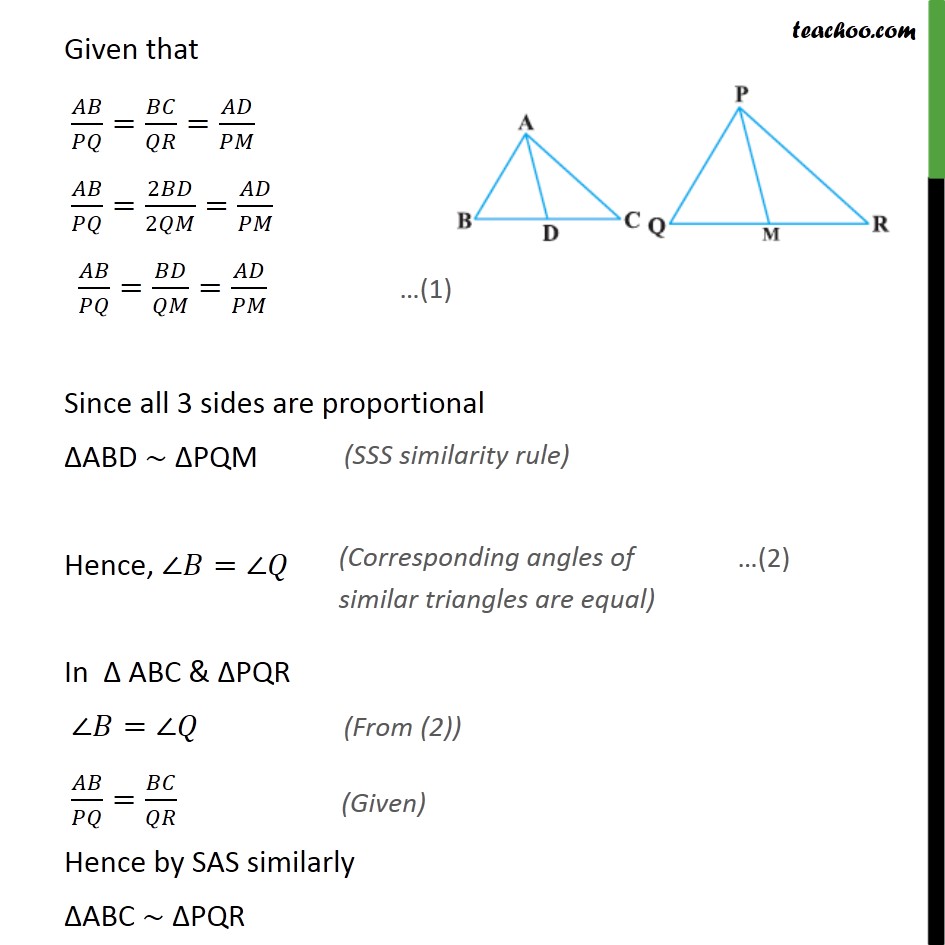
Ex 6.3, 12 Sides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of PQR (see figure). Show that ABC PQR. Given: ABC where AD is the median PQR where PM is the median & / = / = / To Prove: ABC PQR. Proof:- Since AD is the median, BD = CD = 1/2 BC Similarly, PM is the median, QM = RM = 1/2QR Given that / = / = / / =2 /2 = / / = / = / Since all 3 sides are proportional ABD PQM Hence, = In ABC & PQR = / = / Hence by SAS similarly ABC PQR

Davneet Singh's photo - Teacher, Engineer, Marketer

Made by

Davneet Singh

Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 12 years. He provides courses for Maths and Science at Teachoo.