If a ̂ and b ̂ are unit vectors, then prove that
|a ̂+b ̂ |= 2𝑐𝑜𝑠 θ/2, where 𝜃 is the angle between them.
This question is similar to MIsc 17 (MCQ) - Chapter 10 Class 12 - Vector Algebra
![[SQP] If 𝑎̂ and 𝑏̂ are unit vectors, then prove that |a + b| = 2 cos - CBSE Class 12 Sample Paper for 2022 Boards (For Term 2)](https://cdn.teachoo.com/cda28acf-5b35-4c32-90ab-44abbb10d9e0/slide9.jpg)
CBSE Class 12 Sample Paper for 2022 Boards (For Term 2)
CBSE Class 12 Sample Paper for 2022 Boards (For Term 2)
Last updated at Dec. 14, 2024 by Teachoo
This question is similar to MIsc 17 (MCQ) - Chapter 10 Class 12 - Vector Algebra
![[SQP] If 𝑎̂ and 𝑏̂ are unit vectors, then prove that |a + b| = 2 cos - CBSE Class 12 Sample Paper for 2022 Boards (For Term 2)](https://cdn.teachoo.com/cda28acf-5b35-4c32-90ab-44abbb10d9e0/slide9.jpg)


Transcript
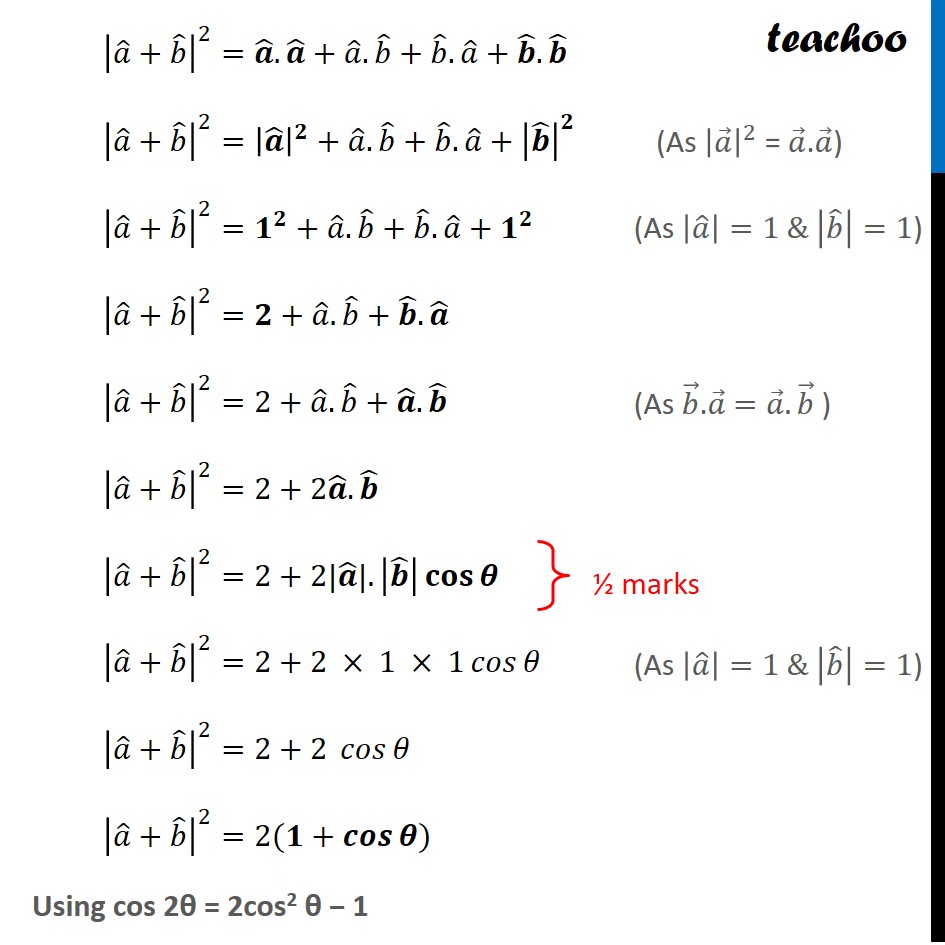
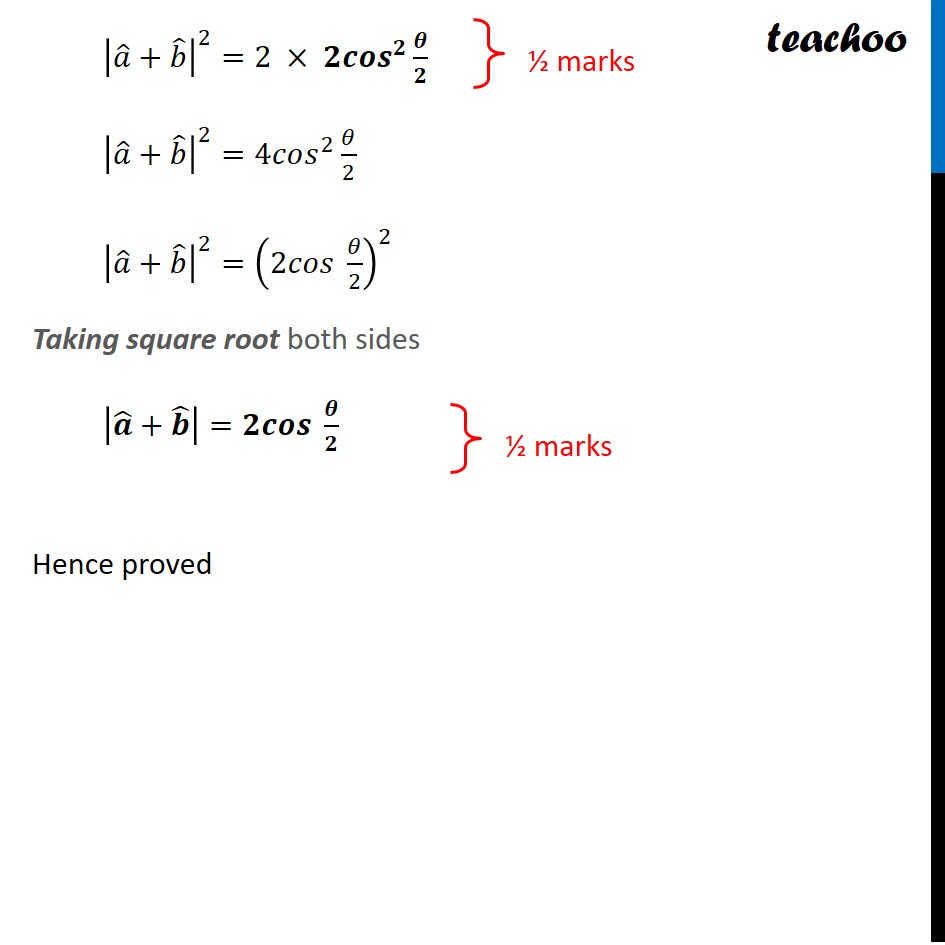
Question 3 If 𝑎 ̂ and 𝑏 ̂ are unit vectors, then prove that |𝑎 ̂+𝑏 ̂ |= 2𝑐𝑜𝑠 𝜃/2, where 𝜃 is the angle between them. Given 𝑎 ̂ & 𝑏 ̂ are unit vectors, So, |𝒂 ̂ | = 1 & |𝒃 ̂ | = 1 We need to find |𝒂 ̂+𝒃 ̂ | Let’s find |𝒂 ̂+𝒃 ̂ |^𝟐 instead Now, |𝒂 ̂+𝒃 ̂ |^𝟐=(𝒂 ̂+𝒃 ̂ ).(𝒂 ̂+𝒃 ̂ ) |𝑎 ̂+𝑏 ̂ |^2=𝑎 ̂.(𝑎 ̂+𝑏 ̂ )+𝑏 ̂.(𝑎 ̂+𝑏 ̂ ) (As |𝑎 ⃗ |^2 = 𝑎 ⃗.𝑎 ⃗) |𝑎 ̂+𝑏 ̂ |^2=𝒂 ̂.𝒂 ̂+𝑎 ̂.𝑏 ̂+𝑏 ̂.𝑎 ̂+𝒃 ̂.𝒃 ̂ |𝑎 ̂+𝑏 ̂ |^2=|𝒂 ̂ |^𝟐+𝑎 ̂.𝑏 ̂+𝑏 ̂.𝑎 ̂+|𝒃 ̂ |^𝟐 |𝑎 ̂+𝑏 ̂ |^2=𝟏^𝟐+𝑎 ̂.𝑏 ̂+𝑏 ̂.𝑎 ̂+𝟏^𝟐 |𝑎 ̂+𝑏 ̂ |^2=𝟐+𝑎 ̂.𝑏 ̂+𝒃 ̂.𝒂 ̂ |𝑎 ̂+𝑏 ̂ |^2=2+𝑎 ̂.𝑏 ̂+𝒂 ̂.𝒃 ̂ |𝑎 ̂+𝑏 ̂ |^2=2+2𝒂 ̂.𝒃 ̂ |𝑎 ̂+𝑏 ̂ |^2=2+2|𝒂 ̂|.|𝒃 ̂ | 𝐜𝐨𝐬𝜽 |𝑎 ̂+𝑏 ̂ |^2=2+2 × 1 × 1 𝑐𝑜𝑠𝜃 |𝑎 ̂+𝑏 ̂ |^2=2+2 𝑐𝑜𝑠𝜃 |𝑎 ̂+𝑏 ̂ |^2=2(𝟏+𝒄𝒐𝒔𝜽) Using cos 2θ = 2cos2 θ − (As |𝑎 ⃗ |^2 = 𝑎 ⃗.𝑎 ⃗) (As |𝑎 ̂ |=1 & |𝑏 ̂ |=1) (As |𝑎 ̂ |=1 & |𝑏 ̂ |=1) |𝑎 ̂+𝑏 ̂ |^2=2 × 𝟐𝒄𝒐𝒔^𝟐 𝜽/𝟐 |𝑎 ̂+𝑏 ̂ |^2=4𝑐𝑜𝑠^2 𝜃/2 |𝑎 ̂+𝑏 ̂ |^2=(2𝑐𝑜𝑠 𝜃/2)^2 Taking square root both sides |𝒂 ̂+𝒃 ̂ |=𝟐𝒄𝒐𝒔 𝜽/𝟐 Hence proved