Ex 5.2, 1 - Chapter 5 Class 10 Arithmetic Progressions

Transcript

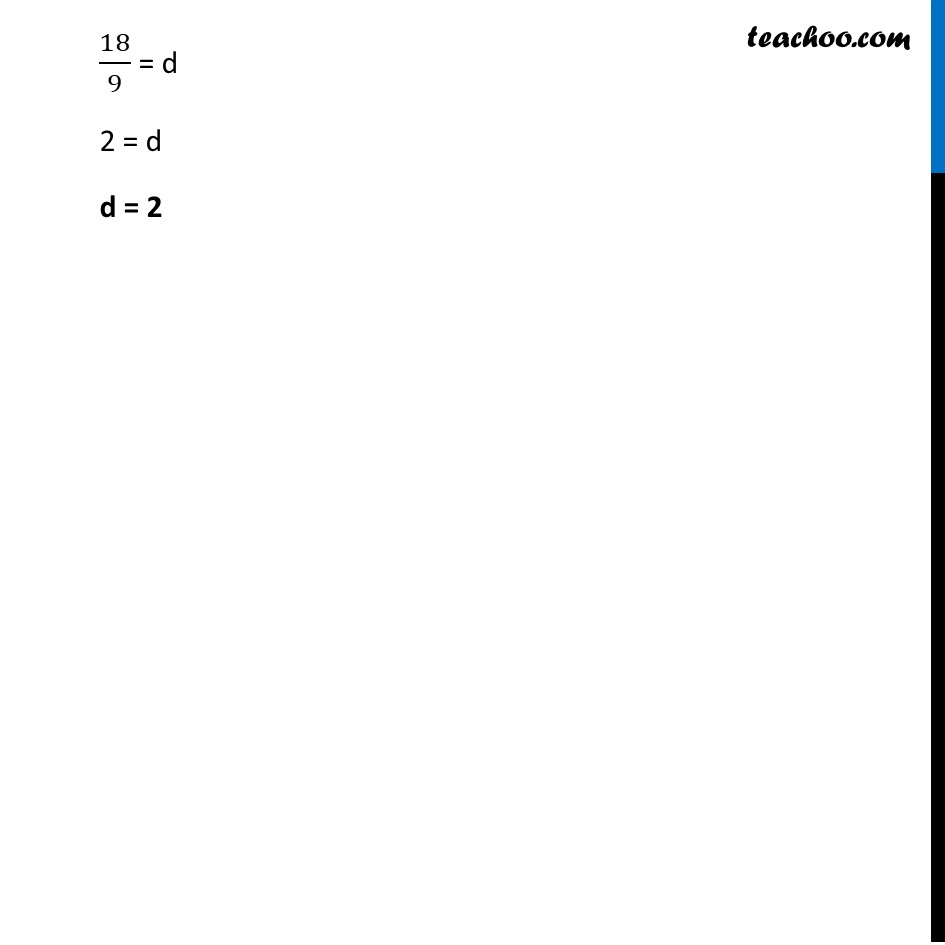
Ex 5.2, 1 Fill in the blanks in the following table, given that a is the first term, d the common difference and an the nth term of the A.P. (i) Here, a = 7 , d = 3 , n = 8 Now, an = a + (n – 1) d Putting values = 7 + (8 – 1) × 3 = 7 + 7 × 3 = 7 + 21 = 28 Ex 5.2, 1 Fill in the blanks in the following table, given that a is the first term, d the common difference and anthe nth term of the A.P. (ii) Here, a = –18 , n = 10 , an = 0 an = a + (n – 1) d Putting values 0 = – 18 + (10 – 1) × d 0 = – 18 + (9) × d 0 + 18 = 9d 18/9 = d 2 = d d = 2 Ex 5.2, 1 Fill in the blanks in the following table, given that a is the first term, d the common difference and an the nth term of the A.P. (iii) Here, d = –3 , n = 18 , an = – 5 Now, an = a + (n – 1) d Putting values –5 = a + (18 – 1) × – 3 –5 = a + 17 × – 3 –5 = a – 51 –5 + 51 = a 46 = a a = 46 Ex 5.2, 1 Fill in the blanks in the following table, given that a is the first term, d the common difference and an the nth term of the A.P. (iv) Here, a = –18.9, d = 2.5 , an = 3.6 Now, an = a + (n – 1) d Putting values 3.6 = –18.9 + (n – 1) × 2.5 3.6 = –18.9 + 2.5 n – 2.5 3.6 = –21.4 + 2.5 n 3.6 + 21.4 = 2.5 n 25 = 2.5 n 25/2.5 = n 10 = n n = 10 Ex 5.2, 1 Fill in the blanks in the following table, given that a is the first term, d the common difference and an the nth term of the A.P. (v) Given a = 3.5, d = 0 , n = 105 Now, an = a + (n – 1) d Putting values = 3.5 + (105 – 1) × 0 = 3.5 + 104 × 0 = 3.5 + 0 = 3.5 Hence, an = 3.5