Ex 5.1, 4 (i) - (v) - Chapter 5 Class 10 Arithmetic Progressions

Transcript

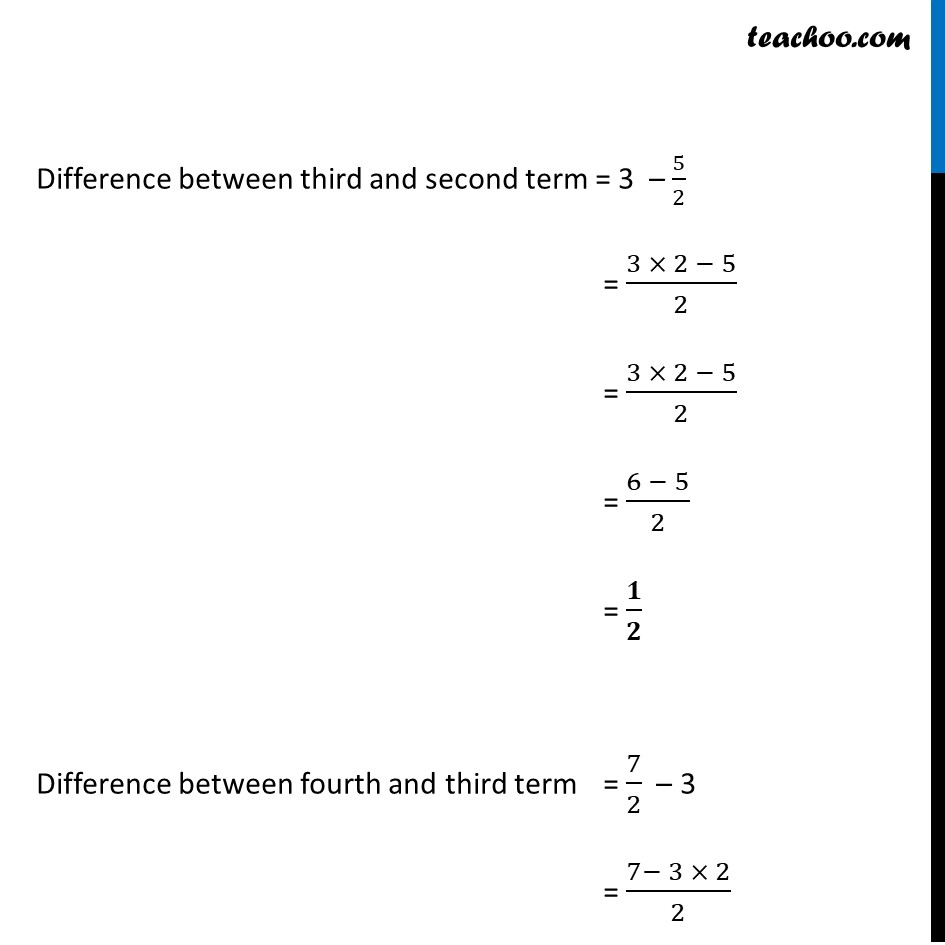
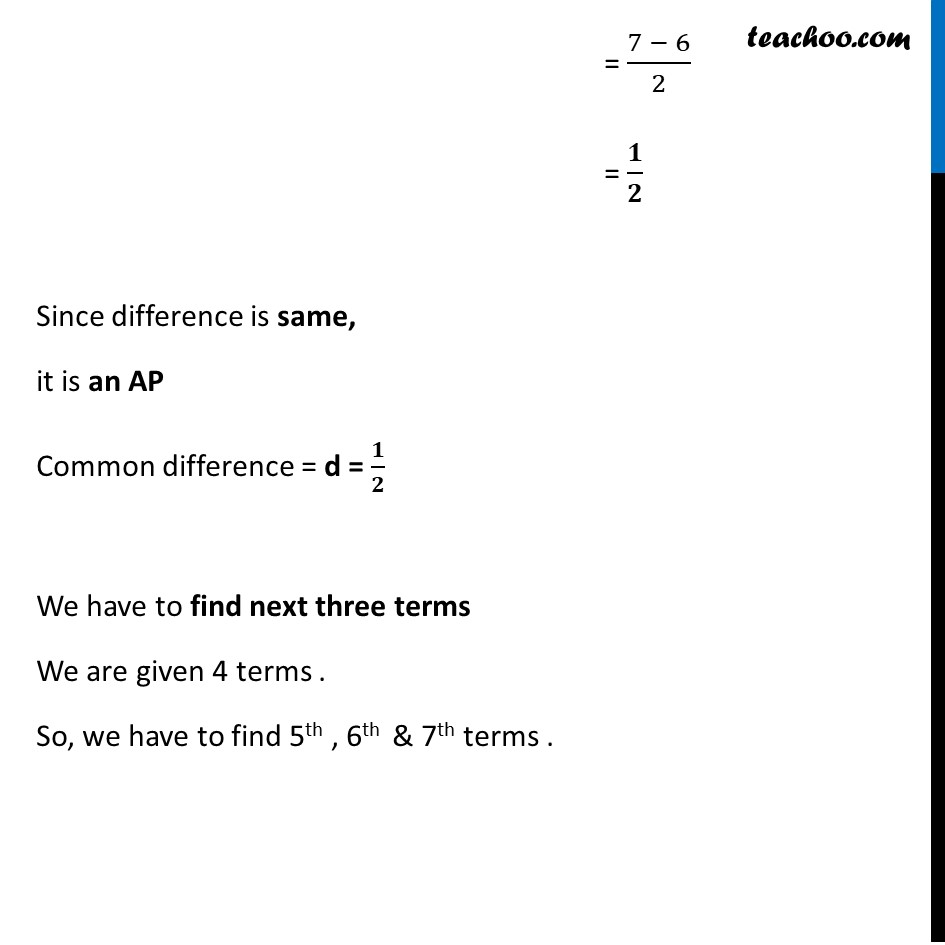
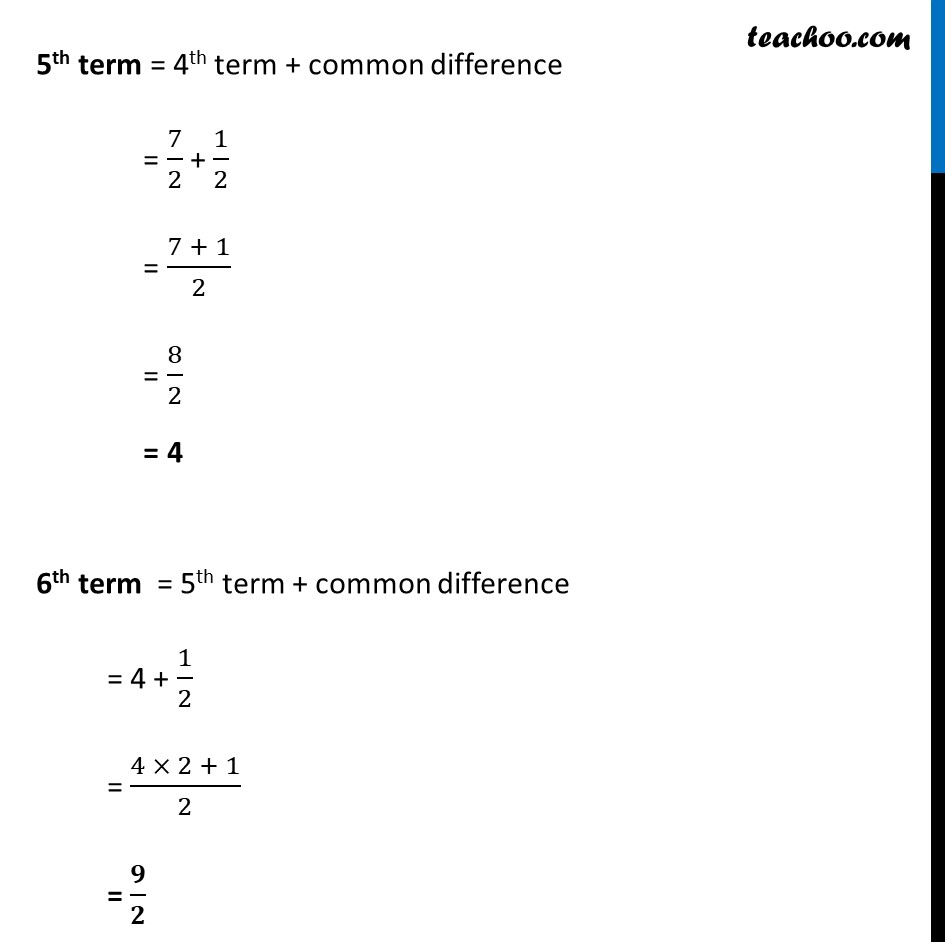
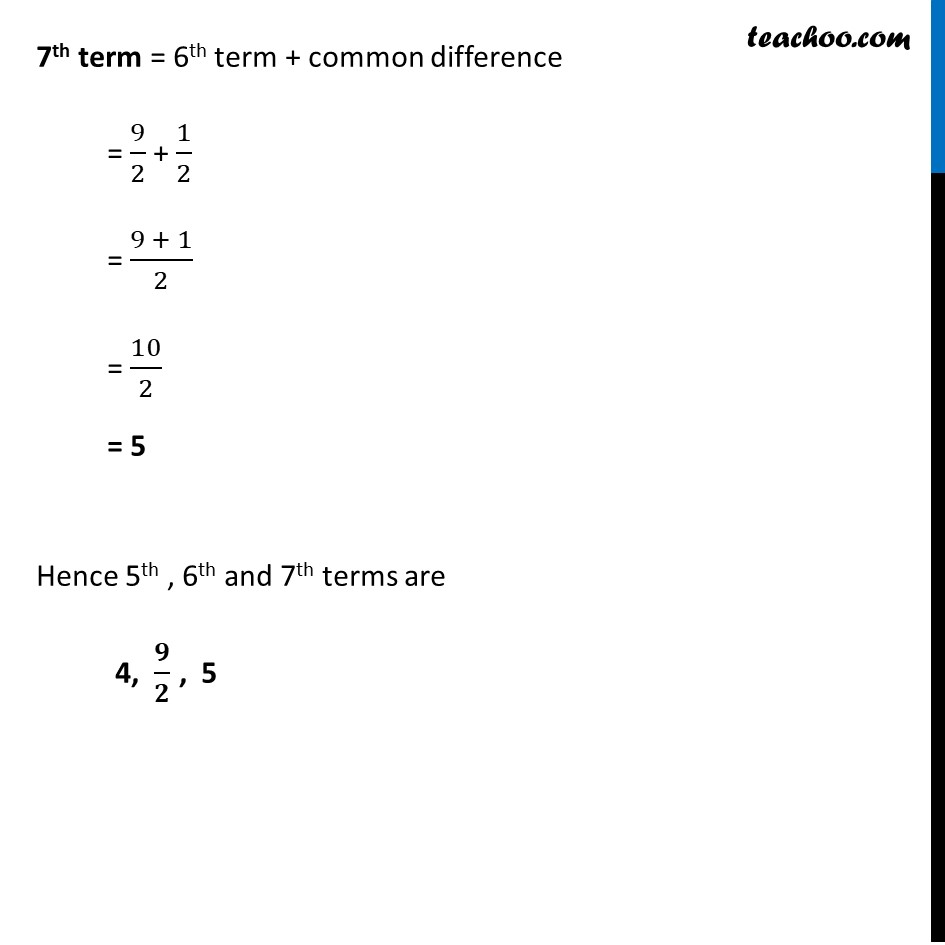
Ex 5.1, 4 Which of the following are APs? If they form an A.P. Find the common difference d and write three more terms. (i) 2, 4, 8, 16 … 2, 4, 8, 16….. Difference of second and first term = 4 – 2 = 2 Difference of third and second term = 8 – 4 = 4 Since 2 ≠ 4 Difference is not same Hence, it is not an AP Ex 5.1, 4 Which of the following are APs? If they form an A.P. Find the common difference d and write three more terms. (ii) 2, 5/2, 3, 7/2….. 2, 5/2 , 3, 7/2 , ….. Difference between second and first term = 5/2 – 2 = (5 − 2 × 2)/2 = (5 − 4)/2 = 𝟏/𝟐 Difference between third and second term = 3 – 5/2 = (3 × 2 − 5)/2 = (3 × 2 − 5)/2 = (6 − 5)/2 = 𝟏/𝟐 Difference between fourth and third term = 7/2 – 3 = (7− 3 × 2)/2 = (7 − 6)/2 = 𝟏/𝟐 Since difference is same, it is an AP Common difference = d = 𝟏/𝟐 We have to find next three terms We are given 4 terms . So, we have to find 5th , 6th & 7th terms . 5th term = 4th term + common difference = 7/2 + 1/2 = (7 + 1)/2 = 8/2 = 4 6th term = 5th term + common difference = 4 + 1/2 = (4 × 2 + 1)/2 = 𝟗/𝟐 7th term = 6th term + common difference = 9/2 + 1/2 = (9 + 1)/2 = 10/2 = 5 Hence 5th , 6th and 7th terms are 4, 𝟗/𝟐 , 5 Ex 5.1, 4 Which of the following are APs? If they form an A.P. find the common difference d and write three more terms. (iii) − 1.2, − 3.2, − 5.2, − 7.2 … –1.2, –3.2, –5.2, –7.2 Difference between second and first term = –3.2 – (–1.2) = –3.2 + 1.2 = –2.0 = –2 Difference between third and second term = –5.2 – ( – 3.2) = –5.2 + 3.2 = –2.0 = –2 Difference between fourth and third term = –7.2 – – 5.2 = –7.2 + 5.2 = –2.0 = –2 Since difference is same, it is an AP Common difference = d = –2 We have to find next three terms We are given 4 terms . So, we have to find 5th , 6th & 7th terms . 5th term = Fourth term + Common Difference = –7.2 + (–2) = –7.2 – 2 = –9.2 6th term = Fifth term + Common Difference = –9.2 + (–2) = –9.2 – 2 = –11.2 7th term = Sixth term + Common Difference = –11.2 + (–2) = –11.2 – 2 = –13.2 Hence 5th , 6th and 7th terms are – 9.2, –11.2, –13.2 Ex 5.1, 4 Which of the following are APs? If they form an A.P. find the common difference d and write three more terms. (iv) − 10, − 6, − 2, 2 … –10, –6, –2, 2, …. Difference between 2nd and 1st term = –6 – (–10) = –6 + 10 = 4 Difference between third and second term = – 2 – (–6) = – 2 + 6 = 4 Difference between fourth and third term = 2 – (–2) = 2 + 2 = 4 Since difference is same, it is an AP Common difference = d = 4 We have to find next three terms We are given 4 terms . So, we have to find 5th , 6th & 7th terms. 5th term = Fourth term + Common Difference = 2 + 4 = 6 6th term = Fifth term + Common Difference = 6 + 4 = 10 7th term = Sixth term + Common Difference = 10 + 4 = 14 Hence 5th , 6th and 7th terms are 6, 10 , 14 Ex 5.1, 4 Which of the following are APs? If they form an A.P. find the common difference d and write three more terms. (v) 3,3 + √2, 3 + 2 √2,3 + 3 √2…….. 3, 3 + √2 , 3 + 2√2 , 3 + 3√2 , ……. Difference between second and first term = 3 + √2 – 3 = √𝟐 Difference between third and second term = (3 + 2√2 ) – (3 + √2 ) = 3 + 2√2 – 3 – √2 = 3 – 3 + 2√2 – √2 = 0 + √2 (2 – 1) = √2 (1) = √𝟐 Difference between fourth and third term = (3 + 3√2 ) – (3 + 2√2) = 3 + 3√2 – 3 – 2√2 = 3 – 3 + 3√2 – 2√2 = 0 + 3√2 – 2√2 = √2 (3 – 2 ) = √2 × 1 = √𝟐 Since difference is same, it is an AP Common difference = d = √𝟐 We have to find next three terms We are given 4 terms . So, we have to find 5th , 6th & 7th terms. 5th term = Fourth term + Common Difference = (3 + 3√2) + √2 = 3 + √2 [ 3 + 1] = 3 + √2 (4) = 3 + 4 √𝟐 6th term = 5th term + Common Difference = (3 + 4√2) + √2 = 3 + √2 (4 + 1) = 3 + √2 × 5 = 3 + 5 √𝟐 Similarly , 7th term = 6th term + Common Difference = (3 + 5 √2) + √2 = 3 + √2 (5 + 1) = 3 + √2 × 6 = 3 + 6 √𝟐