Check sibling questions

Question 3 - Examples - Chapter 4 Class 10 Quadratic Equations

Last updated at May 29, 2023 by

Example 9 - Find roots of 4x2 + 3x + 5 = 0 by completing sq - Solving by completing square

Example 9 - Chapter 4 Class 10 Quadratic Equations - Part 2
Example 9 - Chapter 4 Class 10 Quadratic Equations - Part 3

Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class

Book a free demo

Transcript

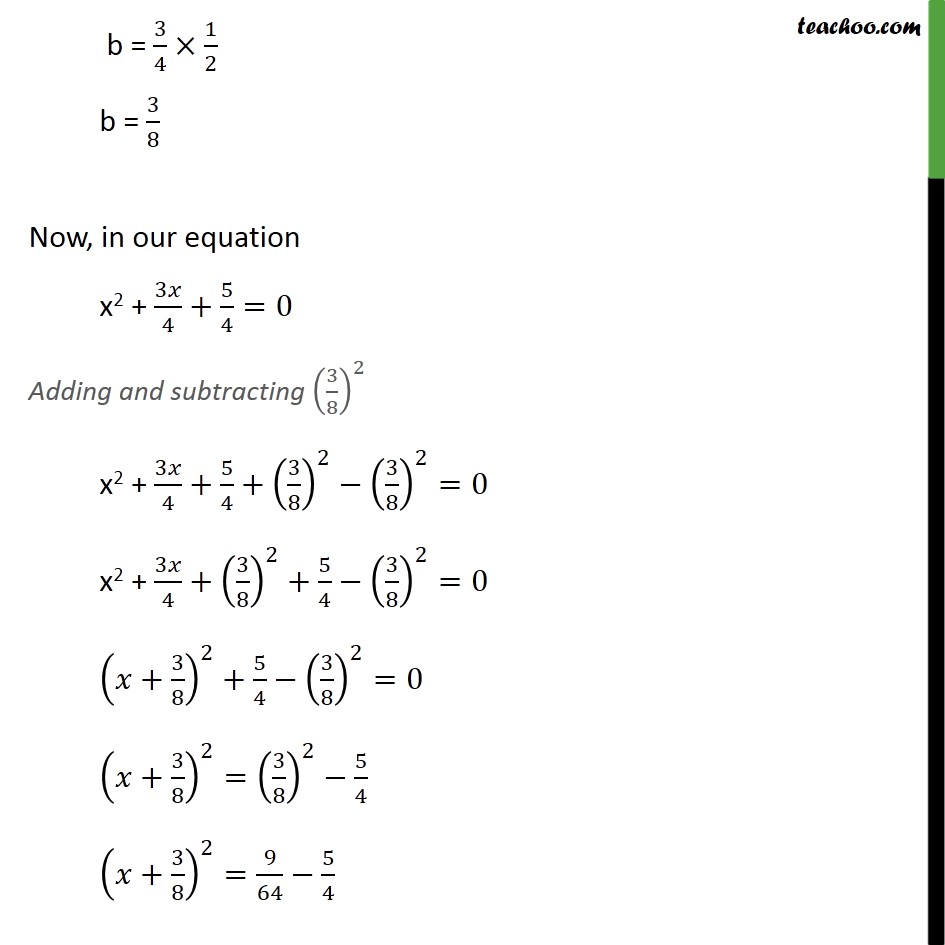
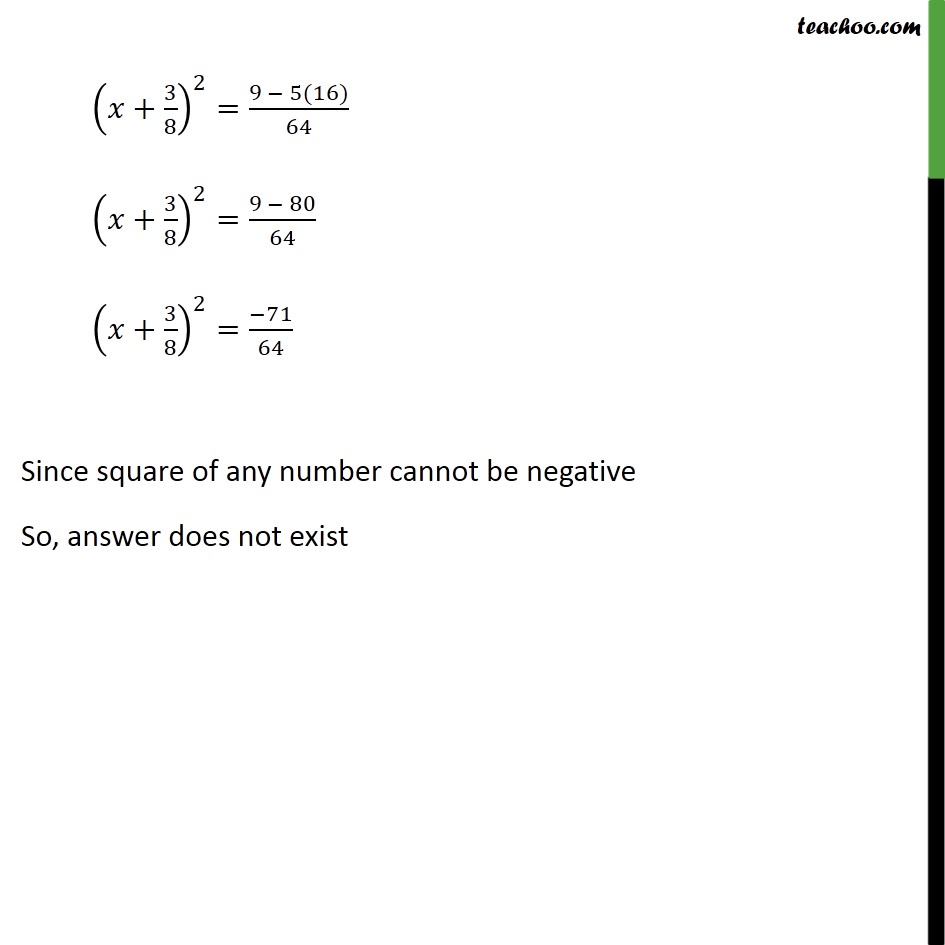
Question 3 Find the roots of 4x2 + 3x + 5 = 0 by the method of completing the square. 4x2 + 3x + 5 = 0 Dividing by 4 4𝑥2/4+3𝑥/4+5/4=0 x2 + 3𝑥/4+5/4=0 We know that (a + b)2 = a2 + 2ab + b2 Here, a = x & 2ab = 3𝑥/4 2xb = 3𝑥/4 2b = 3/4 b = 3/4×1/2 b = 3/8 Now, in our equation x2 + 3𝑥/4+5/4=0 Adding and subtracting (3/8)^2 x2 + 3𝑥/4+5/4+(3/8)^2−(3/8)^2=0 x2 + 3𝑥/4+(3/8)^2+5/4−(3/8)^2=0 (𝑥+3/8)^2+5/4−(3/8)^2=0 (𝑥+3/8)^2=(3/8)^2−5/4 (𝑥+3/8)^2=9/64−5/4 (𝑥+3/8)^2=(9 − 5(16))/64 (𝑥+3/8)^2=(9 − 80)/64 (𝑥+3/8)^2=(−71)/64 Since square of any number cannot be negative So, answer does not exist

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.