Question 5 - Past Year MCQ - Chapter 7 Class 10 Coordinate Geometry

Last updated at August 12, 2026 by

If P (2, 4), Q (0, 3), R (3, 6) and S (5, y) are the vertices of a parallelogram PQRS, then the value of y is

(A) 7Β 

(B) 5

(C) βˆ’7Β 

(D) βˆ’8

This question is simlar to Example 10 - Chapter 7 Class 10 Coordinate Geometry

If P (2, 4), Q (0, 3), R (3, 6) and S (5, y) are vertices of [MCQ] - Past Year MCQ

part 2 - Question 5 - Past Year MCQ - Serial order wise - Chapter 7 Class 10 Coordinate Geometry

Remove Ads Take short quiz All Quiz and Worksheets
Teachoo Β· Class 10 Explore Class 10

Transcript

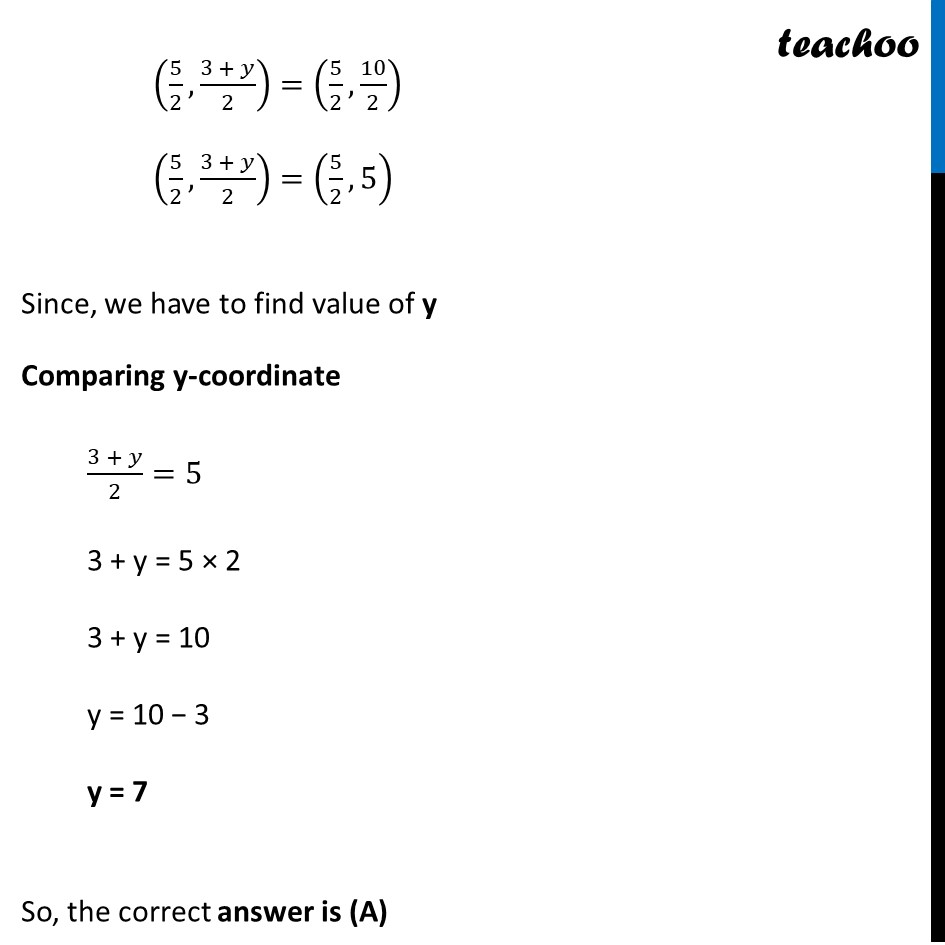
Question 5 If P (2, 4), Q (0, 3), R (3, 6) and S (5, y) are the vertices of a parallelogram PQRS, then the value of y is (A) 7 (B) 5 (C) βˆ’7 (D) βˆ’8 Since PQRS is a parallelogram It’s Diagonals bisect each other Therefore, Mid point of SQ = Mid point of PR ((0 + 5)/2,(3 + 𝑦)/2)=((2 + 3)/2,(4 + 6)/2) (5/2,(3 + 𝑦)/2)=(5/2,10/2) (5/2,(3 + 𝑦)/2)=(5/2,5) Since, we have to find value of y Comparing y-coordinate (3 + 𝑦)/2=5 3 + y = 5 Γ— 2 3 + y = 10 y = 10 βˆ’ 3 y = 7 So, the correct answer is (A)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.