Maximum slope of the curve y = –x 3 + 3x 2 + 9x – 27 is:
(A) 0 (B) 12
(C) 16 (D) 32
Question 15 - NCERT Exemplar - MCQs - Chapter 6 Class 12 Application of Derivatives
Last updated at Dec. 16, 2024 by Teachoo
NCERT Exemplar - MCQs
Question 1
Important
You are here
You are here
Chapter 6 Class 12 Application of Derivatives
Serial order wise
Transcript
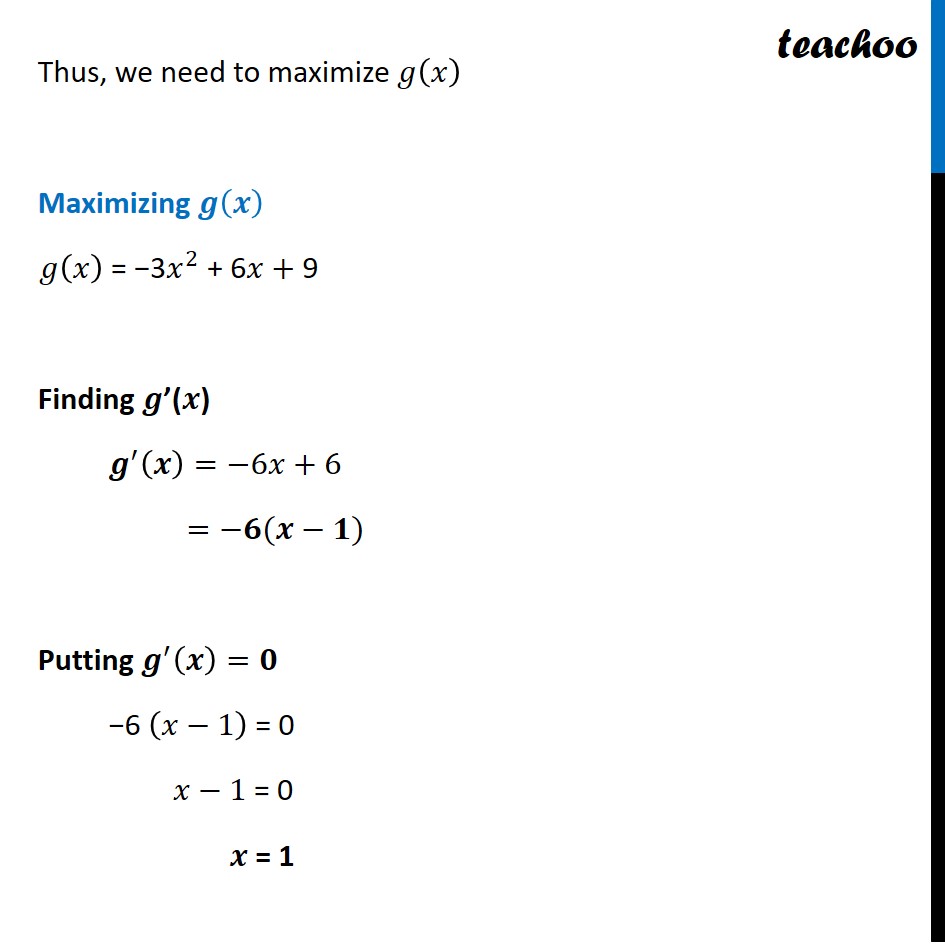
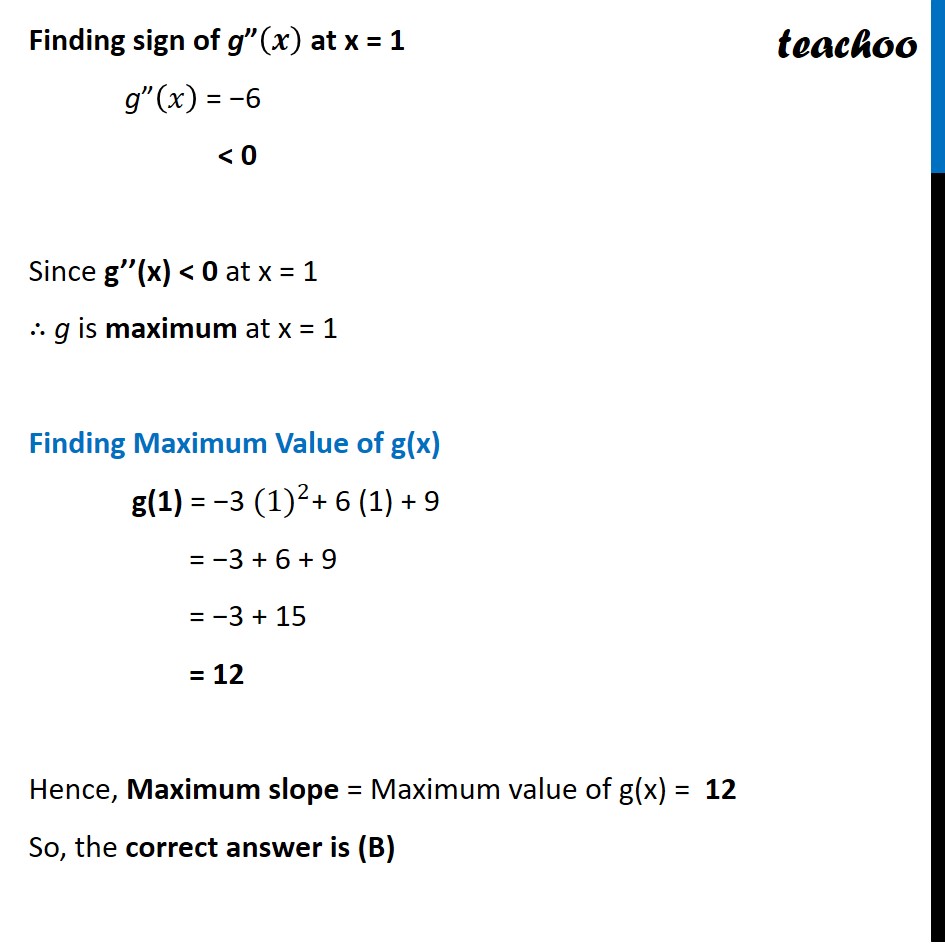
Question 15 Maximum slope of the curve y = βx3 + 3x2 + 9π₯ β 27 is: 0 (B) 12 (C) 16 (D) 32 Given y = β π₯^3 + 3π₯^2 + 9π₯β 27 Now, Slope of the curve =π π/π π = β3π₯^2 + 6π₯+ 9 We need to find maximum slope Letβs assume π(π) = Slope Thus, we need to maximize π(π₯) Maximizing π(π) π(π₯) = β3π₯^2 + 6π₯+ 9 Finding πβ(π) π^β² (π)=β6π₯+6 =βπ(πβπ) Putting π^β² (π)=π β6 (π₯β1) = 0 π₯β1 = 0 π = 1 Finding sign of gβ(π) at x = 1 gβ(π₯) = β6 < 0 Since gββ(x) < 0 at x = 1 β΄ g is maximum at x = 1 Finding Maximum Value of g(x) g(1) = "β3 " γ(1)γ^2 "+ 6 (1) + 9" = β3 + 6 + 9 = β3 + 15 = 12 Hence, Maximum slope = Maximum value of g(x) = 12 So, the correct answer is (B)
