Question 11 - NCERT Exemplar - MCQs - Chapter 6 Class 12 Application of Derivatives

Last updated at April 16, 2024 by

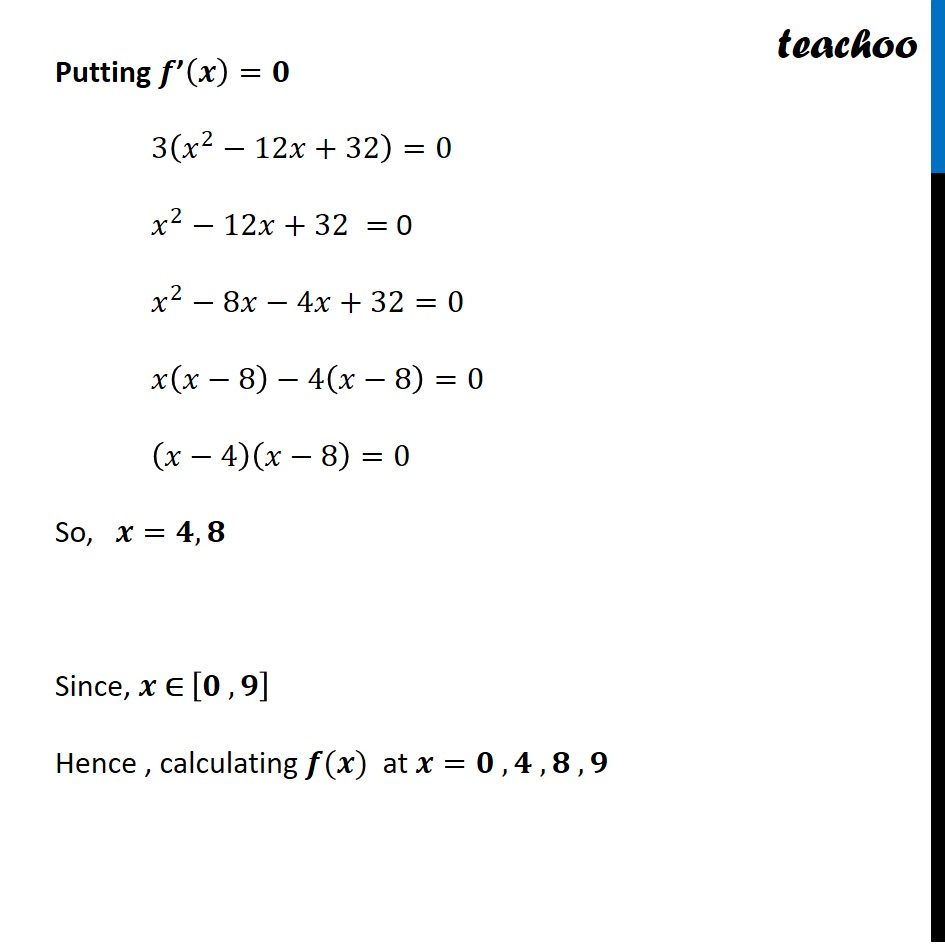
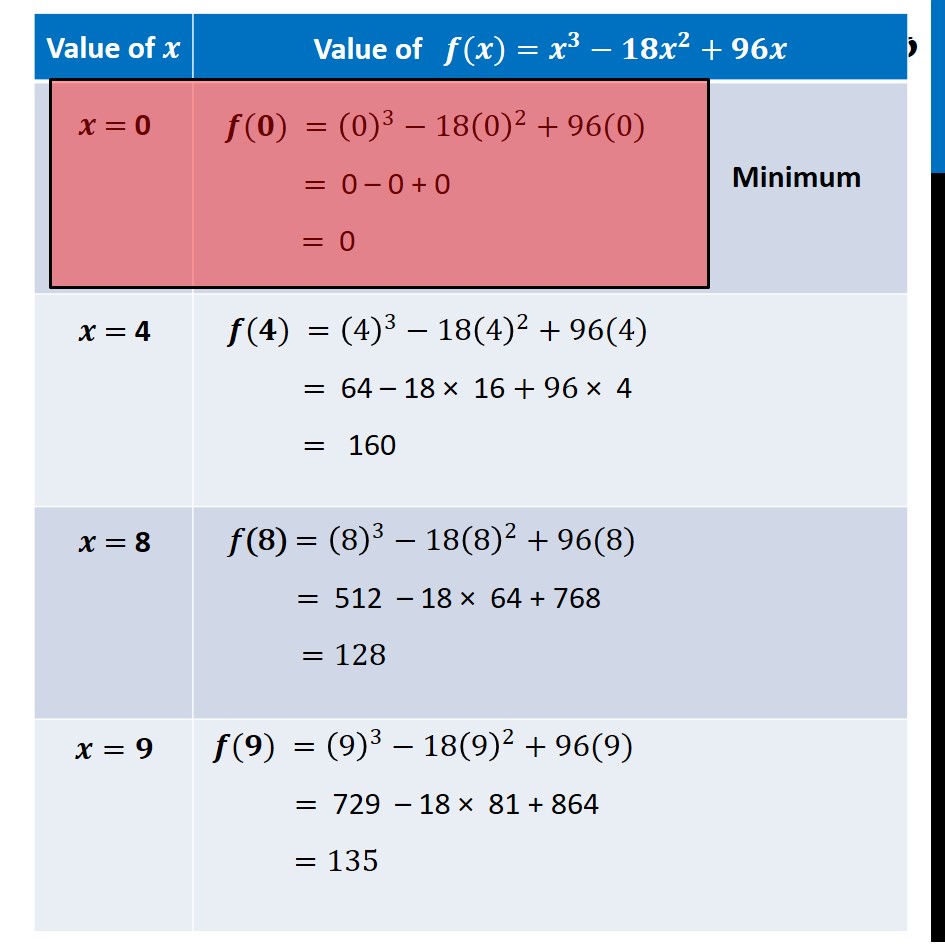
The smallest value of the polynomial x 3 – 18x 2 + 96x in [0, 9] is

(A)126                      (B) 0

(C) 135                    (D) 160

 

This question is similar to Ex 6.5, 7 - Chapter 6 Class 12 - Application of Derivatives

Slide94.JPG

Slide95.JPG
Slide96.JPG
Slide97.JPG

Transcript

Question 11 The smallest value of the polynomial x3 – 18x2 + 96x in [0, 9] is 126 (B) 0 (C) 135 (D) 160 𝒇(𝑥)=𝑥^3−18𝑥^2+96𝑥 Finding 𝒇’(x) 𝒇′(𝒙)=〖3𝑥〗^2−36𝑥+96 𝑓′(𝑥)=𝟑(𝒙^𝟐−𝟏𝟐𝒙+𝟑𝟐) Putting 𝒇’(𝒙)=𝟎 3(𝑥^2−12𝑥+32)=0 𝑥^2−12𝑥+32 = 0 𝑥^2−8𝑥−4𝑥+32=0 𝑥(𝑥−8)−4(𝑥−8)=0 (𝑥−4)(𝑥−8)=0 So, 𝒙=𝟒, 𝟖 Since, 𝒙 ∈ [𝟎 , 𝟗] Hence , calculating 𝒇(𝒙) at 𝒙=𝟎 , 𝟒 , 𝟖 , 𝟗 𝒇(𝟒) =(4)^3−18(4)^2+96(4) = 64 – 18 × 16 + 96 × 4 = 160 𝑓(8) =(8)^3−18(8)^2+96(8) = 512 – 18 × 64 + 768 =128 𝒇(𝟗) =(9)^3−18(9)^2+96(9) = 729 – 18 × 81 + 864 =135 Hence, Minimum value of 𝑓(𝑥) is 0 at 𝒙 = 0 So, the correct answer is (B)

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.