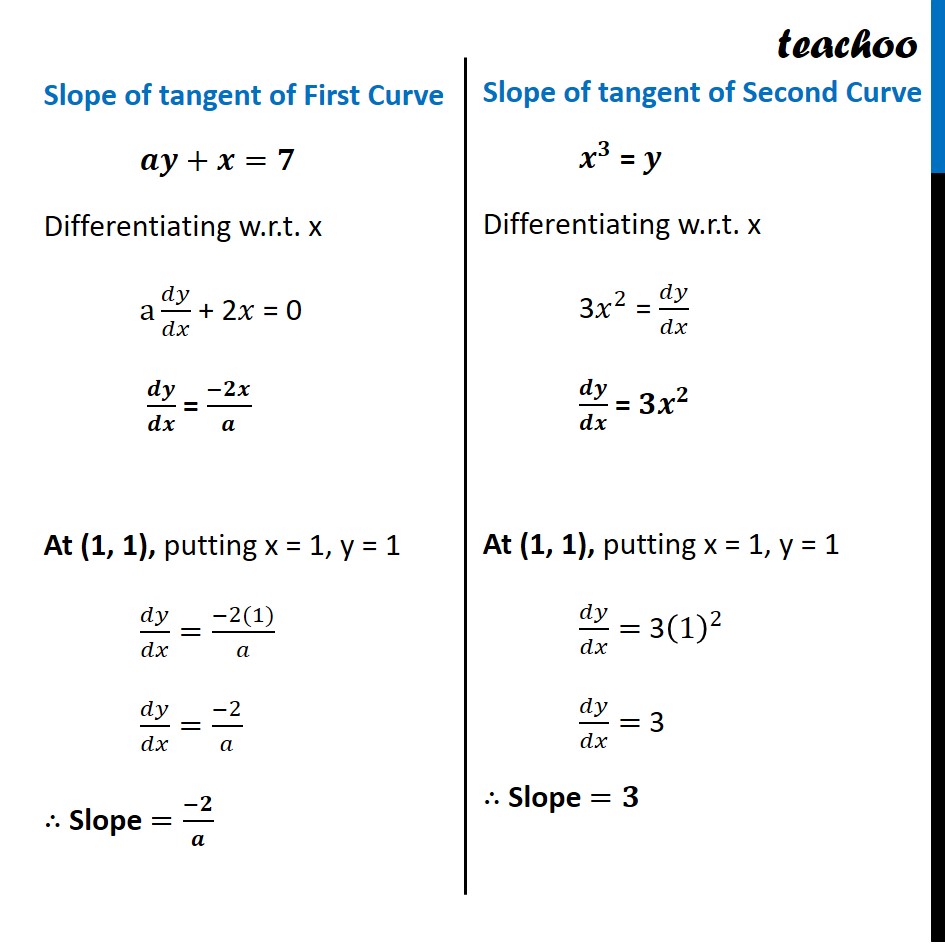
If the curve ay + x 2 = 7 and x 3 = y, cut orthogonally at (1, 1), then the value of a is:
(A)1 (B) 0 (C) –6 (D) 6

NCERT Exemplar - MCQs
Last updated at December 16, 2024 by Teachoo



Transcript
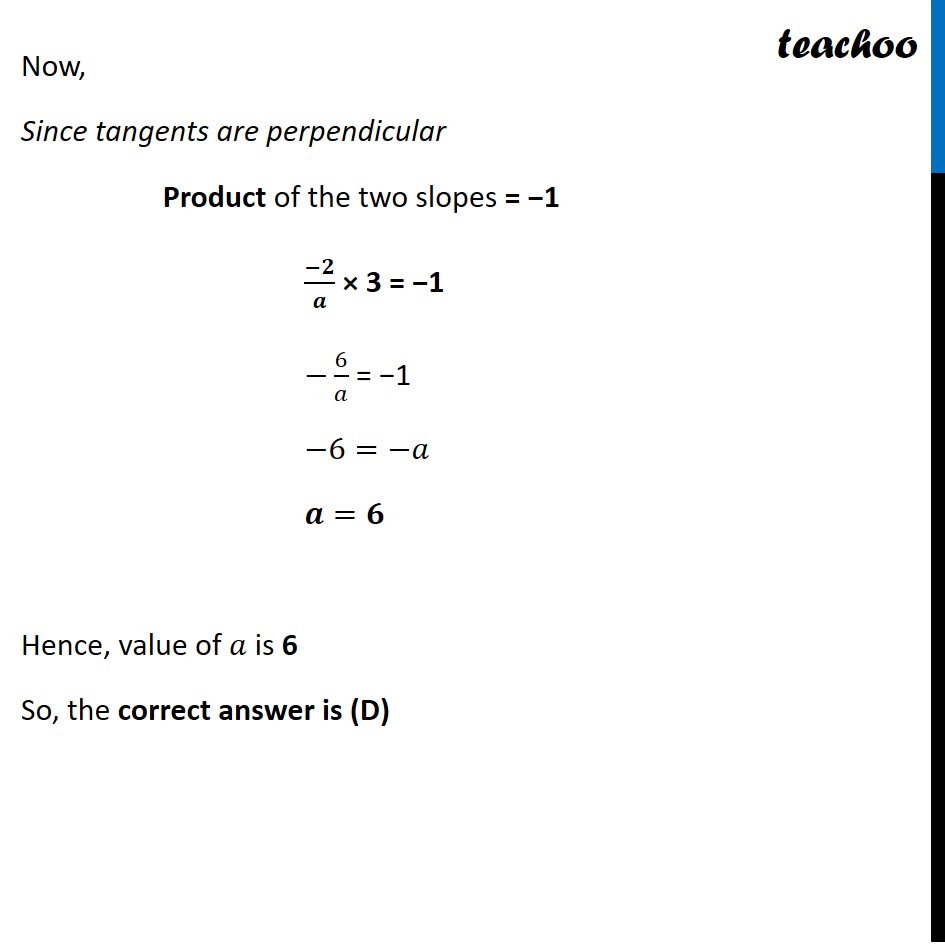
Question 8 If the curve ay + x2 = 7 and x3 = y, cut orthogonally at (1, 1), then the value of a is: 1 (B) 0 (C) –6 (D) 6 Two curves cut each other orthogonally means the cut each other at right angles And, if two curves cut at right angles, then their tangents are perpendicular at point of intersection Finding Slope of Tangents of both curves at (1, 1) Slope of tangent of First Curve 𝒂𝒚+𝒙=𝟕 Differentiating w.r.t. x a 𝑑𝑦/𝑑𝑥 + 2𝑥 = 0 𝒅𝒚/𝒅𝒙 = (−𝟐𝒙)/𝒂 At (1, 1), putting x = 1, y = 1 𝑑𝑦/𝑑𝑥=(−2(1))/𝑎 𝑑𝑦/𝑑𝑥=(−2)/𝑎 ∴ Slope =(−𝟐)/𝒂 Slope of tangent of Second Curve 𝒙^𝟑 = 𝒚 Differentiating w.r.t. x 3𝑥^2 = 𝑑𝑦/𝑑𝑥 𝒅𝒚/𝒅𝒙 = 𝟑𝒙^𝟐 At (1, 1), putting x = 1, y = 1 𝑑𝑦/𝑑𝑥= 3(1)^2 𝑑𝑦/𝑑𝑥= 3 ∴ Slope =𝟑 Now, Since tangents are perpendicular Product of the two slopes = −1 (−𝟐)/𝒂 × 3 = −1 −6/𝑎 = −1 −6=−𝑎 𝒂=𝟔 Hence, value of 𝑎 is 6 So, the correct answer is (D)