Question 6 - NCERT Exemplar - MCQs - Chapter 6 Class 12 Application of Derivatives

Last updated at August 14, 2026 by

The curve y = x^(1/5) has at (0, 0)

(A) a vertical tangent (parallel to y-axis)

(B) a horizontal tangent (parallel to x-axis)

(C) an oblique tangent

(D) no tangent

The curve y = x^(1/5) has at (0, 0) - Class 12 AOD [MCQ] - Teachoo - NCERT Exemplar - MCQs

part 2 - Question 6 - NCERT Exemplar - MCQs - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 3 - Question 6 - NCERT Exemplar - MCQs - Serial order wise - Chapter 6 Class 12 Application of Derivatives

Remove Ads Take short quiz All Quiz and Worksheets
Teachoo · Class 12 Explore Class 12

Transcript

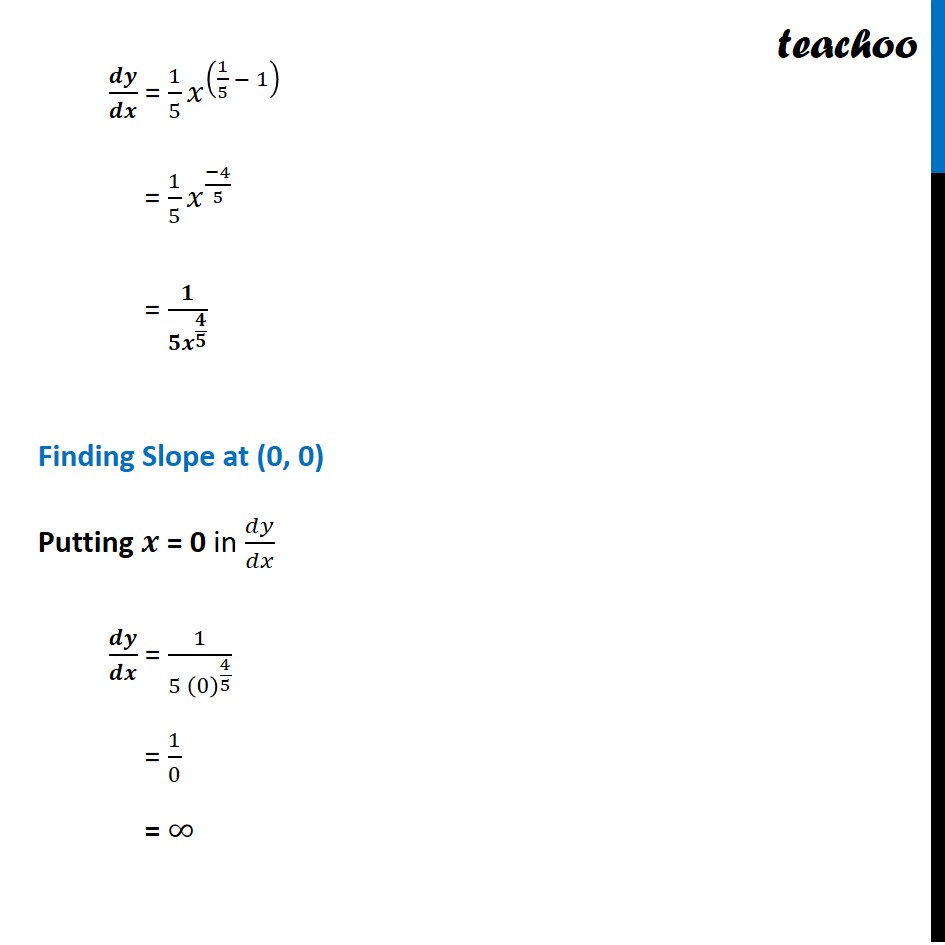
Question 6 The curve y = 𝑥^(1/5) has at (0, 0) (A) a vertical tangent (parallel to y-axis) (B) a horizontal tangent (parallel to x-axis) (C) an oblique tangent (D) no tangent Since options are about finding tangent So, finding slope of tangent i.e. 𝒅𝒚/𝒅𝒙 at (0, 0) Finding 𝒅𝒚/𝒅𝒙 𝑦 = 𝑥^(1/5) 𝒅𝒚/𝒅𝒙 = 1/5 𝑥^((1/5 − 1) ) = 1/5 𝑥^((−4)/5) = 𝟏/(𝟓𝒙^(𝟒/𝟓) ) Finding Slope at (0, 0) Putting 𝒙 = 0 in 𝑑𝑦/𝑑𝑥 𝒅𝒚/𝒅𝒙 = 1/(5 (0)^(4/5) ) = 1/0 = ∞ Now, Slope = tan 𝜽 ∞ = tan 𝜃 tan θ = ∞ ∴ 𝜽 = 90° So tangent makes angle 90° with x-axis Thus, tangent is parallel to y-axis i.e. a vertical tangent So, the correct answer is (A)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.