1. Chapter 6 Class 12 Application of Derivatives (Term 1)
  2. Serial order wise
  3. NCERT Exemplar - MCQs

Question 5 - NCERT Exemplar - MCQs - Chapter 6 Class 12 Application of Derivatives (Term 1)

Last updated at Nov. 23, 2021 by

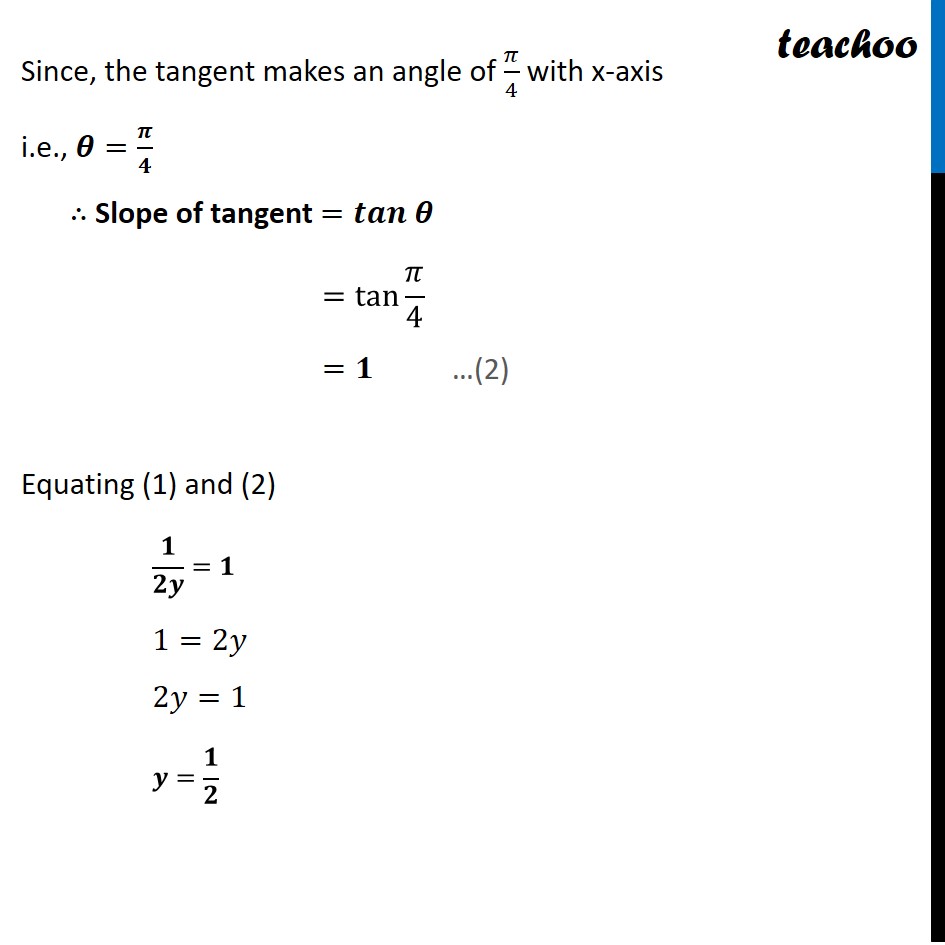
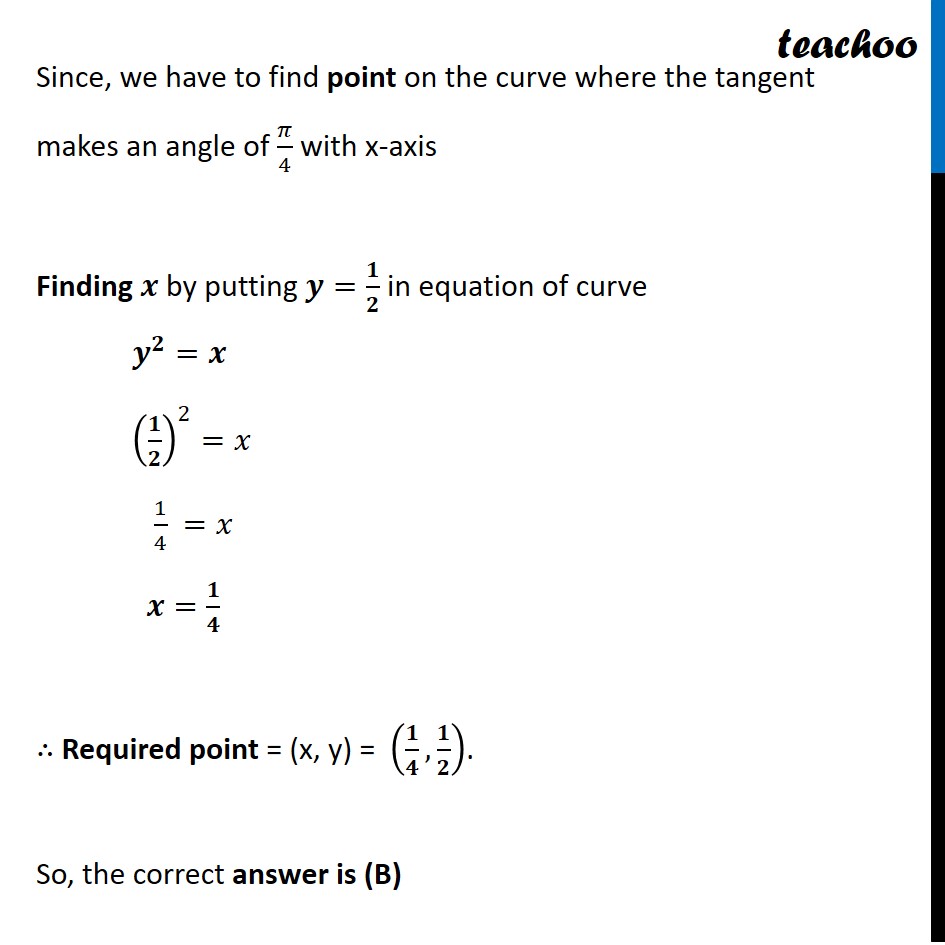
The point on the curve y 2 = x, where the tangent makes an angle of π/4 with x-axis is

(A) (1/2, 1/4)             (B) (1/4, 1/2) 

(C) (4, 2)                   (D) (1, 1)

 

This question is similar to Example 17 - Chapter 6 Class 12 - Application of Derivatives

Slide18.JPG

Slide19.JPG
Slide20.JPG

  1. Chapter 6 Class 12 Application of Derivatives (Term 1)
  2. Serial order wise

    3. NCERT Exemplar - MCQs

    Finding rate of change →

Transcript

Question 5 The point on the curve y2 = x, where the tangent makes an angle of πœ‹/4 with x-axis is (A) (1/2, 1/4) (B) (1/4, 1/2) (C) (4, 2) (D) (1, 1) 𝑦^2=π‘₯ Slope of the tangent is π’…π’š/𝒅𝒙 Finding π’…π’š/𝒅𝒙 2y 𝑑𝑦/𝑑π‘₯=1 π’…π’š/𝒅𝒙=𝟏/πŸπ’š Since, the tangent makes an angle of πœ‹/4 with x-axis i.e., 𝜽=𝝅/πŸ’ ∴ Slope of tangent =𝒕𝒂𝒏 𝜽 =tanβ‘γ€–πœ‹/4γ€— =𝟏 Equating (1) and (2) 𝟏/πŸπ’š=𝟏 1=2𝑦 2𝑦=1 π’š=𝟏/𝟐 …(2) Since, we have to find point on the curve where the tangent makes an angle of πœ‹/4 with x-axis Finding 𝒙 by putting π’š=𝟏/𝟐 in equation of curve π’š^𝟐=𝒙 (𝟏/𝟐)^2=π‘₯ 1/4 =π‘₯ 𝒙=𝟏/πŸ’ ∴ Required point = (x, y) = (𝟏/πŸ’,𝟏/𝟐). So, the correct answer is (B)

Chapter 6 Class 12 Application of Derivatives (Term 1)
Serial order wise

About the Author

Davneet Singh's photo - Teacher, Engineer, Marketer
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 10 years. He provides courses for Maths and Science at Teachoo.