Question 4 - NCERT Exemplar - MCQs - Chapter 6 Class 12 Application of Derivatives

Last updated at July 14, 2026 by

The equation of the normal to the curve y = sin x at (0, 0) is:

(A)x = 0Β  Β  Β  Β  Β  Β  Β  Β  Β  (B) y = 0

(C) x + y = 0Β  Β  Β  Β  Β  Β (D) x – y = 0

Β 

This question is similar to Ex 6.3, 14 (i) - Chapter 6 Class 12 - Application of Derivatives

[MCQ] - The equation of the normal to the curve y = sinx at (0, 0) is: - NCERT Exemplar - MCQs

part 2 - Question 4 - NCERT Exemplar - MCQs - Serial order wise - Chapter 6 Class 12 Application of Derivatives
part 3 - Question 4 - NCERT Exemplar - MCQs - Serial order wise - Chapter 6 Class 12 Application of Derivatives

Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 2 questions, selected from your answers, mistakes, and progress.
Remove Ads Share on WhatsApp

Transcript

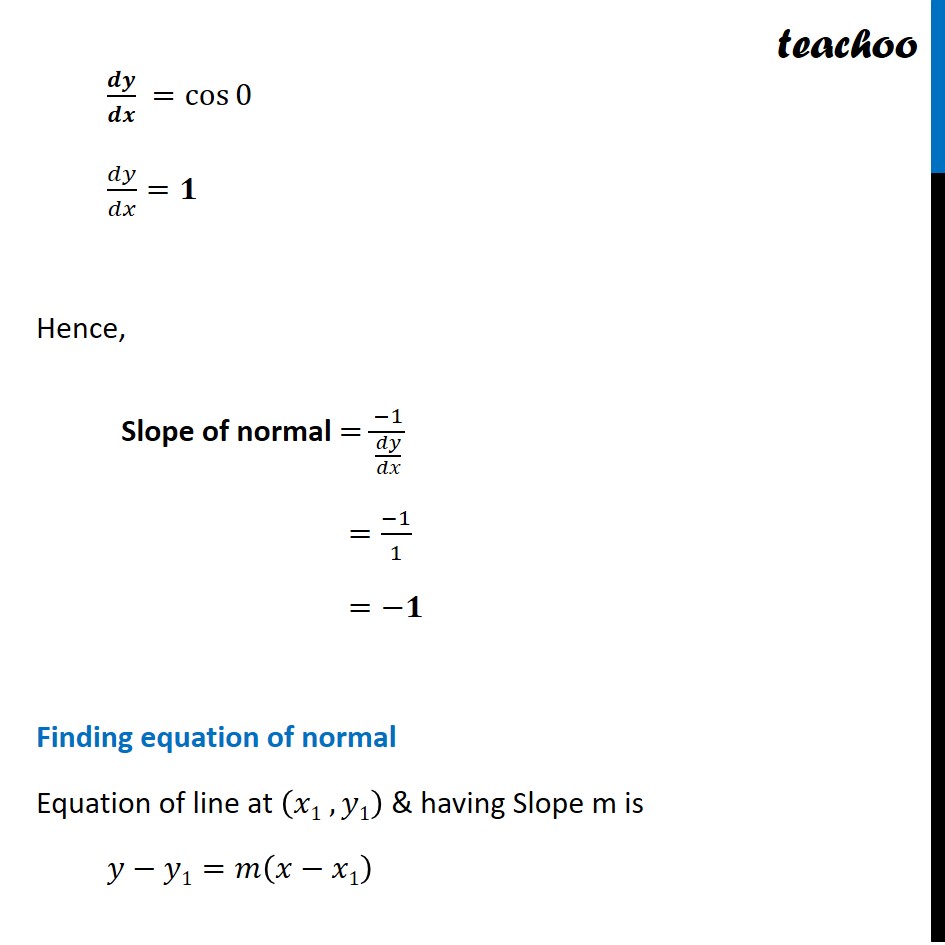
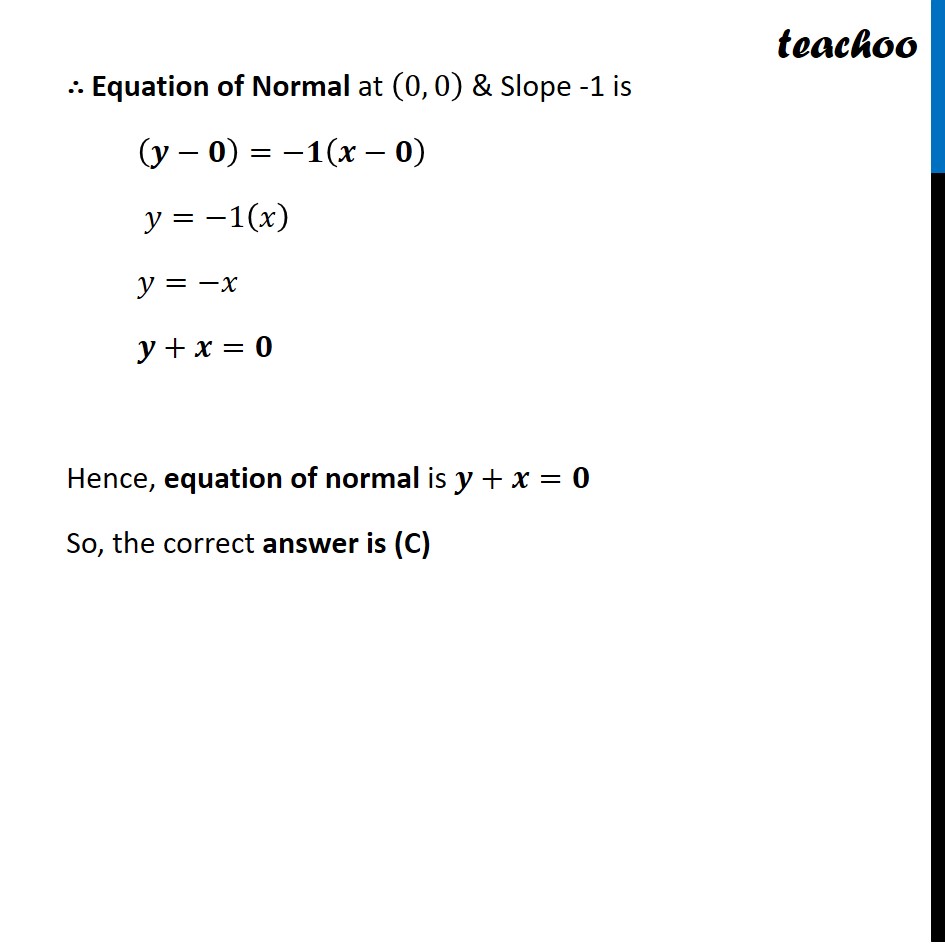
Question 4 The equation of the normal to the curve y = sin x at (0, 0) is: x = 0 (B) y = 0 (C) x + y = 0 (D) x – y = 0 𝑦=sin⁑π‘₯ Since Slope of normal =(βˆ’1)/(𝑑𝑦/𝑑π‘₯) Differentiating 𝑦 w.r.t. π‘₯ 𝑑𝑦/𝑑π‘₯=πœπ¨π¬β‘π’™ Since given point is (0, 0) Putting 𝒙=𝟎 in (1) π’…π’š/𝒅𝒙 =cos⁑0 𝑑𝑦/𝑑π‘₯=𝟏 Hence, Slope of normal =(βˆ’1)/(𝑑𝑦/𝑑π‘₯) =(βˆ’1)/1 =βˆ’πŸ Finding equation of normal Equation of line at (π‘₯1 , 𝑦1) & having Slope m is π‘¦βˆ’π‘¦1=π‘š(π‘₯βˆ’π‘₯1) ∴ Equation of Normal at (0, 0) & Slope -1 is (π’šβˆ’πŸŽ)=βˆ’πŸ(π’™βˆ’πŸŽ) 𝑦=βˆ’1(π‘₯) 𝑦=βˆ’π‘₯ π’š+𝒙=𝟎 Hence, equation of normal is π’š+𝒙=𝟎 So, the correct answer is (C)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.