Example 8

Transcript

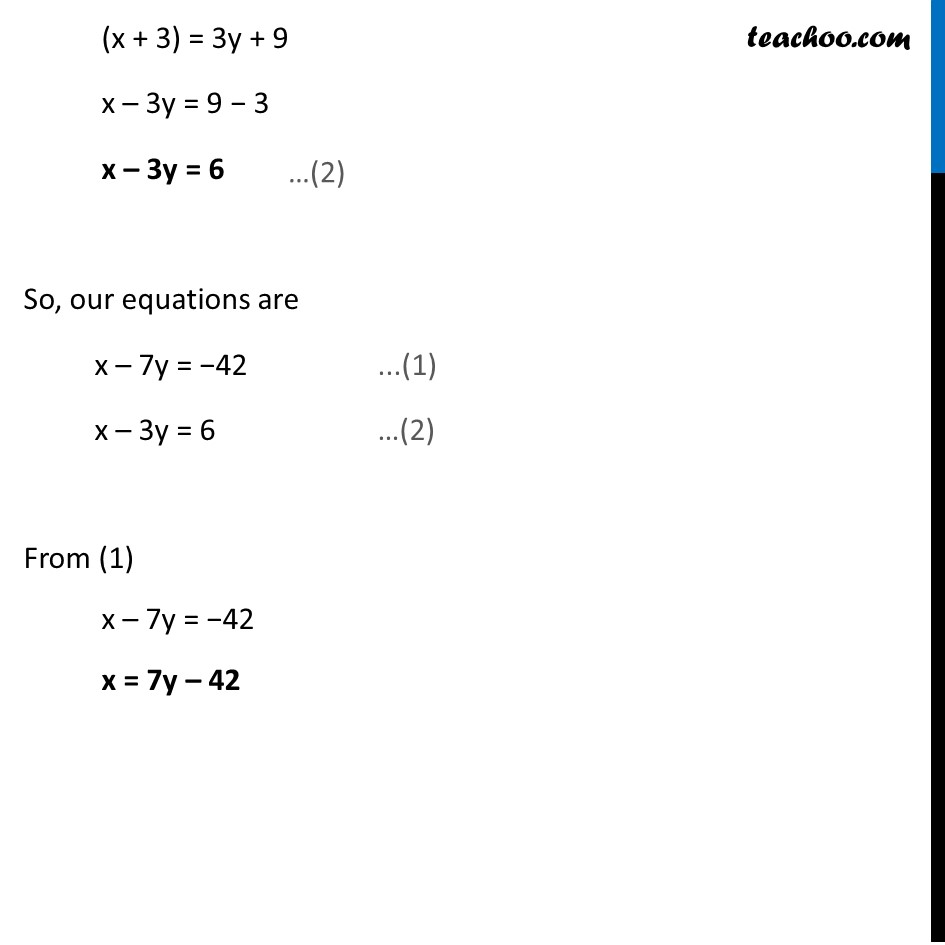
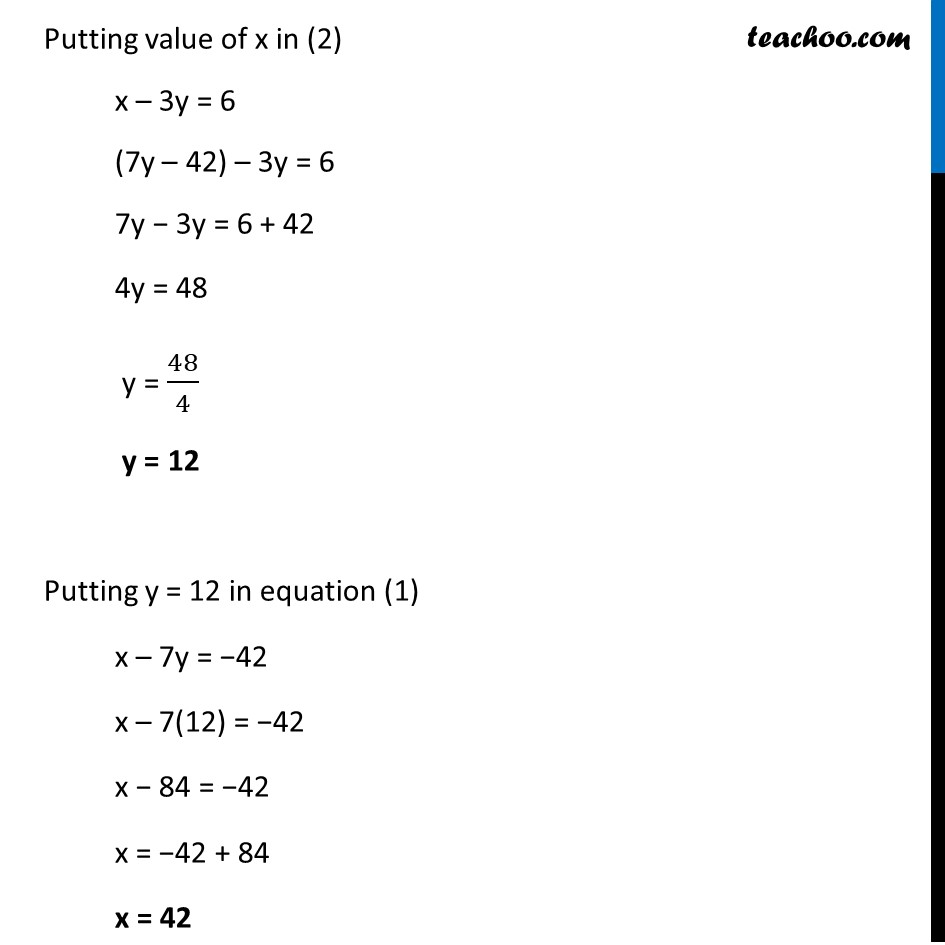
Example 8 Aftab tells his daughter, “Seven years ago, I was seven times as old as you were then. Also, three years from now, I shall be three times as old as you will be.” (Isn’t this interesting?) Solve by the method of substitution. Let the current age of Aftab be x years. & current age of Aftab’s daughter be y years. Seven years ago, Age of Aftab = x – 7 Age of Aftab’s daughter = y – 7 Aftab was seven times as old as Aftab’s daughter (x – 7) = 7(y – 7 ) x – 7 = 7y – 49 x – 7 – 7y + 49 = 0  x – 7y + 42 = 0 Three years from now, Age of Aftab = x + 3 Age of Aftab’s daughter = y + 3 Aftab will be three times as old as his daughter (x + 3) = 3(y + 3 ) (x + 3) = 3y + (3 × 3) (x + 3) = 3y + 9 x + 3 – 3y - 9 = 0 x – 3y - 6 = 0 So, our two equations are x – 7y + 42 = 0 …(1) x – 3y – 6 = 0 …(2) From (1) x – 7y + 42 = 0 x = 7y – 42 Putting value of x in (2) x – 3y – 6 = 0 (7y – 42) – 3y – 6 = 0 7y – 42 – 3y – 6 = 0 4y – 48 = 0 4y = 48 y = 48/4 y = 12 Putting y = 12 in equation (1) x – 7y + 42 = 0  x – 7(12) + 42 = 0 x – 84 + 42 = 0 x – 42 = 0 x = 42 So, x = 42, y = 12 is the solution of the equations Hence, Aftab’s age = x = 42 years His daughter’s age = y = 12 years