Question 4 - Chapter 2 Class 10 Polynomials (Important Question)

Last updated at Dec. 13, 2024 by

Ex 2.3, 4 - On dividing x3 - 3x2 + x + 2 by polynomial g(x) - Ex 2.3

Ex 2.3, 4 - Chapter 2 Class 10 Polynomials - Part 2
Ex 2.3, 4 - Chapter 2 Class 10 Polynomials - Part 3

Go Ad-free

Transcript

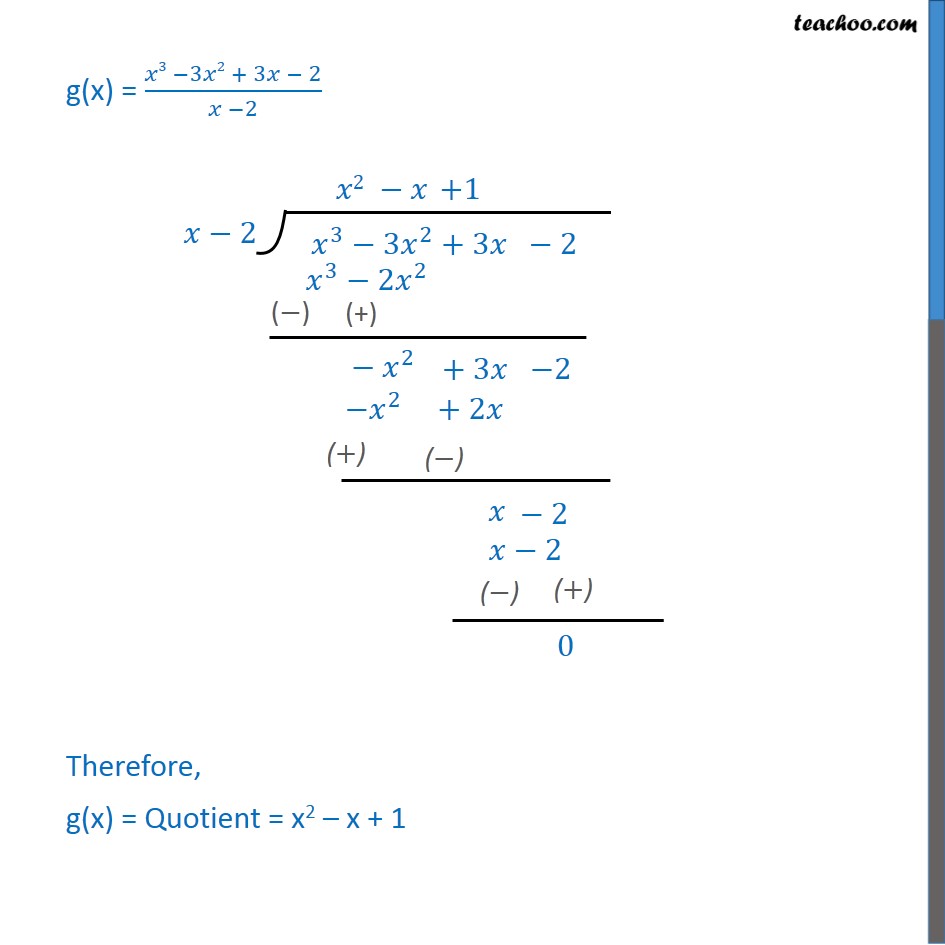
Ex2.3, 4 On dividing x3 3x2 + x + 2 by a polynomial g(x), the quotient and remainder were x 2 and 2x + 4, respectively. Find g(x). Introduction Dividend = Divisor Quotient + Remainder 7 = 3 2 + 1 Ex2.3, 4 On dividing x3 3x2 + x + 2 by a polynomial g(x), the quotient and remainder were x 2 and 2x + 4, respectively. Find g(x). We know that Dividend = Divisor Quotient + Remainder Here, Dividend = x3 3x2 + x + 2 Divisor = g(x) Quotient = (x 2) Remainder = ( 2x + 4) Putting values in (1) x3 3x2 + x + 2 = g(x) (x 2) + (-2x + 4) x3 3x2 + x + 2 + 2x 4 = g(x) (x 2) x3 3x2 + 3x 2 = g(x) (x 2) g(x) = ( 3 3 2 + 3 2)/( 2) Therefore, g(x) = Quotient = x2 x + 1

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo