CBSE Class 10 Sample Paper for 2022 Boards - Maths Standard [MCQ]

CBSE Class 10 Sample Paper for 2022 Boards - Maths Basic [MCQ] →
Class 10
Solutions of Sample Papers for Class 10 Boards

Question 14 - CBSE Class 10 Sample Paper for 2022 Boards - Maths Standard [MCQ] - Solutions of Sample Papers for Class 10 Boards

Last updated at Dec. 16, 2024 by

If the angles of βˆ†ABC are in ratio 1 : 1 : 2, respectively (the largest angle being angle C), then the value of  (sec A)/(cosec B)-tan⁡A/cot⁡B  is :

(a) 0   (b) 1/2  (c) 1   (d) √3  /2

Slide28.JPG

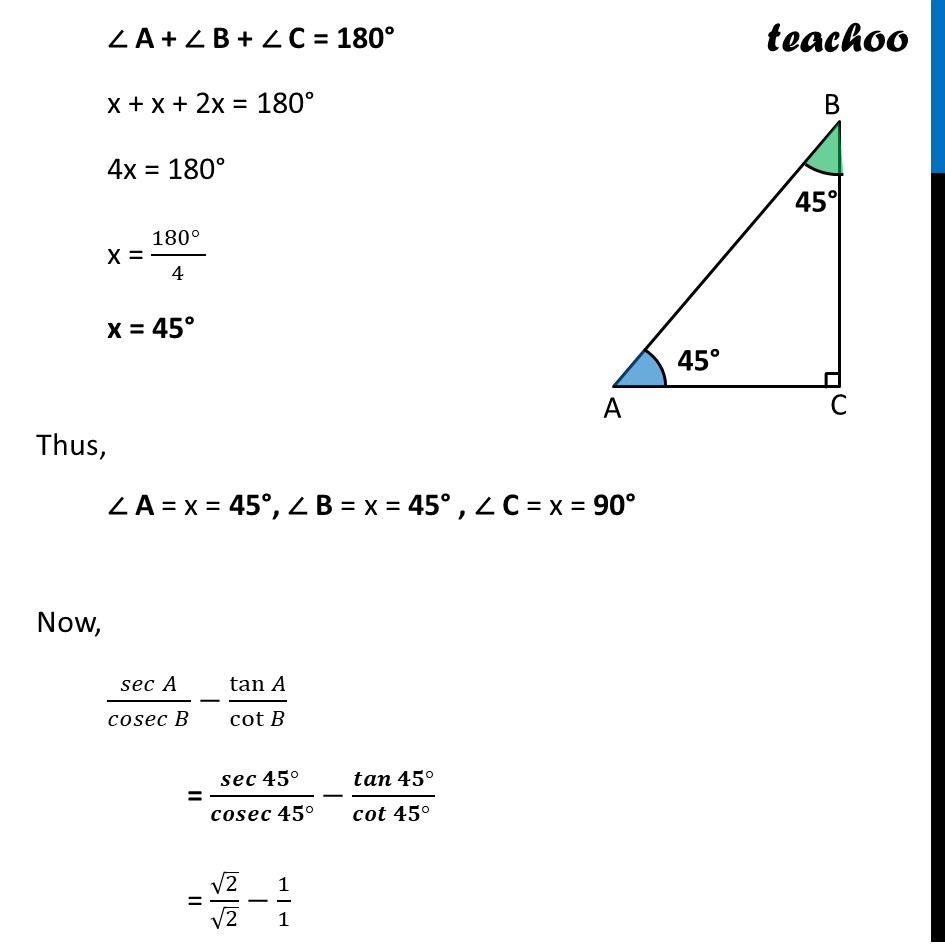
Slide29.JPG
Slide30.JPG

Go Ad-free

Transcript

Question 14 If the angles of βˆ†ABC are in ratio 1 : 1 : 2, respectively (the largest angle being angle C), then the value of (𝑠𝑒𝑐 𝐴)/(π‘π‘œπ‘ π‘’π‘ 𝐡)βˆ’tan⁑𝐴/cot⁑𝐡 is : (a) 0 (b) 1/2 (c) 1 (d) √3 /2 Since angles are in ratio 1 : 1 : 2 Let angles be ∠ A = x ∠ B = x ∠ C = 2x Now, In Ξ” ABC By Angle sum property ∠ A + ∠ B + ∠ C = 180Β° x + x + 2x = 180Β° 4x = 180Β° x = (180Β° )/4 x = 45Β° Thus, ∠ A = x = 45Β°, ∠ B = x = 45Β° , ∠ C = x = 90Β° Now, (𝑠𝑒𝑐 𝐴)/(π‘π‘œπ‘ π‘’π‘ 𝐡)βˆ’tan⁑𝐴/cot⁑𝐡 = (𝒔𝒆𝒄 πŸ’πŸ“Β° )/(𝒄𝒐𝒔𝒆𝒄 πŸ’πŸ“Β°)βˆ’π’•π’‚π’β‘γ€–πŸ’πŸ“Β°γ€—/π’„π’π’•β‘γ€–πŸ’πŸ“Β° γ€— = √2/√2βˆ’1/1 = 1 βˆ’ 1 = 0 So, the correct answer is (a)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo