CBSE Class 12 Sample Paper for 2022 Boards (MCQ Based - for Term 1)

CBSE Class 12 Sample Paper for 2021 Boards →
Class 12
Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Question 43 - CBSE Class 12 Sample Paper for 2022 Boards (MCQ Based - for Term 1) - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Dec. 16, 2024 by

The maximum value of [x (x - 1) + 1 ] (1/3) , 0 ≤ 𝑥 ≤ 1 is:

(a) 0       (b) 1/2         (c) 1     (d) ∛(1/3)

 

This question is inspired from Ex 6.5,29 (MCQ) - Chapter 6 Class 12 - Application of Derivatives

Slide111.JPG

Slide112.JPG
Slide113.JPG

Share on WhatsApp

Transcript

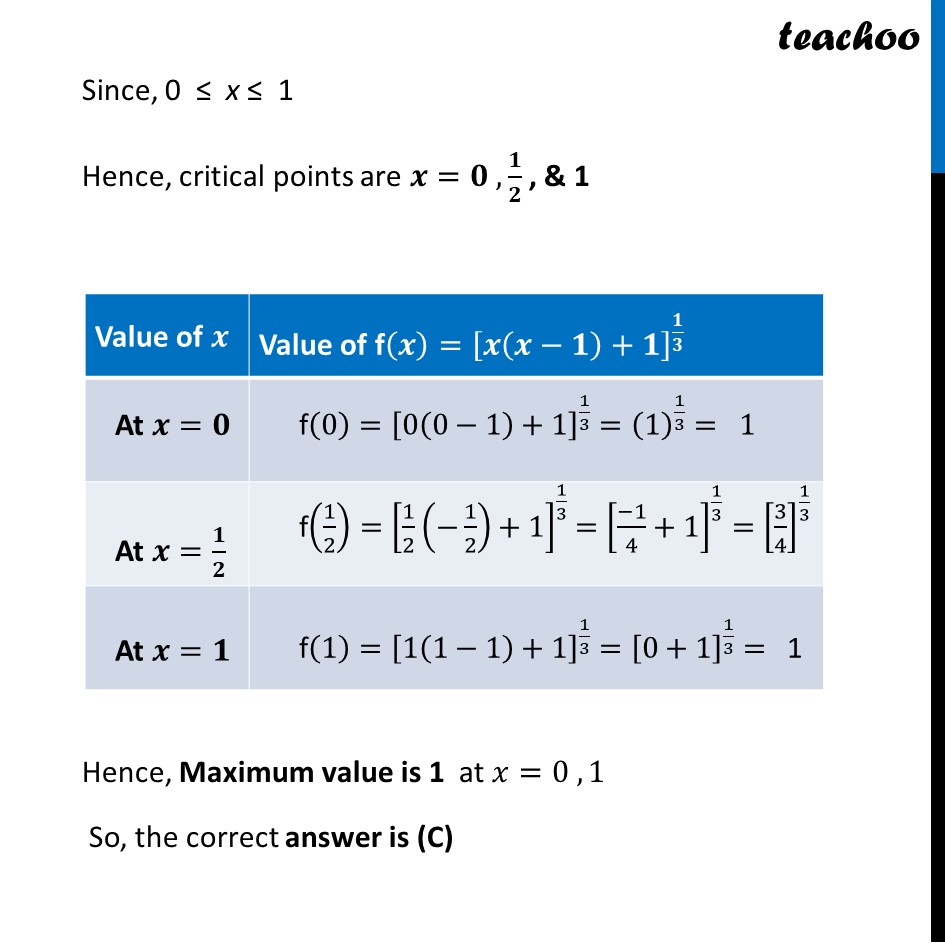
Question 43 The maximum value of ["𝑥 (𝑥 − 1) + 1" ]^(1/3) , 0 ≤ 𝑥 ≤ 1 is: (a) 0 (b) 1/2 (c) 1 (d) ∛(1/3) Let f(𝑥)=[𝑥(𝑥−1)+1]^(1/3) Finding f’(𝒙) 𝑓(𝑥)=[𝑥[𝑥−1]+1]^(1/3) 𝑓(𝑥)=[𝑥^2−𝑥+1]^(1/3) 𝑓^′ (𝑥)=(𝑑(𝑥^2 − 𝑥 + 1)^(1/3))/𝑑𝑥 𝑓^′ (𝑥)=1/3 (𝑥^2−𝑥+1)^(1/3 − 1) . 𝑑(𝑥^2 − 𝑥 + 1)/𝑑𝑥 𝑓^′ (𝑥)=1/3 (𝑥^2−𝑥+1)^((−2)/3) (2𝑥−1) 𝑓^′ (𝑥)=1/(3(𝑥^2 − 𝑥 + 1)^(2/3) ) .(2𝑥−1) 𝑓^′ (𝑥)=(2𝑥 − 1)/(3(𝑥^2 − 𝑥 + 1)^(2/3) ) Putting f’(𝒙)=𝟎 (2𝑥−1)/(3(𝑥^2 − 𝑥 + 1)^(2/3) )=0 2𝑥−1=0 2𝑥=1 𝒙=𝟏/𝟐 Since, 0 ≤ x ≤ 1 Hence, critical points are 𝒙=𝟎 ,𝟏/𝟐 , & 1 Hence, Maximum value is 1 at 𝑥=0 , 1 So, the correct answer is (C)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo