1. Class 12
  2. Solutions of Sample Papers and Past Year Papers - for Class 12 Boards
  3. CBSE Class 12 Sample Paper for 2022 Boards (MCQ Based - for Term 1)

Question 20 - CBSE Class 12 Sample Paper for 2022 Boards (MCQ Based - for Term 1) - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at Sept. 4, 2021 by

The least value of the function 𝑓(π‘₯) = 2 π‘π‘œπ‘  π‘₯ + π‘₯ in the closed interval [0,Β  Ο€/2] is:

(a) 2Β  Β  Β  Β (b) Ο€/2 + √3
(c) Ο€/2Β  Β  (d) The least value does not exist

Slide49.JPG

Slide50.JPG
Slide51.JPG

  1. Class 12
  2. Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

    3. CBSE Class 12 Sample Paper for 2022 Boards (MCQ Based - for Term 1)

    CBSE Class 12 Sample Paper for 2021 BoardsΒ β†’

Transcript

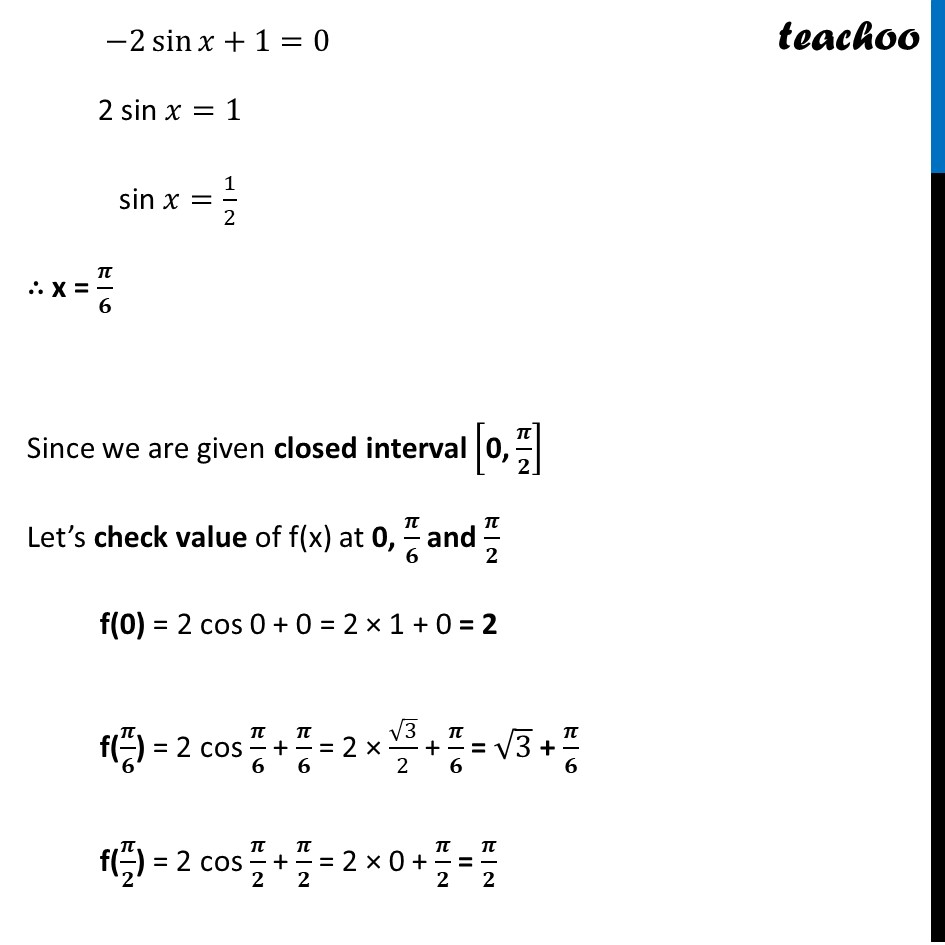
Question 20 The least value of the function 𝑓(π‘₯) = 2 π‘π‘œπ‘  π‘₯ + π‘₯ in the closed interval ["0," πœ‹/2] is: (a) 2 (b) πœ‹/2 + √3 (c) πœ‹/2 (d) The least value does not exist Let f(π‘₯)="2 π‘π‘œπ‘  π‘₯ + π‘₯" Finding f’(𝒙) 𝑓’(π‘₯)=𝑑(2 cos⁑π‘₯ + π‘₯)/𝑑π‘₯ =βˆ’2 sin⁑π‘₯+1 Putting f’(𝒙)=𝟎 βˆ’2 sin⁑π‘₯+1=0 2 sin π‘₯=1 sin π‘₯=1/2 ∴ x = 𝝅/πŸ” Since we are given closed interval ["0," 𝝅/𝟐] Let’s check value of f(x) at 0, 𝝅/πŸ” and 𝝅/𝟐 f(0) = 2 cos 0 + 0 = 2 Γ— 1 + 0 = 2 f(𝝅/πŸ”) = 2 cos 𝝅/πŸ” + 𝝅/πŸ” = 2 Γ— √3/2 + 𝝅/πŸ” = √3 + 𝝅/πŸ” f(𝝅/𝟐) = 2 cos 𝝅/𝟐 + 𝝅/𝟐 = 2 Γ— 0 + 𝝅/𝟐 = 𝝅/𝟐 Since value of f(x) is lowest at x = 𝝅/𝟐 So, the correct answer is (C)

Class 12
Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

About the Author

Davneet Singh's photo - Teacher, Engineer, Marketer
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 10 years. He provides courses for Maths and Science at Teachoo.