Ex 12.2, 4 (iv) - Chapter 12 Class 11 Limits and Derivatives

Transcript

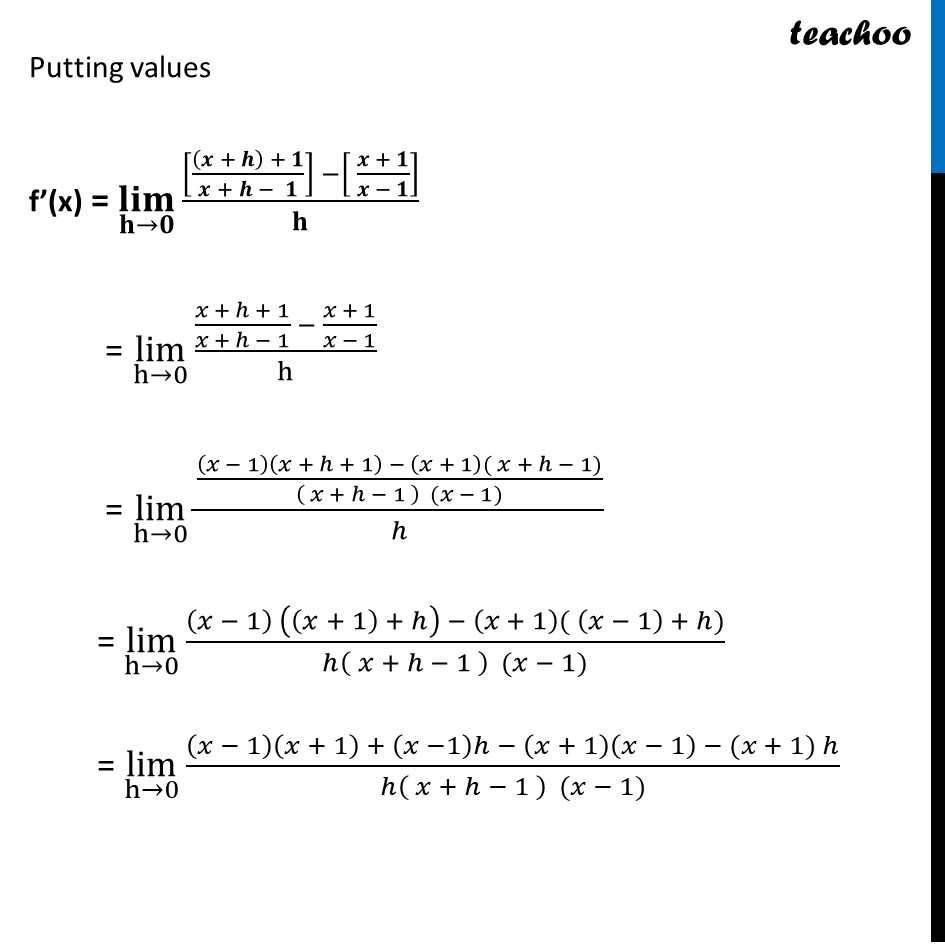
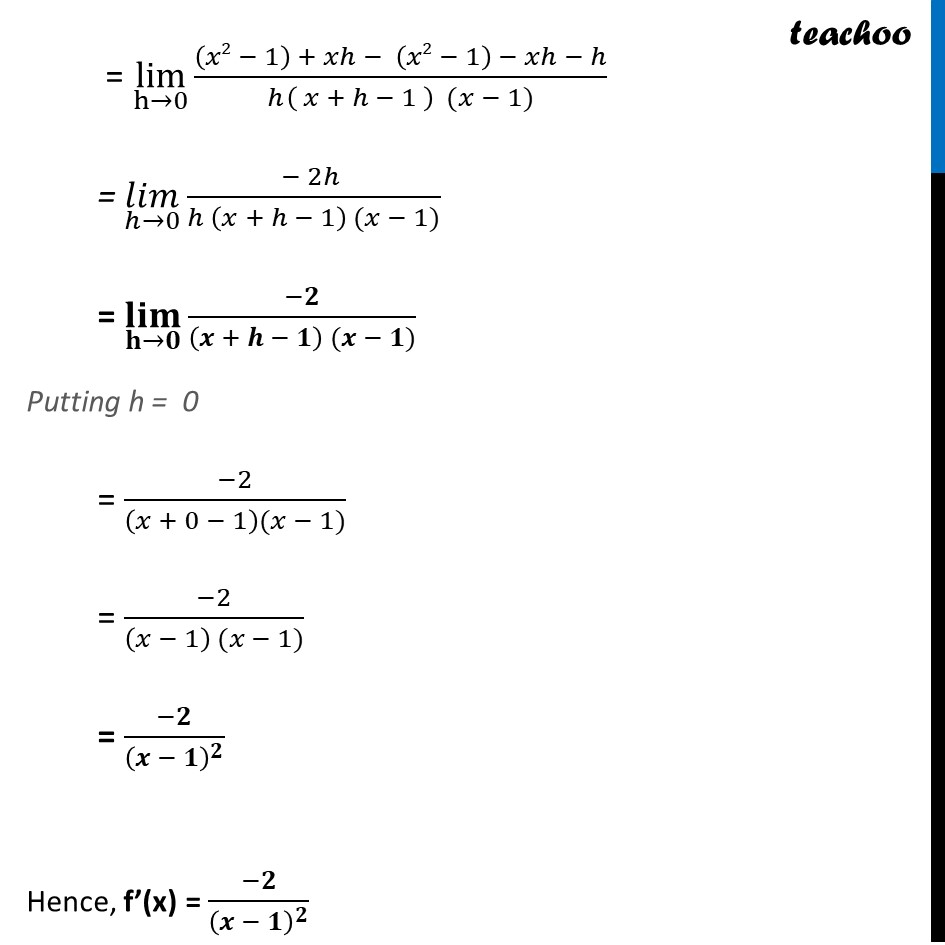
Ex 12.2, 4 Find the derivative of the following functions from first principle. (iv) (𝑥 + 1)/(𝑥 − 1) Let f (x) = (𝑥 + 1)/(𝑥 − 1) We need to find Derivative of f(x) i.e. f’ (x) We know that f’(x) = lim┬(h→0) f⁡〖(x + h) − f(x)〗/h Here, f (x) = (𝑥 + 1)/(𝑥 − 1) So, f (x + h) = ((𝑥 + ℎ) + 1)/((𝑥 + ℎ) − 1) Ex 12.2, 4 Find the derivative of the following functions from first principle. (iv) (𝑥 + 1)/(𝑥 − 1) Let f (x) = (𝑥 + 1)/(𝑥 − 1) We need to find Derivative of f(x) i.e. f’ (x) We know that f’(x) = lim┬(h→0) f⁡〖(x + h) − f(x)〗/h Here, f (x) = (𝑥 + 1)/(𝑥 − 1) So, f (x + h) = ((𝑥 + ℎ) + 1)/((𝑥 + ℎ) − 1) Putting values f’(x) = lim┬(h→0)⁡〖([((𝑥 + ℎ) + 1)/(𝑥 + ℎ − 1)] −[ (𝑥 + 1)/(𝑥 − 1)])/h〗 = lim┬(h→0)⁡〖((𝑥 + ℎ + 1)/(𝑥 + ℎ − 1) − (𝑥 + 1)/(𝑥 − 1))/h〗 = lim┬(h→0)⁡〖(((𝑥 − 1)(𝑥 + ℎ + 1) − (𝑥 + 1)( 𝑥 + ℎ − 1))/(( 𝑥 + ℎ − 1 ) (𝑥 − 1)))/ℎ〗 = lim┬(h→0)⁡〖((𝑥 − 1) ((𝑥 + 1) + ℎ) − (𝑥 + 1)( (𝑥 − 1) + ℎ))/(ℎ( 𝑥 + ℎ − 1 ) (𝑥 − 1))〗 = lim┬(h→0)⁡〖((𝑥 − 1)(𝑥 + 1) + (𝑥 −1)ℎ − (𝑥 + 1)(𝑥 − 1) − (𝑥 + 1) ℎ)/(ℎ( 𝑥 + ℎ − 1 ) (𝑥 − 1))〗 = lim┬(h→0)⁡〖((𝑥2 − 1) + 𝑥ℎ − (𝑥2 − 1) − 𝑥ℎ − ℎ)/(ℎ( 𝑥 + ℎ − 1 ) (𝑥 − 1))〗 = (𝑙𝑖𝑚)┬(ℎ→0)⁡〖(− 2ℎ )/(ℎ (𝑥 + ℎ − 1) (𝑥 − 1))〗 = lim┬(h→0)⁡〖(−2)/((𝑥 + ℎ − 1) (𝑥 − 1))〗 Putting h = 0 = (−2)/((𝑥 + 0 − 1)(𝑥 − 1)) = (−2)/((𝑥 − 1) (𝑥 − 1)) = (−2)/(𝑥 − 1)^2 Hence, f’(x) = (−𝟐)/(𝒙 − 𝟏)^𝟐