Example 6 (iii) - Chapter 7 Class 12 Integrals
Last updated at December 16, 2024 by Teachoo
Examples
Example 1 (i)
You are here
You are here
Chapter 7 Class 12 Integrals
Serial order wise
Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 5 questions, selected from your answers, mistakes, and progress.
Transcript
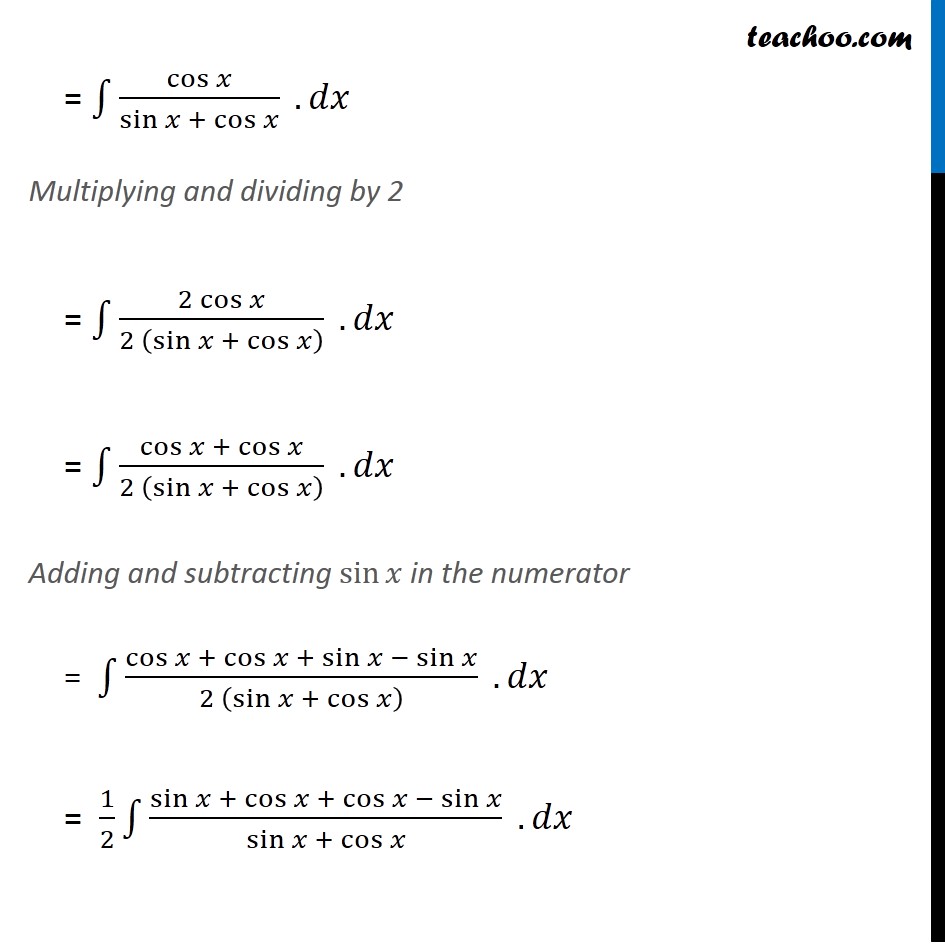
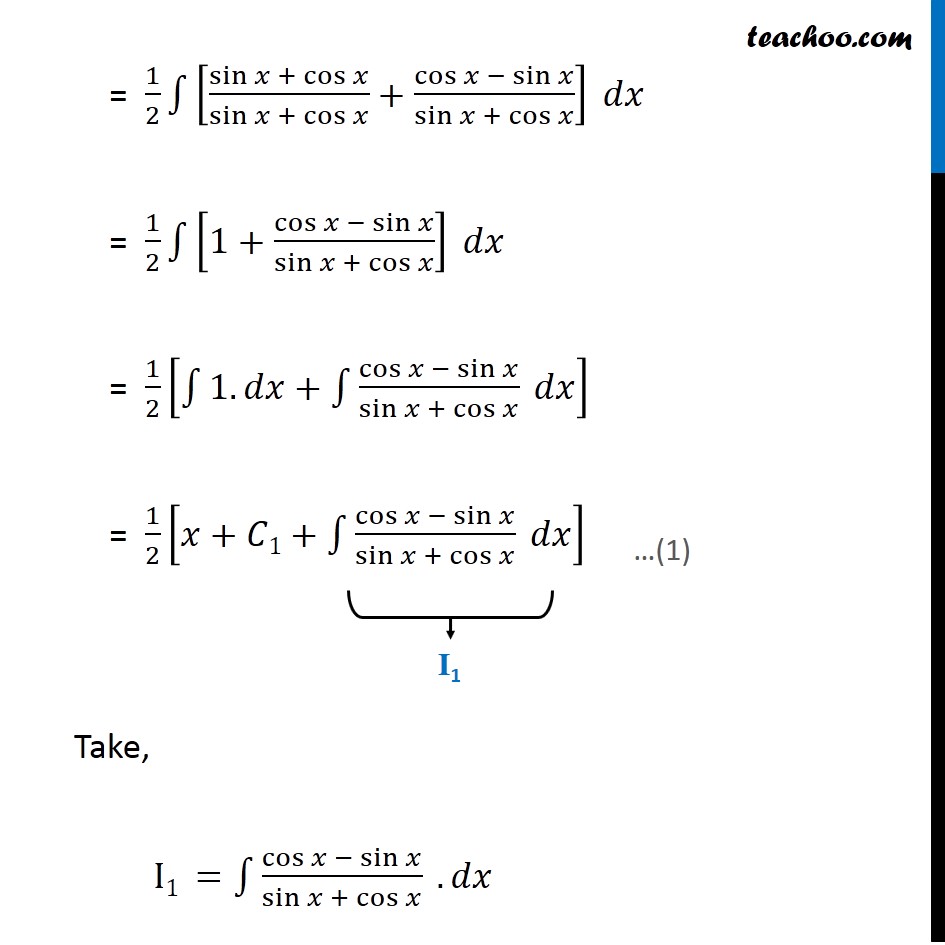
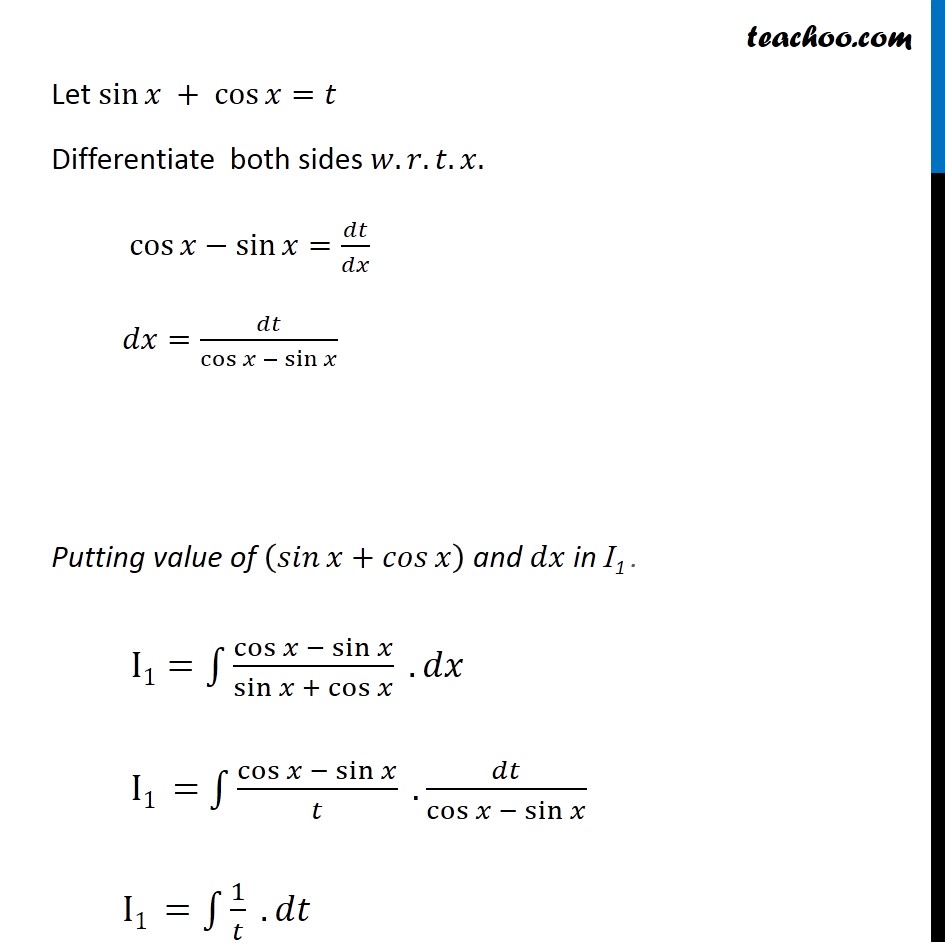
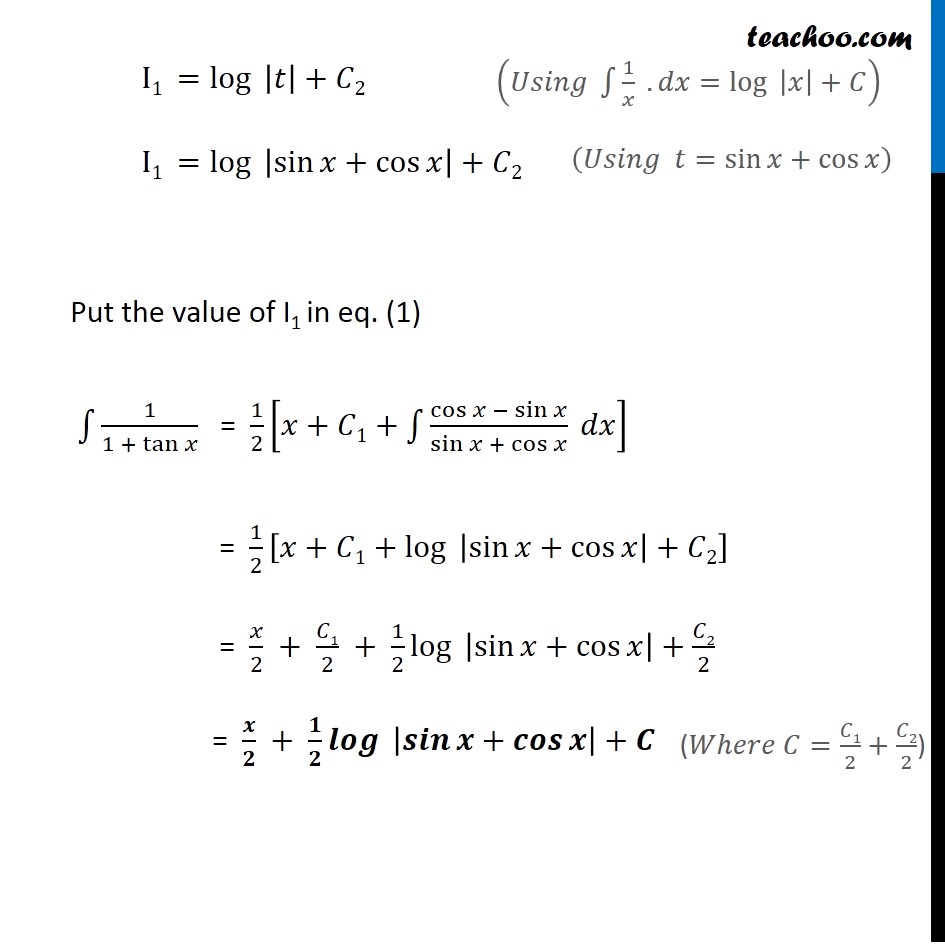
Example 6 Find the following integrals (iii) ∫1▒1/(1 + tan𝑥 ) 𝑑𝑥 The given function cannot be integrated by direct substitution, so we have to simplify the given function . Simplifying the given function and integrating. ∫1▒1/(1 + tan𝑥 ) .𝑑𝑥 = ∫1▒1/(1 + sin𝑥/cos𝑥 ) .𝑑𝑥 = ∫1▒1/((cos𝑥 + sin𝑥)/cos𝑥 ) .𝑑𝑥 (𝑈𝑠𝑖𝑛𝑔 tan𝑥=sin𝑥/cos𝑥 ) = ∫1▒cos𝑥/(sin𝑥 + cos𝑥 ) .𝑑𝑥 Multiplying and dividing by 2 = ∫1▒(2 cos𝑥)/(2 (sin𝑥 + cos𝑥 ) ) .𝑑𝑥 = ∫1▒(cos𝑥 + cos𝑥)/(2 (sin𝑥 + cos𝑥 ) ) .𝑑𝑥 Adding and subtracting sin𝑥 in the numerator = ∫1▒(cos𝑥 + cos𝑥 + sin𝑥 − sin𝑥)/(2 (sin𝑥 + cos𝑥 ) ) .𝑑𝑥 = 1/2 ∫1▒(sin𝑥 + cos𝑥 + cos𝑥 − sin𝑥)/(sin𝑥 + cos𝑥 ) .𝑑𝑥 = 1/2 ∫1▒[(sin𝑥 + cos𝑥)/(sin𝑥 + cos𝑥 )+〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 )] 𝑑𝑥 = 1/2 ∫1▒[1+〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 )] 𝑑𝑥 = 1/2 [∫1▒〖1.𝑑𝑥+∫1▒〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 )〗 𝑑𝑥] = 1/2 [𝑥+𝐶1+∫1▒〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 ) 𝑑𝑥] Take, I1 =∫1▒〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 ) .𝑑𝑥 Let sin𝑥 + cos𝑥=𝑡 Differentiate both sides 𝑤.𝑟.𝑡.𝑥. cos𝑥−sin𝑥=𝑑𝑡/𝑑𝑥 𝑑𝑥=𝑑𝑡/(cos𝑥 − sin𝑥 ) Putting value of (𝑠𝑖𝑛𝑥+𝑐𝑜𝑠𝑥 ) and 𝑑𝑥 in I1 . I1=∫1▒〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 ) .𝑑𝑥 I1 = ∫1▒〖cos𝑥 − sin〗𝑥/𝑡 .𝑑𝑡/(cos𝑥 − sin𝑥 ) I1 =∫1▒1/𝑡 .𝑑𝑡 Let sin𝑥 + cos𝑥=𝑡 Differentiate both sides 𝑤.𝑟.𝑡.𝑥. cos𝑥−sin𝑥=𝑑𝑡/𝑑𝑥 𝑑𝑥=𝑑𝑡/(cos𝑥 − sin𝑥 ) Putting value of (𝑠𝑖𝑛𝑥+𝑐𝑜𝑠𝑥 ) and 𝑑𝑥 in I1 . I1=∫1▒〖cos𝑥 − sin〗𝑥/(sin𝑥 + cos𝑥 ) .𝑑𝑥 I1 = ∫1▒〖cos𝑥 − sin〗𝑥/𝑡 .𝑑𝑡/(cos𝑥 − sin𝑥 ) I1 =∫1▒1/𝑡 .𝑑𝑡 (𝑈𝑠𝑖𝑛𝑔 ∫1▒〖1/𝑥 .〗 𝑑𝑥=log〖 |𝑥|〗+𝐶) (𝑈𝑠𝑖𝑛𝑔 𝑡=sin𝑥+cos𝑥 ) (𝑊ℎ𝑒𝑟𝑒 𝐶=𝐶1/2+𝐶2/2)
