You are here
Given that sin θ = a/b, then cos θ is equal to
(A) b/√(b^2 - a^2 )
(B) b/a
(C) √(b^2 - a^2 )/b
(D) a/√(b^2 - a^2 )
Question 7 - NCERT Exemplar - MCQ - Chapter 8 Class 10 Introduction to Trignometry
Last updated at Dec. 16, 2024 by Teachoo
NCERT Exemplar - MCQ
Chapter 8 Class 10 Introduction to Trignometry
Serial order wise
- Ex 8.1
- Ex 8.2
- Ex 8.3
- Examples
- Case Based Questions (MCQ)
-
NCERT Exemplar - MCQ
- sin 90 - θ, cos 90 - θ formula
Transcript
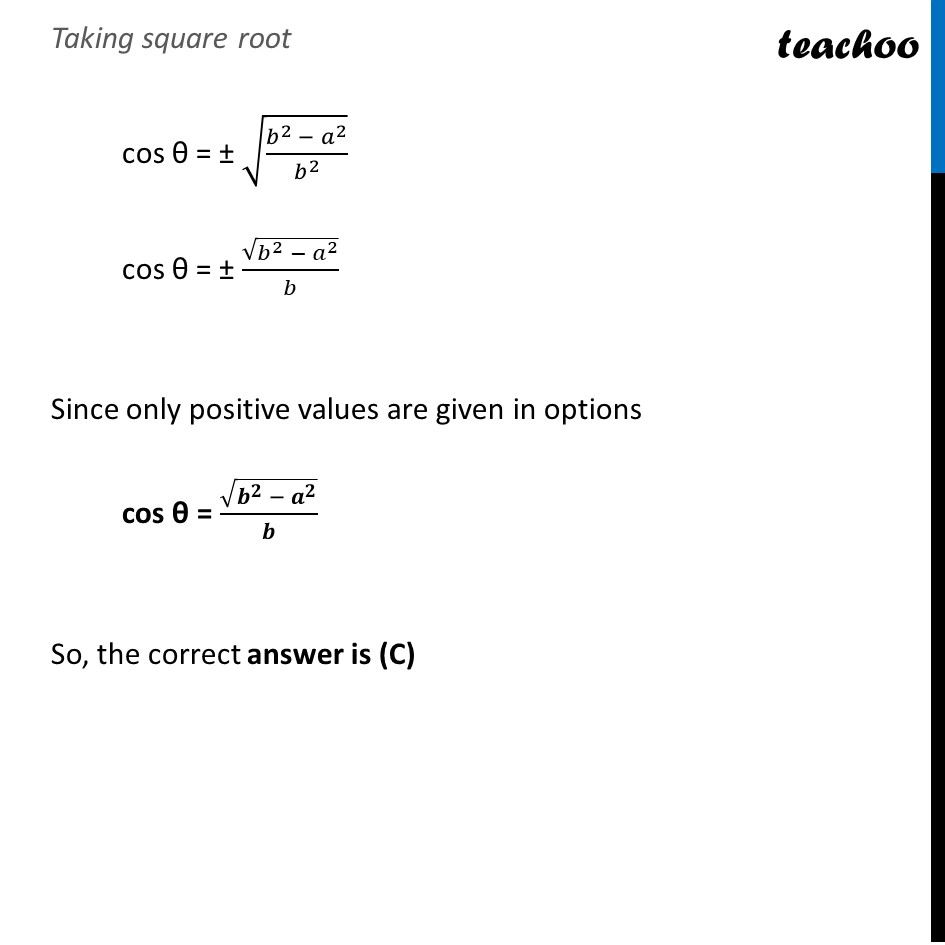
Question 7 Given that sin θ = 𝑎/𝑏, then cos θ is equal to (A) 𝑏/√(𝑏^2 − 𝑎^2 ) (B) 𝑏/𝑎 (C) √(𝑏^2 − 𝑎^2 )/𝑏 (D) 𝑎/√(𝑏^2 − 𝑎^2 ) Now, cos2 θ = 1 − sin2 θ Putting sin θ = 𝑎/𝑏 cos2 θ = 1−(𝑎/𝑏)^2 cos2 θ = 1−𝑎^2/𝑏^2 cos2 θ = (𝒃^𝟐 − 𝒂^𝟐)/𝒃^𝟐 Taking square root cos θ = ± √((𝑏^2 − 𝑎^2)/𝑏^2 ) cos θ = ± √(𝑏^2 − 𝑎^2 )/𝑏 Since only positive values are given in options cos θ = √(𝒃^𝟐 − 𝒂^𝟐 )/𝒃 So, the correct answer is (C)
