Question 13 - NCERT Exemplar - MCQ - Chapter 6 Class 10 Triangles

Last updated at August 14, 2026 by

If ∆ ABC ~ ∆ QRP,  (ar (ABC))/(ar (PQR))=9/4 , AB = 18 cm and BC = 15 cm, then PR is equal to

(A) 10 cm 

(B) 12 cm 

(C) 20/3  cm   

(D) 8 cm

NCERT Exemplar - If ∆ ABC ~ ∆ QRP,  ar (ABC)/ ar (PQR) = 9/4 [MCQ] - NCERT Exemplar - MCQ

part 2 - Question 13 - NCERT Exemplar - MCQ - Serial order wise - Chapter 6 Class 10 Triangles

Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 3 questions, selected from your answers, mistakes, and progress.
Remove Ads
Teachoo · Class 10 Explore Class 10

Transcript

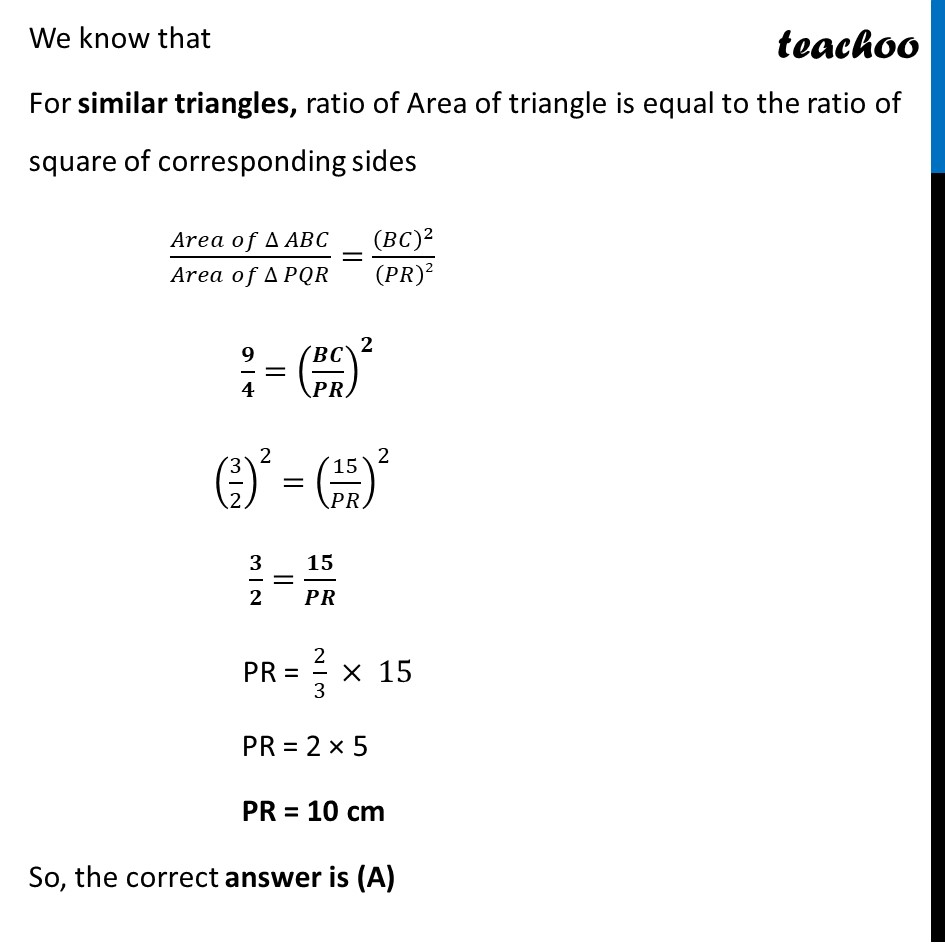
Question 13 If ∆ ABC ~ ∆ QRP, (ar (ABC))/(ar (PQR))=9/4 , AB = 18 cm and BC = 15 cm, then PR is equal to (A) 10 cm (B) 12 cm (C) 20/3 cm (D) 8 cm Our triangles look like We know that For similar triangles, ratio of Area of triangle is equal to the ratio of square of corresponding sides (𝐴𝑟𝑒𝑎 𝑜𝑓 ∆ 𝐴𝐵𝐶)/(𝐴𝑟𝑒𝑎 𝑜𝑓 ∆ 𝑃𝑄𝑅)=(𝐵𝐶)^2/(𝑃𝑅)2 𝟗/𝟒=(𝑩𝑪/𝑷𝑹)^𝟐 (3/2)^2=(15/𝑃𝑅)^2 𝟑/𝟐=𝟏𝟓/𝑷𝑹 PR = 2/3 × 15 PR = 2 × 5 PR = 10 cm So, the correct answer is (A)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.