Question 8 - MCQs from NCERT Exemplar - Chapter 3 Class 10 Pair of Linear Equations in Two Variables

Last updated at Dec. 16, 2024 by

For what value of k, do the equations 3x – y + 8 = 0 and 6x – ky = –16 represent coincident lines?

(A) 1/2   (B) − 1/2   (C) 2    (D) −2

Slide14.JPG

Slide15.JPG

Go Ad-free

Transcript

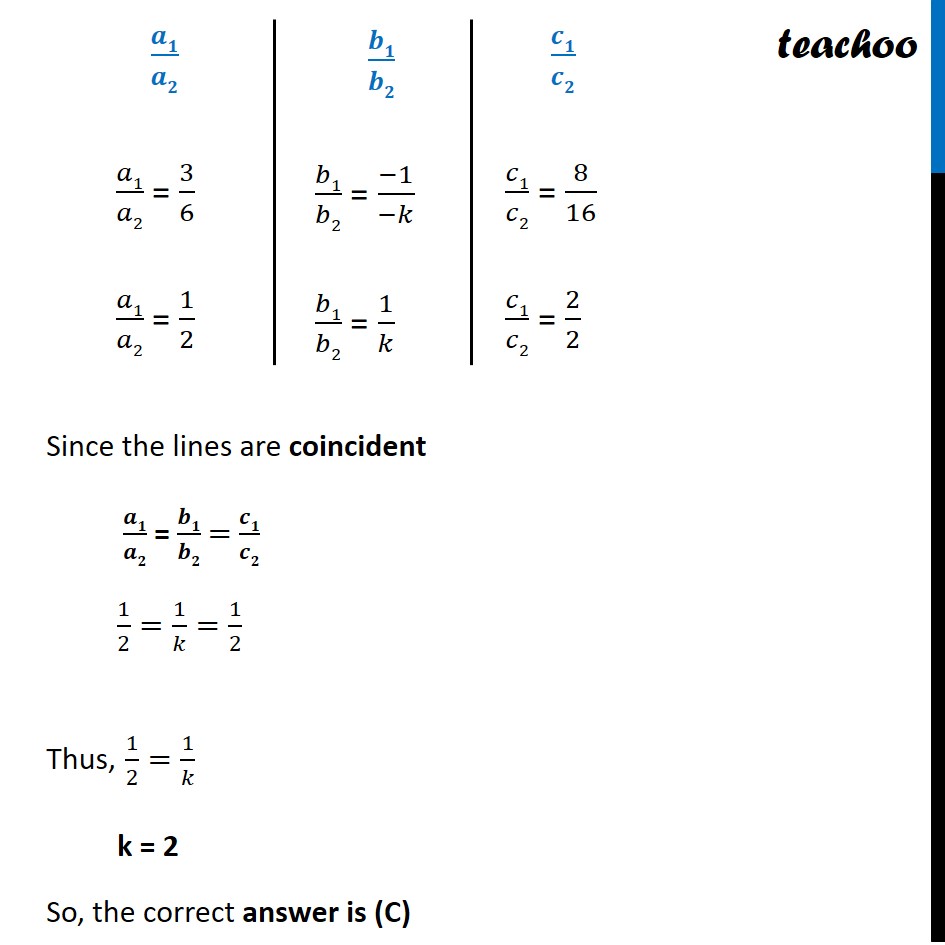
Question 8 For what value of k, do the equations 3x – y + 8 = 0 and 6x – ky = –16 represent coincident lines? (A) 1/2 (B) − 1/2 (C) 2 (D) −2 3x − y + 8 = 0 Comparing with a1x + b1y + c1 = 0 ∴ a1 = 3 , b1 = −1 , c1 = 8 6x − ky + 16 = 0 Comparing with a2x + b2y + c2 = 0 ∴ a2 = 6 , b2 = −k, c2 = 16 Therefore, a1 = 3 , b1 = −1 , c1 = 8 & a2 = 6 , b2 = −k, c2 = 16 𝑎1/𝑎2 = 3/6 𝑎1/𝑎2 = 1/2 𝑏1/𝑏2 = (−1)/(−𝑘) 𝑏1/𝑏2 = 1/𝑘 𝑐1/𝑐2 = 8/16 𝑐1/𝑐2 = 2/2 Since the lines are coincident 𝒂𝟏/𝒂𝟐 = 𝒃𝟏/𝒃𝟐 = 𝒄𝟏/𝒄𝟐 1/2=1/𝑘=1/2 Thus, 1/2=1/𝑘 k = 2 So, the correct answer is (C)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo