Question 3 - MCQs from NCERT Exemplar - Chapter 3 Class 10 Pair of Linear Equations in Two Variables

Last updated at August 12, 2026 by

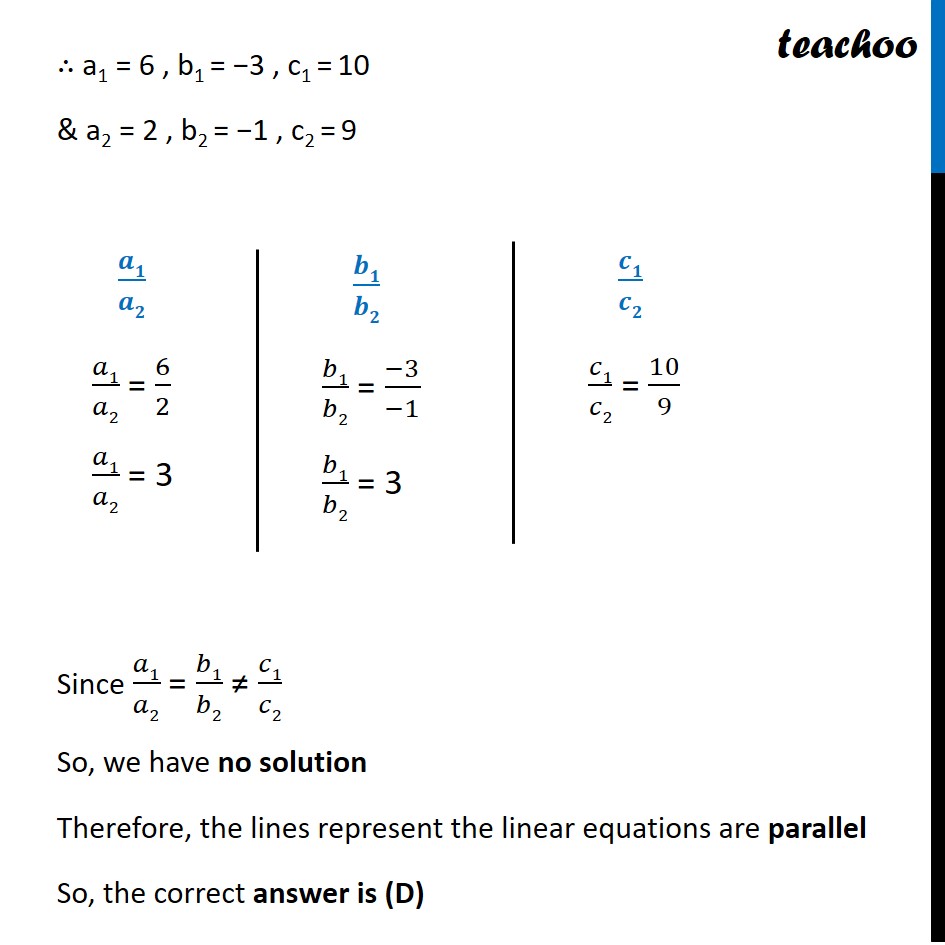
Graphically, the pair of equations 

6x – 3y + 10 = 0 

2x – y + 9 = 0  represents two lines which are 

(a)intersecting at exactly one point 

(b) intersecting at exactly two points 

(c) coincident 

(d) parallel

Graphically, the pair of equations - 6x – 3y + 10 = 0, 2x – y + 9 = 0 - MCQs from NCERT Exemplar

part 2 - Question 3 - MCQs from NCERT Exemplar - Serial order wise - Chapter 3 Class 10 Pair of Linear Equations in Two Variables

Take a fresh quiz. Then take another.
Every attempt is a new AI-adaptive Teachoo quiz with 3 questions, selected from your answers, mistakes, and progress.
Remove Ads
Teachoo · Class 10 Explore Class 10

Transcript

Question 3 Graphically, the pair of equations 6x – 3y + 10 = 0, 2x – y + 9 = 0 represents two lines which are intersecting at exactly one point (b) intersecting at exactly two points (c) coincident (d) parallel 6x – 3y + 10 = 0 Comparing with a1x + b1y + c1 = 0 ∴ a1 = 6 , b1 = −3 , c1 = 10 2x − y + 9 = 0 Comparing with a2x + b2y + c2 = 0 ∴ a2 = 2 , b2 = −1 , c2 = 9 ∴ a1 = 6 , b1 = −3 , c1 = 10 & a2 = 2 , b2 = −1 , c2 = 9 Since 𝑎1/𝑎2 = 𝑏1/𝑏2 ≠ 𝑐1/𝑐2 So, we have no solution Therefore, the lines represent the linear equations are parallel So, the correct answer is (D) 𝑎1/𝑎2 = 6/2 𝑎1/𝑎2 = 3 𝑏1/𝑏2 = (−3)/(−1) 𝑏1/𝑏2 = 3 𝑐1/𝑐2 = 10/9

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.