Question 2 - MCQs from NCERT Exemplar - Chapter 3 Class 10 Pair of Linear Equations in Two Variables

Last updated at April 16, 2024 by

The sum of the digits of a two-digit number is 9. If 27 is added

to it, the digits of the number get reversed. The number is

(A) 25          (B) 72       (C) 63         (D) 36

Slide3.JPG

Slide4.JPG
Slide5.JPG
Slide6.JPG

Transcript

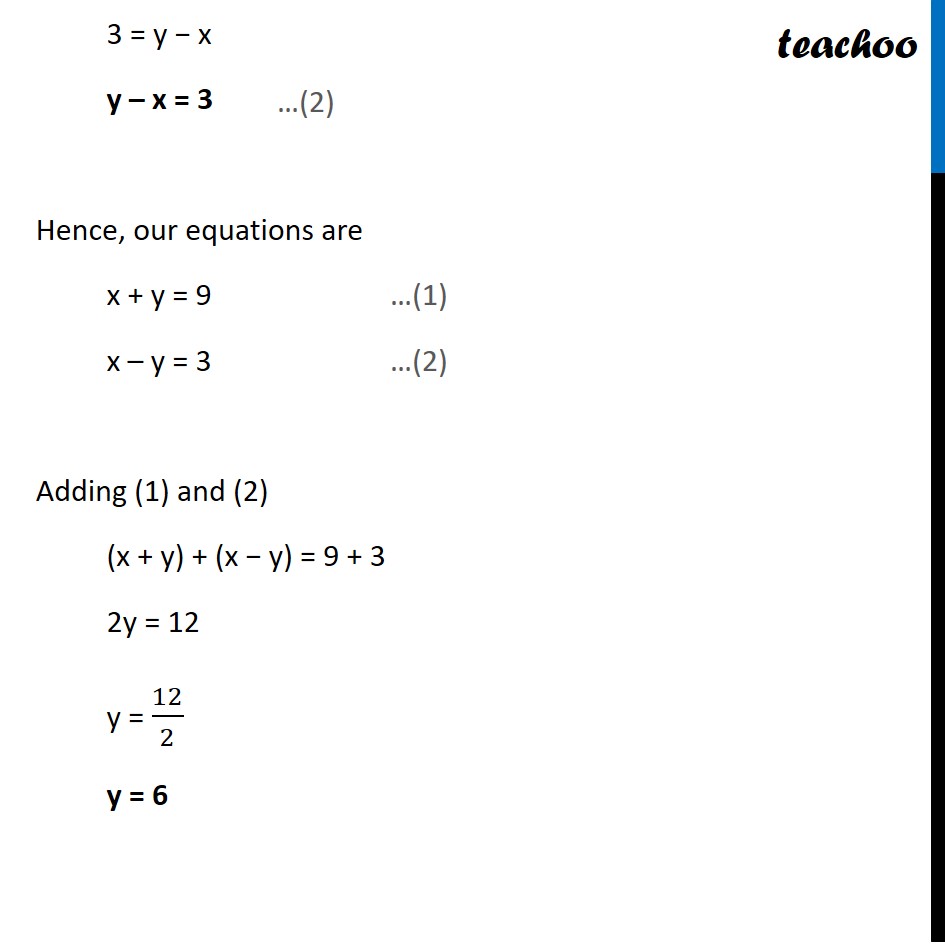
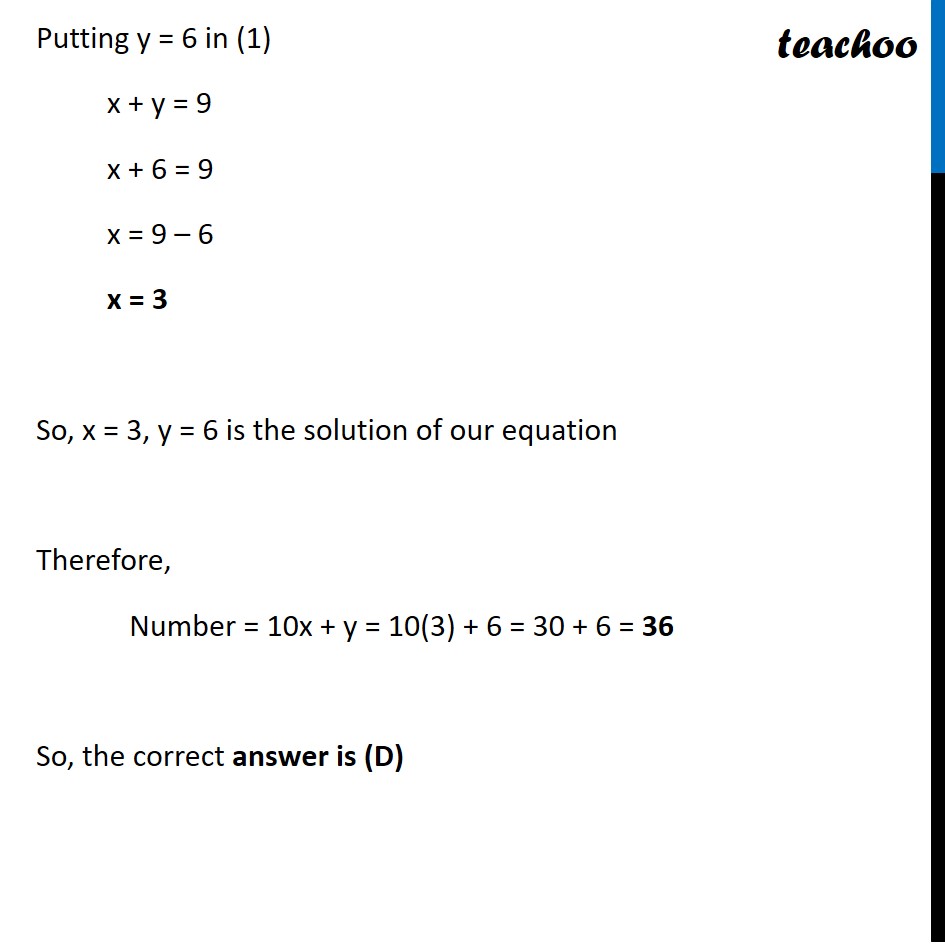
Question 2 The sum of the digits of a two-digit number is 9. If 27 is added to it, the digits of the number get reversed. The number is (A) 25 (B) 72 (C) 63 (D) 36 Number is of the form Let Digit at Units place = y & Digit at Tens place = x Given that Sum of digits of two digit number is 9 ∴ Digit at tens place + Digit at units place = 9 x + y = 9 Also, If 27 is added to number, digits get reversed 27 + Number = Reversed number 27 + 10x + y = 10y + x 27 = 10y + x − 10x − y 27 = 9y − 9x 27 = 9(y − x) 27/9 = y − x 3 = y − x y – x = 3 Hence, our equations are x + y = 9 …(1) x – y = 3 …(2) Adding (1) and (2) (x + y) + (x − y) = 9 + 3 2y = 12 y = 12/2 y = 6 Putting y = 6 in (1) x + y = 9 x + 6 = 9 x = 9 – 6 x = 3 So, x = 3, y = 6 is the solution of our equation Therefore, Number = 10x + y = 10(3) + 6 = 30 + 6 = 36 So, the correct answer is (D)

Go Ad-free
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.