Ex 2.2, 1 (iv) - Chapter 2 Class 10 Polynomials

Transcript

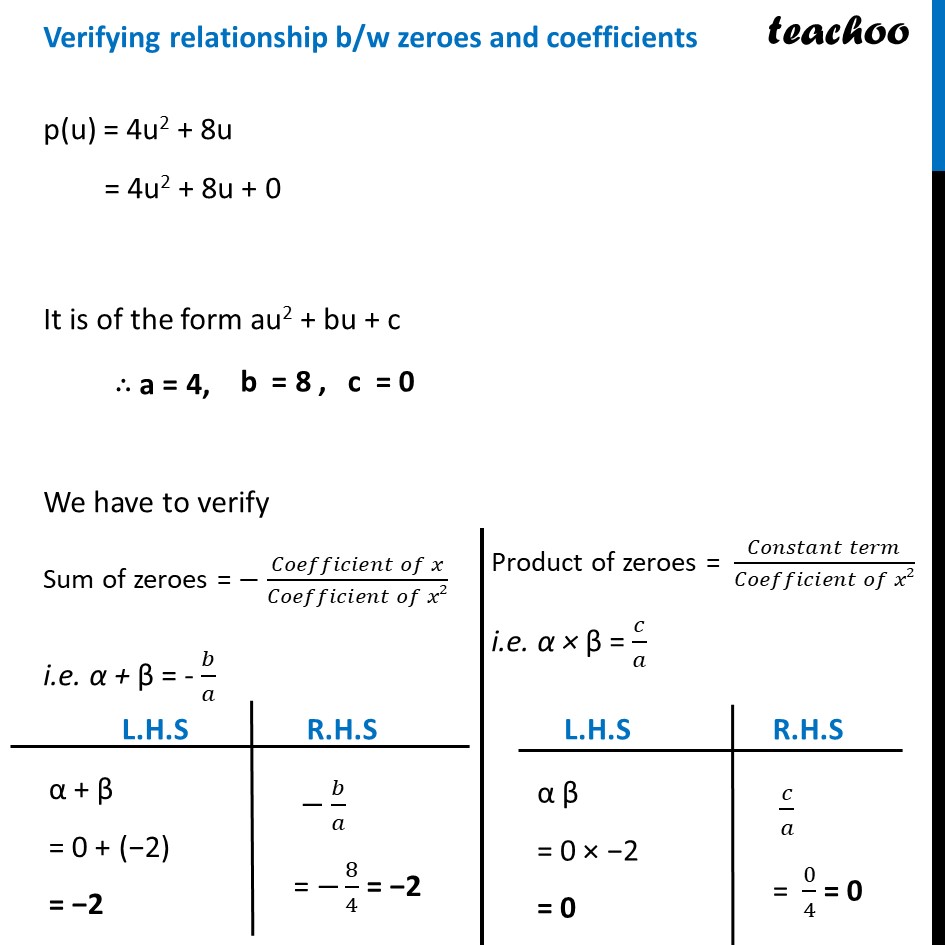
Ex 2.2, 1 Find the zeroes of the following quadratic polynomials and verify the relationship between the zeroes and the coefficients. (iv) 4u2 + 8u Let p(u) = 4u2 + 8u Zero of the polynomial is the value of u where p(u) = 0 Putting p(u) = 0 4u2 + 8u = 0 4u (u + 2) = 0 u (u + 2) = 0/4 u (u + 2) = 0 So, u = 0, –2 Verifying relationship b/w zeroes and coefficients p(u) = 4u2 + 8u = 4u2 + 8u + 0 It is of the form au2 + bu + c ∴ a = 4, We have to verify Sum of zeroes = − (𝐶𝑜𝑒𝑓𝑓𝑖𝑐𝑖𝑒𝑛𝑡 𝑜𝑓 𝑥)/(𝐶𝑜𝑒𝑓𝑓𝑖𝑐𝑖𝑒𝑛𝑡 𝑜𝑓 𝑥2) i.e. α + β = - 𝑏/𝑎 α + β = 0 + (−2) = −2 − 𝑏/𝑎 = −8/4 = −2 α β = 0 × −2 = 0 𝑐/𝑎 = 0/4 = 0 Since, L.H.S = R.H.S Hence relationship between zeroes & coefficient is verified