You are here
Question 15 - Triangles with same base & same parallel lines - Areas of Parallelograms and Triangles
Last updated at Dec. 13, 2024 by Teachoo
Triangles with same base & same parallel lines
Areas of Parallelograms and Triangles
Concept wise
- Introduction
- Paralleograms with same base & same parallel lines
-
Triangles with same base & same parallel lines
- Paralleograms & triangles with same base & same parallel lines
- Median divides triangle into two triangles of equal area
Transcript
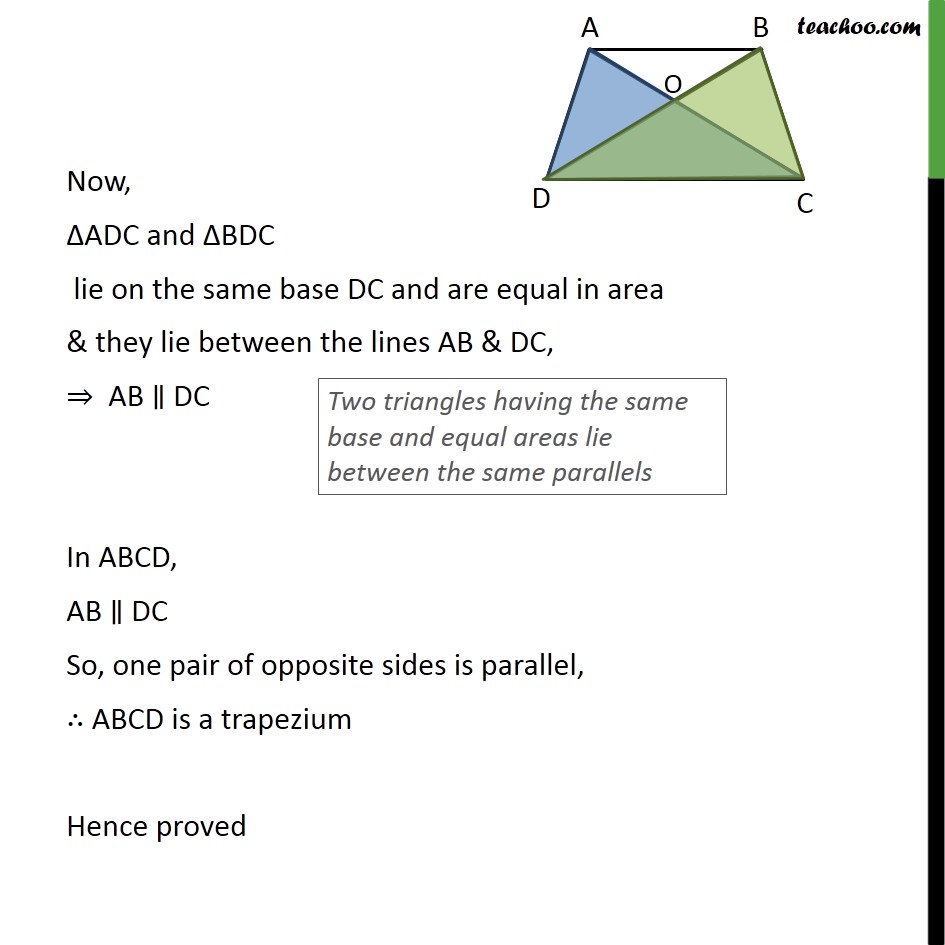
Question 15 Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar(AOD) = ar(BOC). Prove that ABCD is a trapezium . Given: A quadrilateral ABCD where diagonals AC & BD intersect at O & ar(AOD) = ar(BOC) To prove: ABCD is a trapezium Proof : A trapezium is a quadrilateral with one pair of opposite sides parallel Given ar(AOD) = ar(BOC) Adding ar (ODC) on both sides, Ar(AOD) + ar(ODC) = ar(BOC) + ar(ODC) ⇒ ar(ADC) = ar(BDC) Now, ΔADC and ΔBDC lie on the same base DC and are equal in area & they lie between the lines AB & DC, ⇒ AB ∥ DC In ABCD, AB ∥ DC So, one pair of opposite sides is parallel, ∴ ABCD is a trapezium Hence proved
