Ex 4.1, 2 (iv) - Chapter 4 Class 10 Quadratic Equations

Transcript

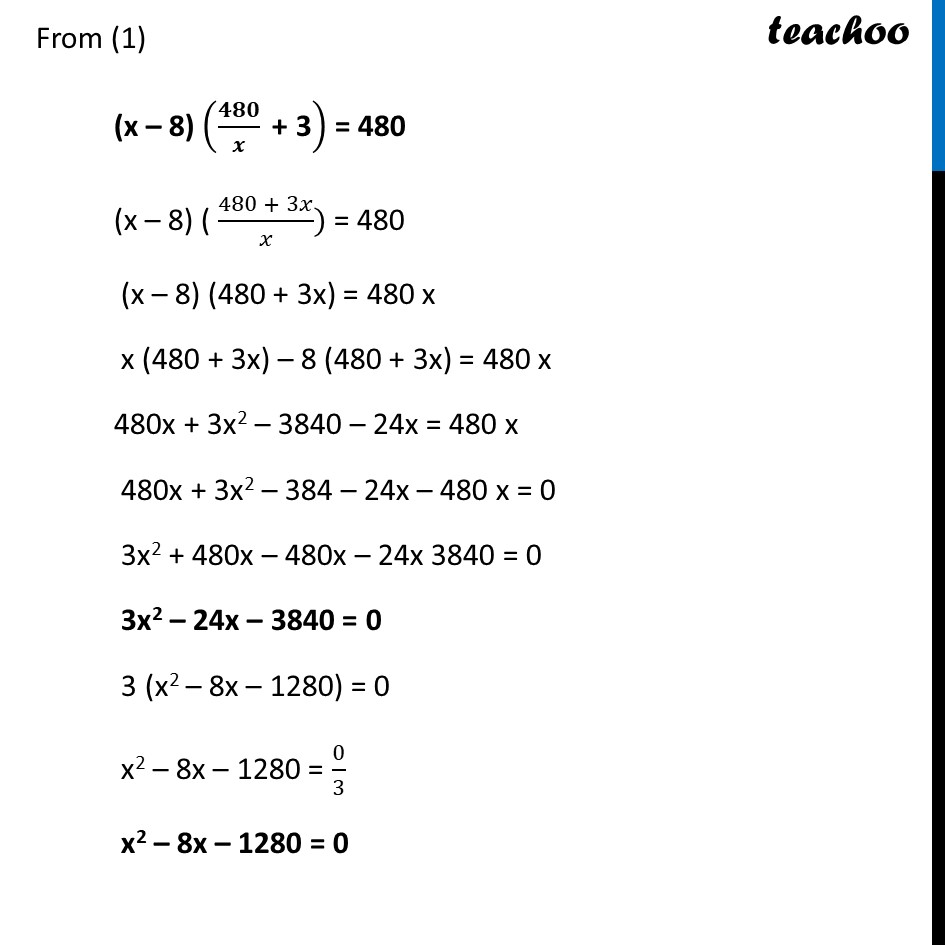
Ex 4.1, 2 Represent the following situations in the form of quadratic equations : (iv) A train travels a distance of 480 km at a uniform speed. If the speed had been 8 km/h less, then it would have taken 3 hours more to cover the same distance. We need to find the speed of the train. Let Speed of train be x km/hr Normal speed Distance = 480 km Speed = x km/hr Speed = 𝐷𝑖𝑠𝑡𝑎𝑛𝑐𝑒/𝑇𝑖𝑚𝑒 x = 480/𝑇𝑖𝑚𝑒 Time = 𝟒𝟖𝟎/𝒙 Speed became 8 km/h less Distance = 480 km Speed = (x – 8) km/hr Time = 480/𝑥 + 3 Speed = 𝐷𝑖𝑠𝑡𝑎𝑛𝑐𝑒/𝑇𝑖𝑚𝑒 x – 8 = 480/((480/𝑥 " + 3" ) ) (x – 8) (𝟒𝟖𝟎/𝒙 " + 3" ) = 480 From (1) (x – 8) (𝟒𝟖𝟎/𝒙 " + 3" ) = 480 (x – 8) ( (480 + 3𝑥)/𝑥) = 480 (x – 8) (480 + 3x) = 480 x x (480 + 3x) – 8 (480 + 3x) = 480 x 480x + 3x2 – 3840 – 24x = 480 x 480x + 3x2 – 384 – 24x – 480 x = 0 3x2 + 480x – 480x – 24x 3840 = 0 3x2 – 24x – 3840 = 0 3 (x2 – 8x – 1280) = 0 x2 – 8x – 1280 = 0/3 x2 – 8x – 1280 = 0 It is the form of ax2 + bx + c = 0 Where a = 1, b = – 8 , c = –1280 Hence, it is a quadratic equation .