Question 5 - Paralleograms with same base & same parallel lines - Areas of Parallelograms and Triangles

Transcript

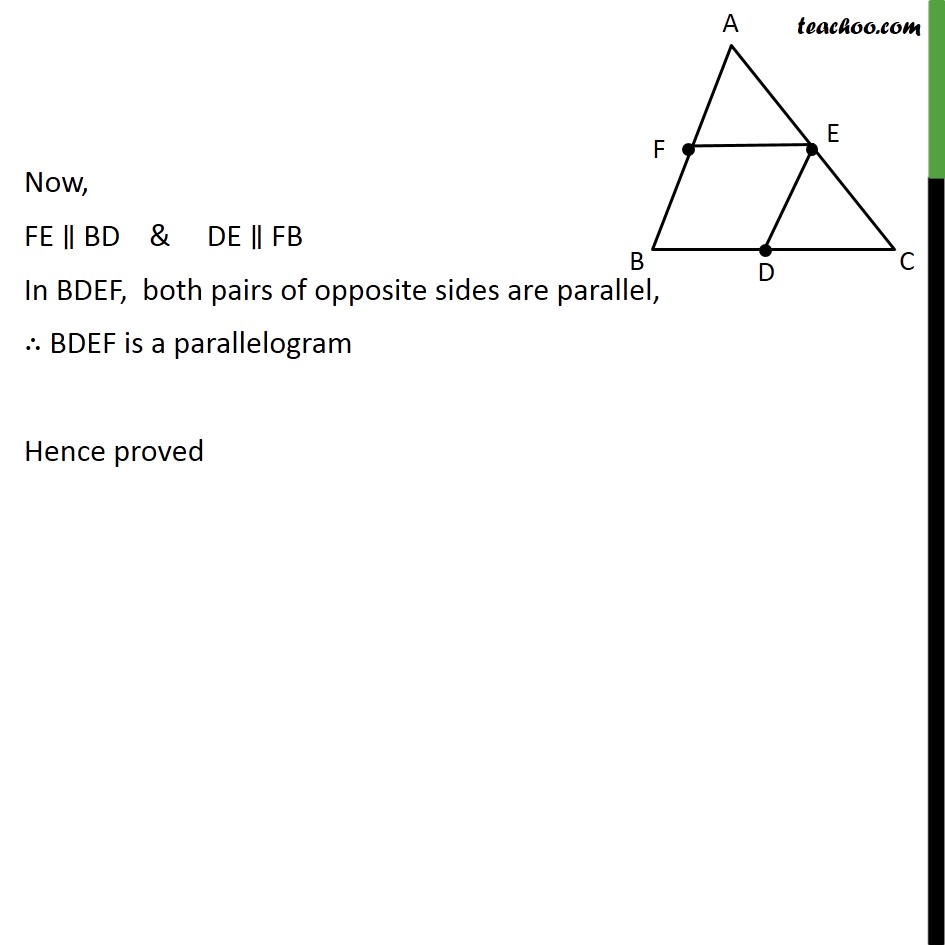
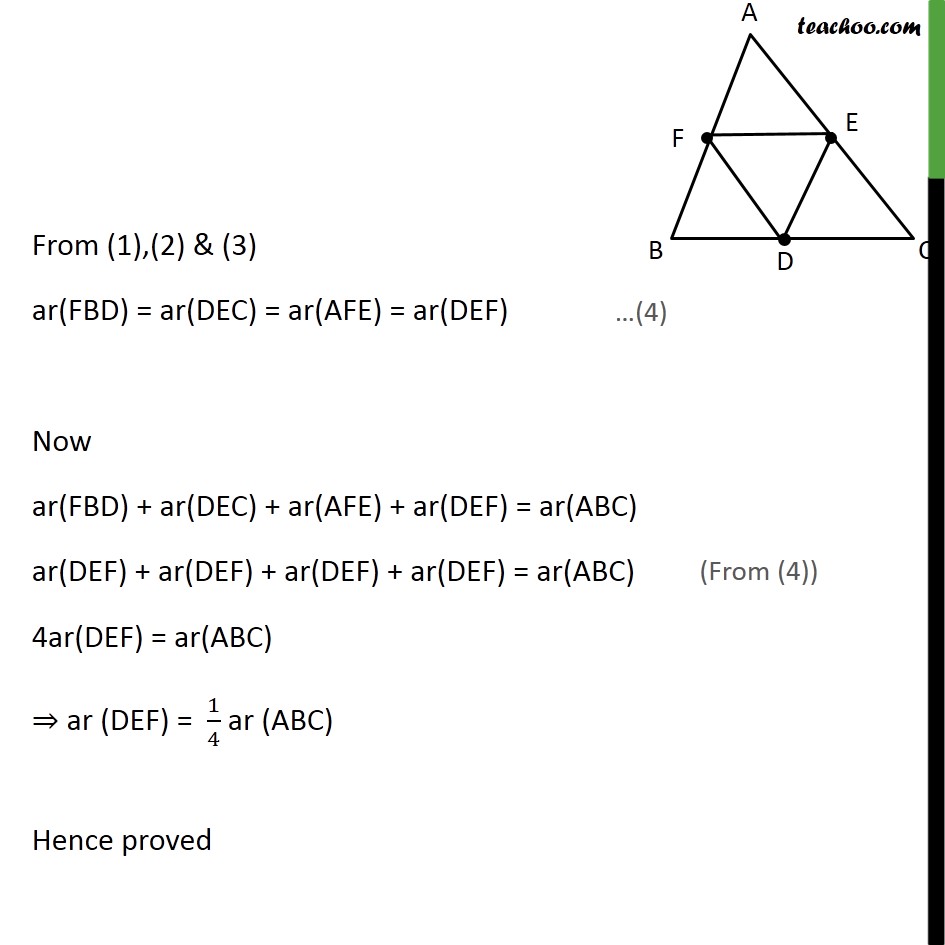
Question 5 D, E and F are respectively the mid-points of the sides BC, CA and AB of a ABC. Show that (i) BDEF is a parallelogram. Given: ABC where D, E, F are mid-points of BC, AC & AB respectively To prove: BDEF is a parallelogram Proof : In ABC, F is mid-point of AB, E is mid-point of AC FE BC FE BD Now, FE BD & DE FB In BDEF, both pairs of opposite sides are parallel, BDEF is a parallelogram Hence proved Question 5 D, E and F are respectively the mid-points of the sides BC, CA and AB of a ABC. Show that (ii) ar (DEF) = 1/4 ar (ABC) In part(i) we proved that BDEF is a parallelogram DBF DEF ar(DBF) = ar(DEF) Similarly, we can prove FDCE is a parallelogram DEC DEF ar(DEC) = ar(DEF) Similarly, we can prove AFDE is a parallelogram AFE DEF ar(AFE) = ar(DEF) From (1),(2) & (3) ar(FBD) = ar(DEC) = ar(AFE) = ar(DEF) Now ar(FBD) + ar(DEC) + ar(AFE) + ar(DEF) = ar(ABC) ar(DEF) + ar(DEF) + ar(DEF) + ar(DEF) = ar(ABC) 4ar(DEF) = ar(ABC) ar (DEF) = 1/4 ar (ABC) Hence proved Question 5 D, E and F are respectively the mid-points of the sides BC, CA and AB of a ABC. Show that (iii) ar (BDEF) = 1/2 ar (ABC) We proved in part(ii) that ar(DEF) = 1/4 ar(ABC) Multiplying both sides by 2 2 ar(DEF) = 2 1/4 ar(ABC) 2 ar(DEF) = 1/2 ar(ABC) ar(DEF) + ar(DEF) = 1/2 ar(ABC) ar(DEF) + ar(FBD) = 1/2 ar(ABC) ar(BDEF) = 1/2 ar(ABC) Hence proved