Question 5 - Chapter 9 Class 9 Areas of parallelograms and Triangles (Important Question)

Last updated at Dec. 13, 2024 by

Ex 9.2, 5 - In figure, PQRS and ABRS are parallelograms - Paralleograms & triangles with same base & same parallel lines

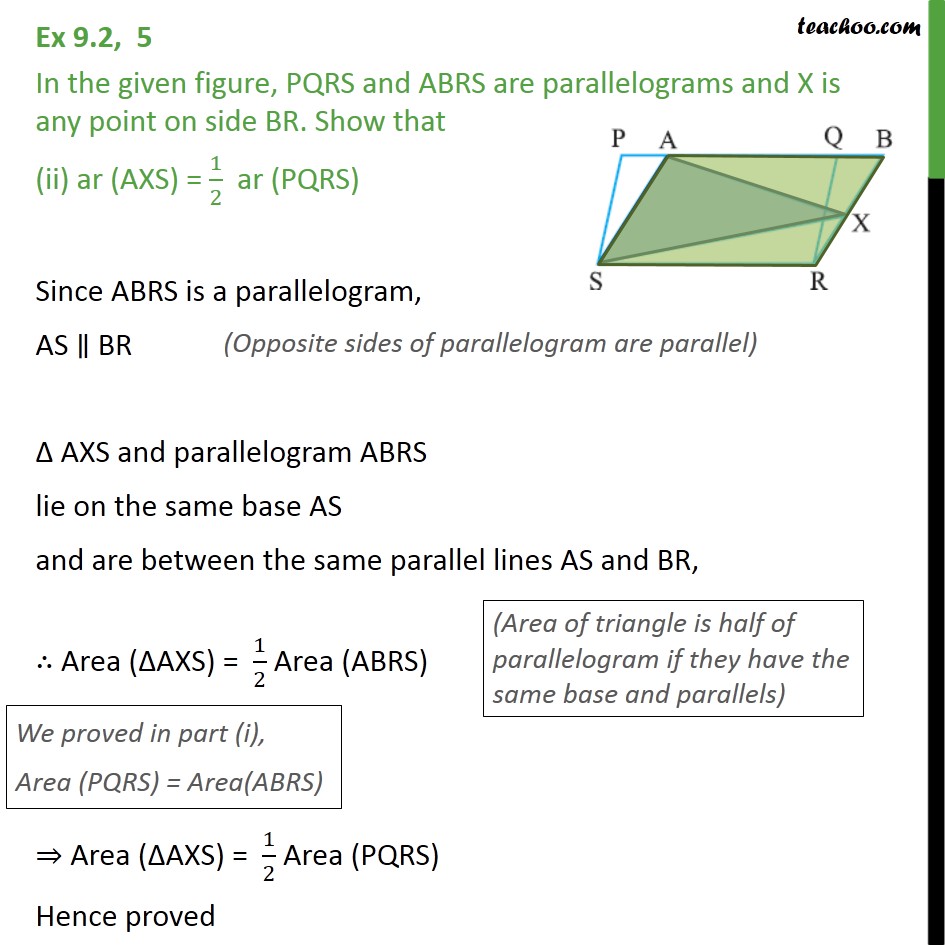
Ex 9.2, 5 - Chapter 9 Class 9 Areas of Parallelograms and Triangles - Part 2

Go Ad-free

Transcript

Question 5 In the given figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that (i) ar (PQRS) = ar (ABRS) Since PQRS is a parallelogram PQ ∥ RS & ABRS is also a parallelogram So, AB ∥ RS Since PQ ∥ RS & AB ∥ RS We can say that PB ∥ RS Now, PQRS & ABRS are two parallelograms with the same base RS and between the same parallels PB & RS ∴ ar (PQRS) = ar (ABRS) Question 5 In the given figure, PQRS and ABRS are parallelograms and X is any point on side BR. Show that (ii) ar (AXS) = 1/2 ar (PQRS) Since ABRS is a parallelogram, AS ∥ BR Δ AXS and parallelogram ABRS lie on the same base AS and are between the same parallel lines AS and BR, ∴ Area (ΔAXS) = 1/2 Area (ABRS) ⇒ Area (ΔAXS) = 1/2 Area (PQRS) Hence proved

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo