CBSE Class 10 Sample Paper for 2021 Boards - Maths Standard

CBSE Class 10 Sample Paper for 2021 Boards - Maths Basic →
Class 10
Solutions of Sample Papers for Class 10 Boards

Question 22 (Choice - 1) - CBSE Class 10 Sample Paper for 2021 Boards - Maths Standard - Solutions of Sample Papers for Class 10 Boards

Last updated at April 16, 2024 by

Find the point on x-axis which is equidistant from the points (2,–2) and (–4,2)

Find point on x-axis which is equidistant from point (2,-2) and (-4,2)

Question 22 (Choice - 1) - CBSE Class 10 Sample Paper for 2021 Boards - Maths Standard - Part 2

Transcript

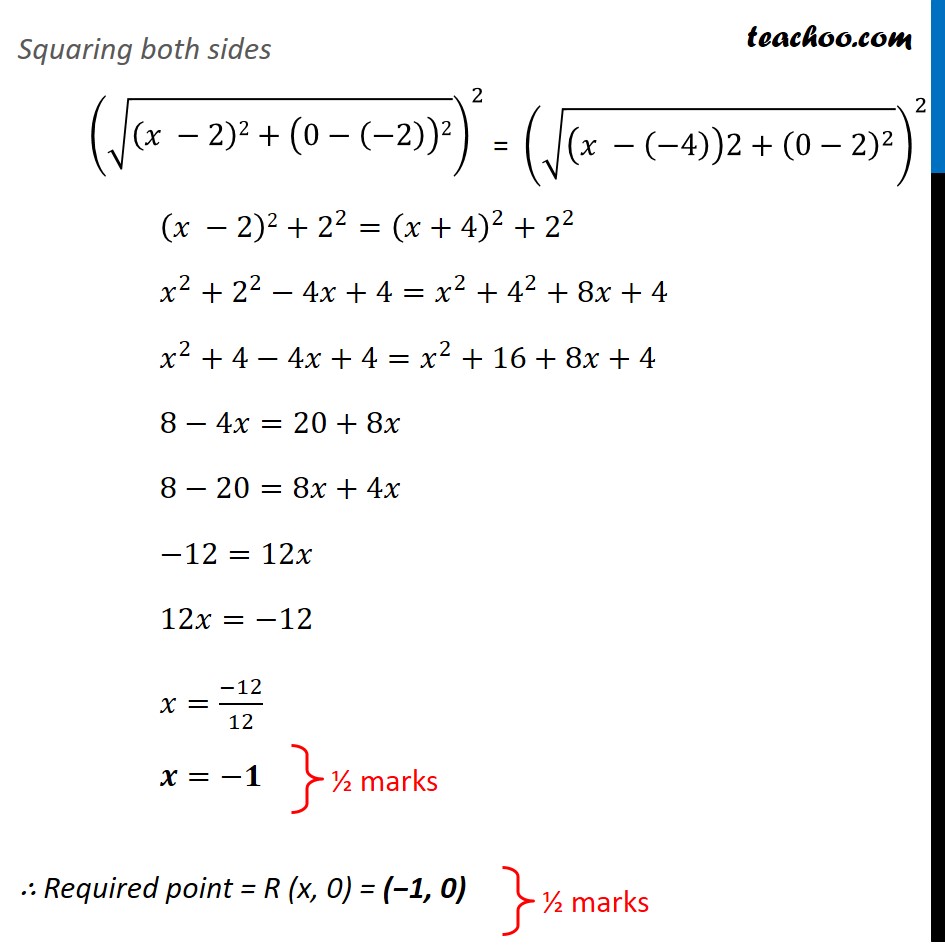
Question 22 (Choice - 1) Find the point on x-axis which is equidistant from the points (2, –2) and (–4, 2) Let the given points be P (2, −2) , Q (−4, 2) Since point equidistant from PQ is on x-axis ∴ It’s y-coordinate will be 0 Let required point be R (x, 0) Now, Point R is equidistant from P & Q Hence, PR = QR √((𝑥 −2)2+(0−(−2))2) = √((𝑥 −(−4))2+(0−2)2) Squaring both sides (√((𝑥 −2)2+(0−(−2))2))^2 = (√((𝑥 −(−4))2+(0−2)^2 ))^2 (𝑥 −2)2+2^2=(𝑥+4)^2+2^2 𝑥^2+2^2−4𝑥+4=𝑥^2+4^2+8𝑥+4 𝑥^2+4−4𝑥+4=𝑥^2+16+8𝑥+4 8−4𝑥=20+8𝑥 8−20=8𝑥+4𝑥 −12=12𝑥 12𝑥=−12 𝑥=(−12)/12 𝒙=−𝟏 ∴ Required point = R (x, 0) = (−1, 0)

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.