CBSE Class 12 Sample Paper for 2020 Boards
You are here
CBSE Class 12 Sample Paper for 2020 Boards
Last updated at Dec. 16, 2024 by Teachoo
Transcript
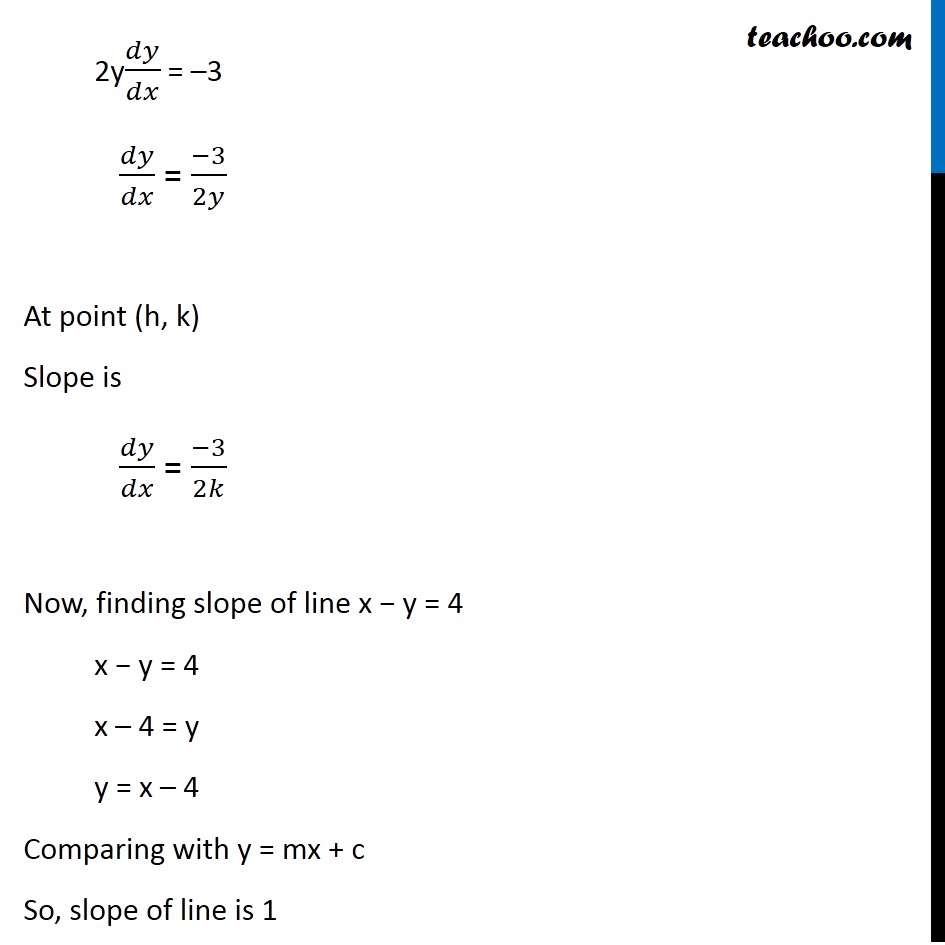
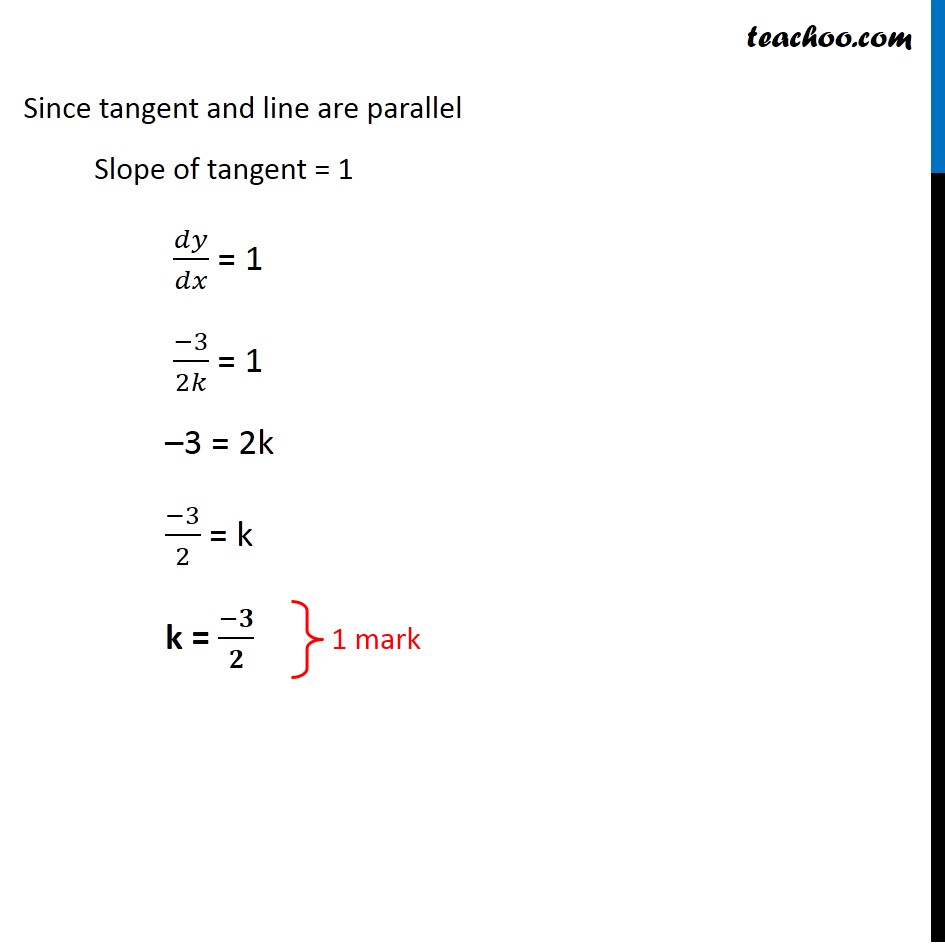
Question 14 (OR 1st Question) If tangent to the curve y2 + 3x − 7 = 0 at the point (ℎ, k) is parallel to line x − y = 4, then value of k is ______? We know that Slope of tangent is 𝑑𝑦/𝑑𝑥 Now, y2 + 3x − 7 = 0 Differentiating w.r.t.𝑥 2y𝑑𝑦/𝑑𝑥 + 3 – 0 = 0 2y𝑑𝑦/𝑑𝑥 + 3 = 0 2y𝑑𝑦/𝑑𝑥 = –3 𝑑𝑦/𝑑𝑥 = (−3)/2𝑦 At point (h, k) Slope is 𝑑𝑦/𝑑𝑥 = (−3)/2𝑘 Now, finding slope of line x − y = 4 x − y = 4 x – 4 = y y = x – 4 Comparing with y = mx + c So, slope of line is 1 Since tangent and line are parallel Slope of tangent = 1 𝑑𝑦/𝑑𝑥 = 1 (−3)/2𝑘 = 1 –3 = 2k (−3)/2 = k k = (−𝟑)/𝟐
