Example 11 - Chapter 2 Class 9 Polynomials
Last updated at May 29, 2018 by Teachoo
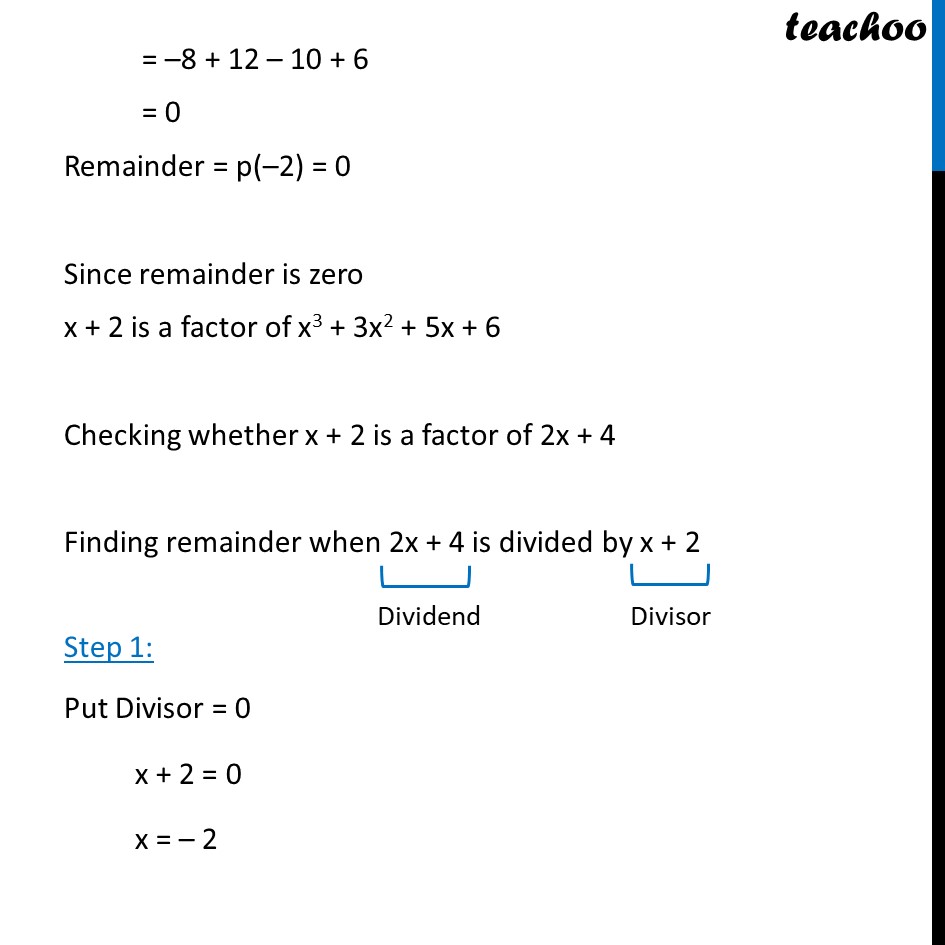
Transcript
Example 11 Examine whether x + 2 is a factor of x3 + 3x2 + 5x + 6 and of 2x + 4. First checking whether x + 2 is a factor of x3 + 3x2 + 5x + 6 Finding remainder when x3 + 3x2 + 5x + 6 is divided by x + 2 Step 1: Put Divisor = 0 x + 2 = 0 x = 2 Step 2: Let p(x) = x3 + 3x2 + 5x + 6 Putting x = 2 p( 2) = ( 2)3 + 3( 2)2 + 5( 2) + 6 = 8 + 12 10 + 6 = 0 Remainder = p( 2) = 0 Since remainder is zero x + 2 is a factor of x3 + 3x2 + 5x + 6 Checking whether x + 2 is a factor of 2x + 4 Finding remainder when 2x + 4 is divided by x + 2 Step 1: Put Divisor = 0 x + 2 = 0 x = 2 Step 2: Let p(x) = 2x + 4 Putting x = 2 p( 2) = 2( 2) + 4 = 4 + 4 = 0 Remainder = p( 2) = 0 Since remainder is zero x + 2 is a factor of 2x + 4
Examples
Example 1
Example 2
Example 3 Important
Example 4
Example 5
Example 6
Example 7 Important
Example 8
Example 9
Example 10
Example 11 You are here
Example 12 Important
Example 13
Example 14
Example 15 Important
Example 16
Example 17
Example 18 Important
Example 19
Example 20
Example 21 Important
Example 22
Example 23 Important
Example 24 Important
Example 25
About the Author
Davneet Singh
Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 9 years. He provides courses for Maths and Science at Teachoo.