NCERT Question 6 - Chapter 12 Class 10 - Electricity

Transcript

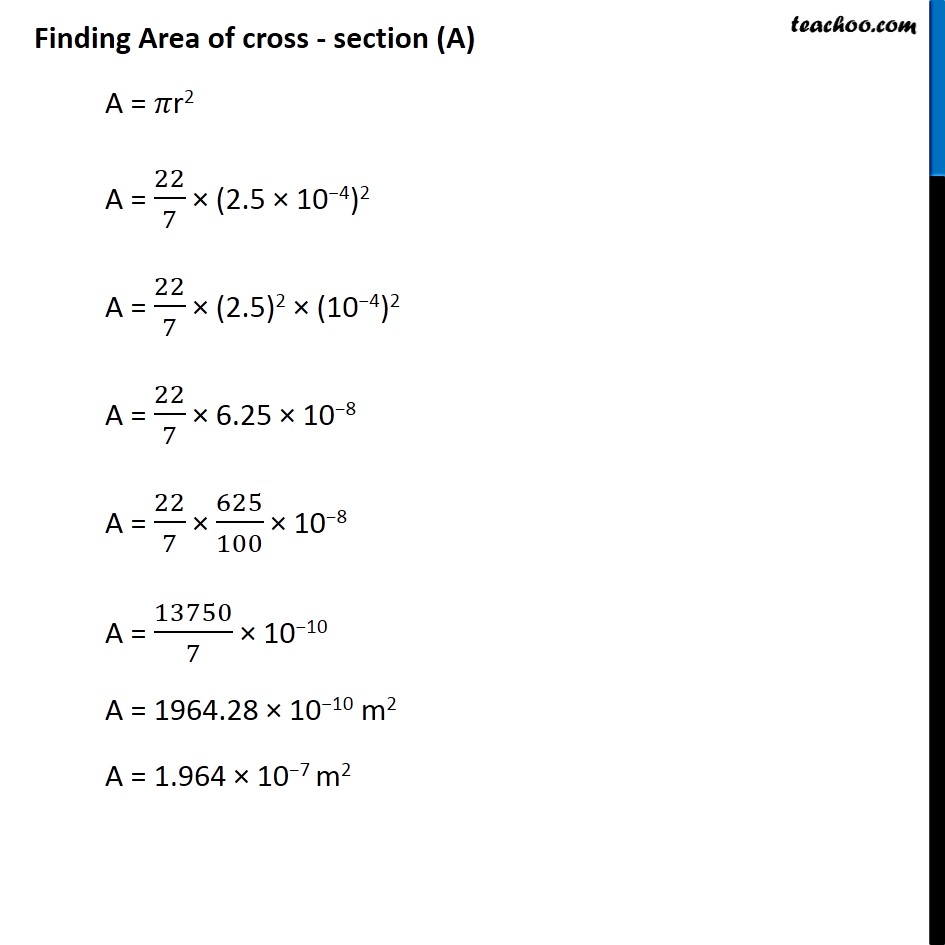
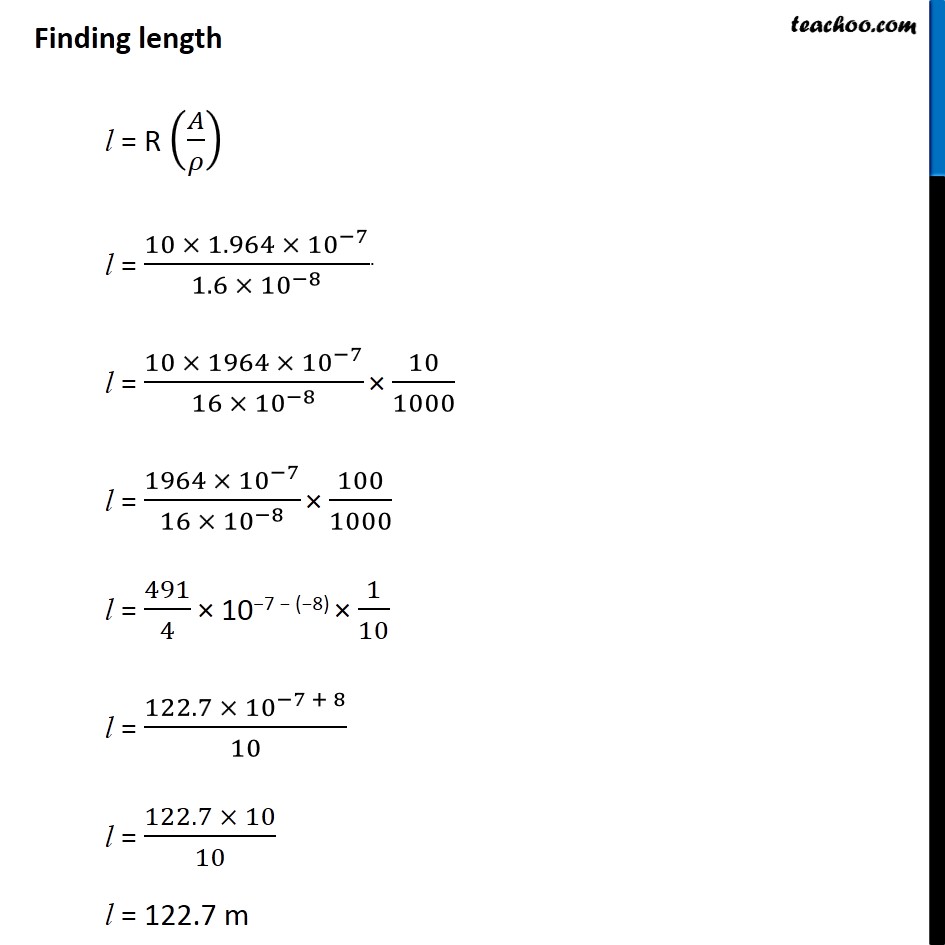
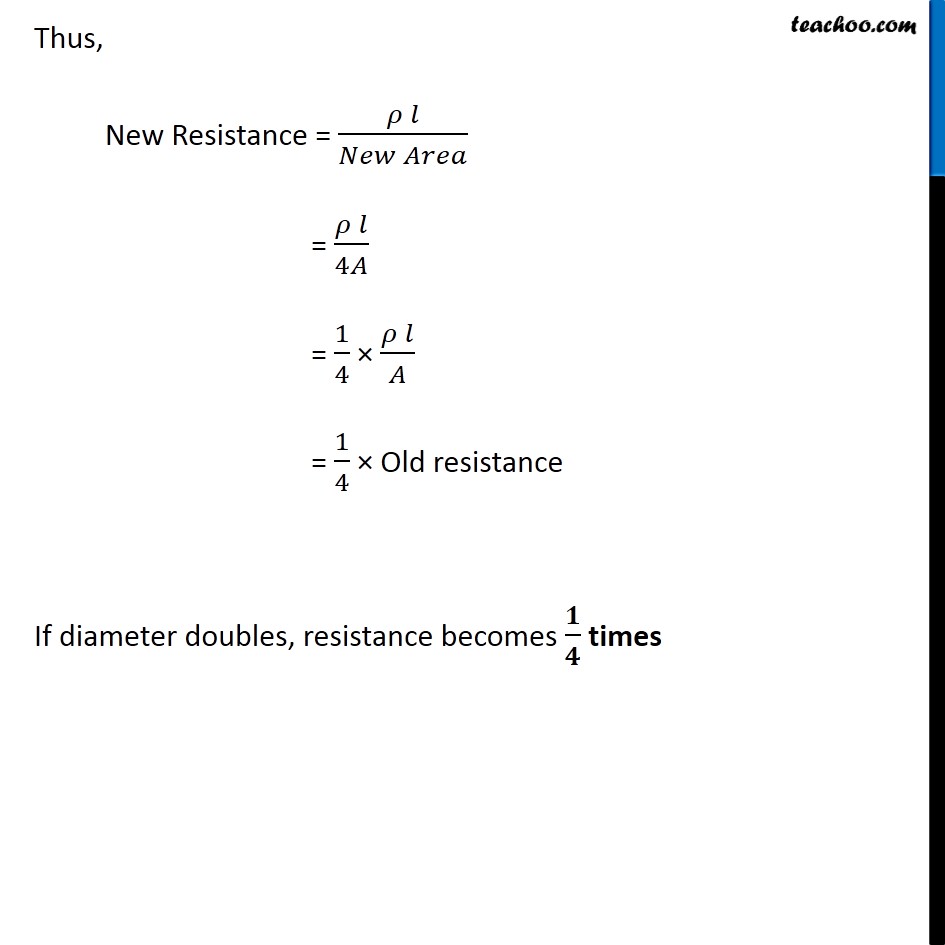
NCERT Question 6 A copper wire has diameter 0.5 mm and resistivity of 1.6 × 10–8 Ω m. What will be the length of this wire to make its resistance 10 Ω? How much does the resistance change if the diameter is doubled? Diameter = 0.5 mm = 0.5 × 10−3 m = 5 × 10−4 m Radius = r = 𝐷𝑖𝑎𝑚𝑒𝑡𝑒𝑟/2 = (5 × 〖10〗^(−4))/2 m = 2.5 × 10−4 m Resistivity = 𝜌 = 1.6 × 10−8 Ω m Resistance = 10 Ω We need to find length of the wire (l) We know that R = 𝜌 𝑙/𝐴 ∴ l = R (𝐴/𝜌) To find l, first we need to find A Finding Area of cross - section (A) A = 𝜋r2 A = 22/7 × (2.5 × 10−4)2 A = 22/7 × (2.5)2 × (10−4)2 A = 22/7 × 6.25 × 10−8 A = 22/7 × 625/100 × 10−8 A = 13750/7 × 10−10 A = 1964.28 × 10−10 m2 A = 1.964 × 10−7 m2 Finding length l = R (𝐴/𝜌) l = (10 × 1.964 × 〖10〗^(−7))/(1.6 × 〖10〗^(−8) ). l = (10 × 1964 × 〖10〗^(−7))/(16 × 〖10〗^(−8) ) × 10/1000 l = (1964 × 〖10〗^(−7))/(16 × 〖10〗^(−8) ) × 100/1000 l = 491/4 × 10−7 − (−8) × 1/10 l = (122.7 × 〖10〗^(−7 + 8))/10 l = (122.7 × 10)/10 l = 122.7 m When diameter is doubled Since New Diameter = 2 × Old Diameter New Radius = 2 × Old Radius And, New Area = 𝜋 (New Radius)2 = 𝜋 × (2r)2 = 4𝜋r2 = 4 × Old Area = 4 × A Thus, New Resistance = (𝜌 𝑙)/(𝑁𝑒𝑤 𝐴𝑟𝑒𝑎) = (𝜌 𝑙)/4𝐴 = 1/4 × (𝜌 𝑙)/𝐴 = 1/4 × Old resistance If diameter doubles, resistance becomes 𝟏/𝟒 times